Powers D.L. Boundary Value Problems and Partial Differential Equations

Подождите немного. Документ загружается.

268 Chapter 4 The Potential Equation

Poisson Equation

Many problems in engineering and physics require the solution of the Poisson

equation,

∇

u =−H in a region R.

Here are three examples of such problems.

(1) u is the deﬂection of a membrane that is fastened at its edges, so u =0on

the boundary of

R; H is proportional to the pressure difference across

the membrane. (See Section 5.1.)

(2) u is the steady-state temperature in a cross section of a long cylindrical

rodthatiscarryinganelectricalcurrent;H is proportional to the power

in resistance heating. (See Section 5.2.)

(3) u is the stress function on the cross section

R of a cylindrical bar or rod

in torsion (the shear stresses are proportional to the partial derivatives

of u); H is proportional to the rate of twist and to the shear modulus of

the material; u = 0 on the boundary of

If H is a constant, a polynomial of the form

P(x, y) = A +Bx +Cy +Dx

+Exy +Fy

is a solution of Poisson’s equation, provided that

2(D +F) =−H.

The other coefﬁcients are arbitrary and may be chosen for convenience in sat-

isfying boundary conditions.

Example 4.

Find the deﬂection u of a membrane that is modeled by this problem. The

constant is H = p/σ ,wherep is the pressure difference (below to above) and

σ is the surface tension in the membrane.

A polynomial can be chosen that satisﬁes the partial differential equation

and boundary conditions on facing sides. For instance,

v(x) =

Hx(a −x)

satisﬁes the Poisson equation and two boundary conditions,

v(0) = 0,v(a) =0.

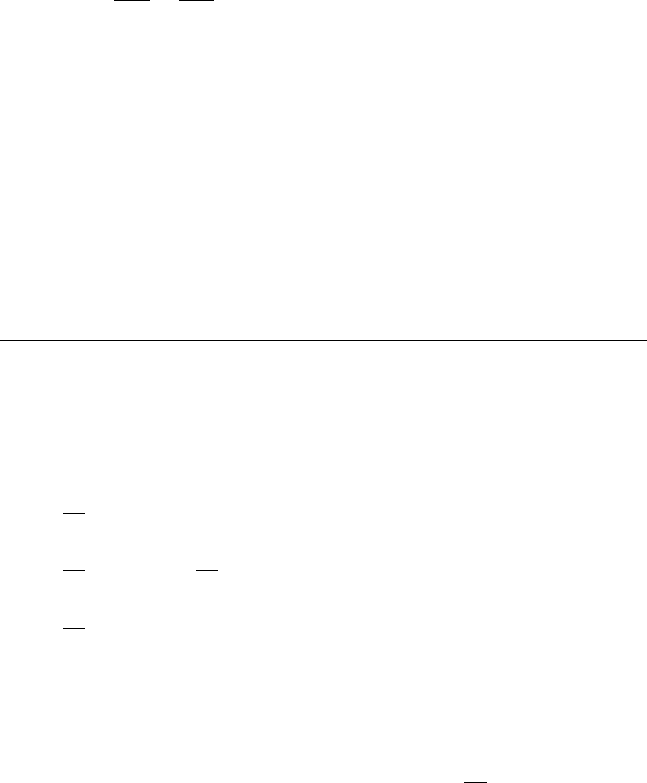
4.3 Further Examples for a Rectangle 269

Thus, we may set u(x, y) = v(x) + w(x, y) and determine that w is a solution

of the problem

∂

∂x

∂

∂y

=0, 0 < x < a, 0 < y < b,

w(0, y) = 0,w(a, y) = 0, 0 < y < b,

w(x, 0) =−v(x), w(x, b) =−v(x), 0 < x < a.

The CD has color graphics of the solution.



In general, if H is a polynomial in x and y, a solution can be found in the

form of a polynomial of total degree 2 higher than H.IfH is a more gen-

eral function, it may be expressed as a double Fourier series (see Chapter 5),

and the partial differential equation can be solved following the idea of Sec-

tion 1.11B.

EXERCISES

1. Solve the problem consisting of the potential equation on the rectangle

0 < x < a,0< y < b with the given boundary conditions. Two of the three

are very easy if a polynomial is subtracted from u.

∂u

∂x

(0, y) = 0; u = 1ontheremainderoftheboundary.

∂u

∂x

(0, y) = 0,

∂u

∂x

(a, y) = 0; u(x, 0) = 0, u(x, b) = 1.

∂u

∂x

(x, 0) = 0, u(x, b) = 0; u(0, y) = 1, u(a, y) =0.

2. Same task as Exercise 1.

a. u(x, b) = 100; the outward normal derivative is 0 on the rest of the

boundary.

b. u(x, b) =100, u(0, y) = 0, u(a, y) = 100,

∂u

∂y

(x, 0) = 0.

3. Finish the work for Example 1: Find the b

, form the series, and check that

all conditions are satisﬁed.

4. In Example 2, check that the given product solution for u

(x, y) satisﬁes

the conditions and determine the coefﬁcients a

and b

5. In Example 2, check that the given product solution for u

(x, y) satisﬁes

the conditions and determine the coefﬁcients A

and B

270 Chapter 4 The Potential Equation

Explain the difference between the cosine series in Example 1 and the co-

sine series for u

(x, y) in Example 2. What is the source of the difference?

7. Finish the work for Example 3. That is, ﬁnd w(x, y) as a series and check

that the boundary conditions are all satisﬁed.

8. Compare the amount of work involved in solving the problem of Exam-

ple 2 (including Exercises 4 and 5) with the work for Example 3 (including

Exercise 7).

9. Finish the work of Example 4: Find the solution, as a series, for w(x, y).

Form u(x, y) and use the ﬁrst term of the series for w(x, y) to obtain an

expression for the value of u(

). This would be the maximum deﬂection

of the membrane.

10. Find the condition on the coefﬁcients so that the following general

second-degree polynomial is a solution of the Poisson equation, ∇

p =

−H,whereH is constant:

p(x, y) = A + Bx +Cy +Dx

+Exy +Fy

11. Same task as Exercise 10, but H = K(x

+ y

) and p is this part of the

general fourth-degree polynomial

p(x, y) = Ax

+Bx

y +Cx

+Dxy

+Ey

∂

∂x

∂

∂y

=H, 0 < x < a, 0 < y < b,

u(0, y) = 0, u(a, y) = 0, 0 < x < a,

u(x, 0) = 0, u(x, b) = 0, 0 < y < b.

4.4 Potential in Unbounded Regions

The potential equation, as well as the heat and wave equations, can be solved

in unbounded regions. Consider the following problem, in which the region

involved is half a vertical strip, or a slot:

∂

∂x

∂

∂y

=0, 0 < x < a, 0 < y, (1)

u(x, 0) = f (x), 0 < x < a, (2)

u(0, y) = g

(y), 0 < y, (3)

u(a, y) = g

(y), 0 < y. (4)

As usual, we required that u(x, y) remain bounded as y →∞.

4.4 Potential in Unbounded Regions 271

In order to make the separation of variables work, we must break this

up into two problems. Following the model of Sections 4.2 and 4.3 we set

u(x, y) = u

(x, y) + u

(x, y) and require that the parts satisfy these two solv-

able problems:

∇

=0, ∇

=0, 0 < x < a, 0 < y,

(x, 0) = f (x), u

(x, 0) = 0, 0 < x < a,

(0, y) = 0, u

(0, y) =g

(y), 0 < y,

(a, y) = 0, u

(a, y) = g

(y), 0 < y.

We attack the problem for u

by assuming the product form and separating

variables:

(x, y) = X(x)Y(y),



(x)

X(x)

=−



(y)

Y(y )

=−λ

The sign of the constant −λ

is determined by the boundary conditions at

x = 0andx = a, which become homogeneous conditions on the factor X(x):

X(0) = 0, X(a) = 0. (5)

(We also can see that the condition to be satisﬁed along y = 0demandsfunc-

tions of x that permit a representation of an arbitrary function.)

The boundary conditions, Eq. (5), together with the differential equation



+λ

X = 0(6)

that comes from the separation of variables, constitute a familiar eigenvalue

problem, whose solution is

(x) = sin



nπx



,λ



nπ



, n =1, 2, 3,....

The equation for Y is



−λ

Y = 0, 0 < y.

In addition to satisfying this differential equation, Y must remain bounded as

y →∞. The solutions of the equation are e

λy

and e

−λy

. Of these, the ﬁrst is

unbounded, so

(y) = exp(−λ

y).

Finally, we can write the solution of the ﬁrst problem as

(x, y) =

∞



n=1

sin



nπx



exp



−nπy



. (7)

The constants a

aretobedeterminedfromtheconditionaty = 0.

272 Chapter 4 The Potential Equation

The solution of the second problem is somewhat different. Again we seek

solutions in the product form u

(x, y) = X(x)Y(y). The homogeneous bound-

ary condition at y = 0 and the boundedness condition become conditions on

Y(y ):

Y(0) = 0, Y(y) bounded as y →∞.

Then the potential equation becomes



(x)

X(x)



(y)

Y(y )

=0, (8)

and both ratios must be constant. If Y



/Y is positive, the auxiliary conditions

force Y to be identically 0. Thus, we take Y



/Y =−µ

,orY



+µ

Y = 0, and

ﬁnd that the solution that satisﬁes the auxiliary conditions is

Y(y ) =sin(µy),

for any µ>0. Then the general solution of the equation X



/X = µ

X(x) = A

sinh(µx)

sinh(µa)

sinh(µ(a − x))

sinh(µa)

We have chosen this special form on the basis of our experience in solving the

potential equation in the rectangle.

Since µ is a continuous parameter, we combine our product solutions by

means of an integral, ﬁnding

(x, y) =



∞



A(µ)

sinh(µx)

sinh(µa)

+B(µ)

sinh(µ(a − x))

sinh(µa)



sin(µy) dµ. (9)

The nonhomogeneous boundary conditions at x =0andx = a are satisﬁed if

(0, y) =



∞

B(µ) sin(µy) dµ = g

(y), 0 < y,

(a, y) =



∞

A(µ) sin(µy) dµ = g

(y), 0 < y.

Obviously these two equations are Fourier integral problems, so we know how

to determine the coefﬁcients A(µ) and B(µ). An example of this kind of prob-

lem is shown on the CD.

The potential equation can also be solved in a strip (0 < x < a, −∞ < y

< ∞), a quarter-plane (0 < x,0< y), or a half-plane (0 < x, −∞< y < ∞).

Along each boundary line, a boundary condition is imposed, and the solution

is required to remain bounded in remote portions of the region considered. In

general, a Fourier integral is employed in the solution, because the separation

constant is a continuous parameter, as in the second problem here.

4.4 Potential in Unbounded Regions 273

EXERCISES

1. Find a formula for the constants a

in Eq. (7).

2. Ve r i f y t h a t u

(x, y) in the form given in Eq. (7) satisﬁes the potential equa-

tion and the homogeneous boundary conditions.

3. Find formulas for A(µ) and B(µ) of Eq. (9).

4. Solve the potential equation in the slot, 0 < x < a,0< y, for each of these

sets of boundary conditions.

a. u(0, y) = 0, u(a, y) = 0, 0 < y; u(x, 0) =1, 0 < x < a;

b. u(0, y) = 0, u(a, y) = e

−y

,0< y; u(x, 0) = 0, 0 < x < a;

c. u(0, y) = f (y) =



1, 0 < y < b,

0, b < y,

u(a, y) = 0, 0 < y;

u(x, 0) = 0, 0 < x < a.

5. Solve the potential equation in the slot, 0 < x < a,0< y, for each of these

sets of boundary conditions.

∂u

∂x

(0, y) = 0, u(a, y) = 0, 0 < y; u(x, 0) = 1, 0 < x < a;

∂u

∂x

(0, y) = 0, u(a, y) = e

−y

,0< y;

u(x, 0) = 0, 0 < x < a;

c. u(0, y) = 0, u(a, y) = f (y) =



1, 0 < y < b,

0, b < y;

∂u

∂y

(x, 0) = 0, 0 < x < a.

6. Show that if the separation constant had been chosen as −µ

instead of

in solving for u

(leading to Y



− µ

Y = 0), then Y(y) ≡ 0isthe

only function that satisﬁes the differential equation, satisﬁes the condition

Y(0) = 0, and remains bounded as y →∞.

7. Solve the problem of potential in a slot under the boundary conditions

u(x, 0) = 1, u(0, y) = u(a, y) = e

−y

8. Show that the function v(x, y) given here satisﬁes the potential equation

and the boundary conditions on the “long” sides in Exercise 7, provided

274 Chapter 4 The Potential Equation

that cos(a/2) = 0:

v(x, y) =

cos



x −



cos





−y

What partial differential equation and boundary conditions are satisﬁed

by w(x, y) = u(x, y) −v(x, y) if u is the function of Exercise 7?

9. Solve the potential equation in the slot 0 < y < b,0< x for each of the

following sets of boundary conditions:

a. u(0, y) = 0, u(x, 0) =0, u(x, b) = f (x) =



1, 0 < x < a,

0, a < x;

b. u(0, y) = 0, u(x, 0) =e

−x

, u(x , b) =0.

10. Find product solutions of this potential problem in a strip:

∂

∂x

∂

∂y

=0, 0 < x < a, −∞ < y < ∞,

subject to the boundedness condition u(x, y) bounded as y →±∞.

11. Solve the potential problem consisting of the equation and boundedness

conditions from Exercise 10 and the boundary conditions

u(0, y) = 0, u(a, y) =e

−|y|

, −∞< y < ∞.

12. Show how to solve the potential problem of Exercise 10 together with the

boundary conditions

u(0, y) = g

(y), u(a, y) = g

(y), −∞ < y < ∞,

where g

and g

are suitable functions.

13. Find product solutions of this potential problem in the quarter-plane:

∂

∂x

∂

∂y

=0, 0 < x, 0 < y,

u(0, y) = 0, u(x, 0) = f (x).

Note that u(x, y) must remain bounded as x →∞and as y →∞.

14. Solve the potential equation in the quarter-plane, x > 0, y > 0, subject to

the boundary conditions

u(0, y) = e

−y

, y > 0; u(x, 0) = e

−x

, x > 0.

4.5 Potential in a Disk 275

15. Find product solutions of the potential equation in the half-plane y > 0:

∂

∂x

∂

∂y

=0, −∞< x < ∞, 0 < y < ∞,

u(x, 0) = f (x), −∞ < x < ∞.

What boundedness conditions must u(x, y) satisfy?

16. Convert your solution of Exercise 15 into the following formula (see Ex-

ercise 8 of Section 4.4 and Section 2.11):

u(x, y) =



∞

−∞

f (x



)

+(x −x



)



17. Use the formula in Exercise 16 to solve the potential problem in the upper

half-plane, with boundary condition

u(x, 0) = f (x) =



1, 0 < x,

0, x < 0.

18. Solve the problem stated in Exercise 15 if the boundary function is

f (x) =



1, |x | < a,

0, |x | > a.

19. Show that u(x, y) = x is the solution of the potential equation in a slot

under the boundary conditions f (x) = x, g

(y) = 0, g

(y) = a. Can this

solution be found by the method of this section?

4.5 Potential in a Disk

If we need to solve the potential equation in a circular disk x

+ y

< c

,itis

naturaltousepolarcoordinatesr, θ , in terms of which the disk is described

by 0 < r < c. We found in Section 4.1 that the potential equation in polar

coordinates is

∂

∂r



∂v

∂r



∂

∂θ

=0.

There are some special features of this coordinate system. First, it is clear that

some coefﬁcients of the Laplacian are negative powers of r. Thus, we must

enforce a boundedness condition at r = 0. Second, θ and θ +2π refer to the

same angle. Therefore, we must require that the function v(r,θ) be periodic

with period 2π in θ .

276 Chapter 4 The Potential Equation

The Dirichlet problem on a disk can now be stated as

∂

∂r



∂v

∂r



∂

∂θ

=0, 0 ≤r < c, (1)

v(c,θ)= f (θ ), (2)

v(r,θ +2π)=v(r,θ), 0 < r < c, (3)

v(r,θ) bounded as r →0+. (4)

By assuming v(r,θ)=R(r)Q(θ) we can separate variables. The potential equa-

tion becomes







Q +



=0.

Separation is effected by dividing through by RQ/r

r(rR



(r))



R(r)



(θ)

Q(θ)

=0.

As usual, both terms must be constant. We know that if Q



/Q is a posi-

tive constant, then Q will be exponential, not periodic. Therefore we choose



/Q =−λ

and obtain this singular eigenvalue problem:



+λ

Q = 0, (5)

Q(θ +2π) = Q(θ). (6)

The accompanying equation for R(r) is







−λ

R =0, (7)

R(r) bounded as r → 0+. (8)

The general solution of Eq. (5) (if λ>0) is

Q(θ) = A cos(λθ) +B sin(λθ).

This function is periodic for all λ, but the period is 2π only if λ is an inte-

ger. Thus we have λ

= n, n = 1, 2,....Inaddition,ifλ = 0, we have a peri-

odic solution that is any constant. Thus, the solution of the singular eigenvalue

problem of Eqs. (5) and (6) is

=0, Q

(θ) = 1,

=n, Q

(θ) = A cos(nθ)+B sin(nθ), n = 1, 2, 3,....

The novelty here is that we have two eigenfunctions for each eigenvalue n =

1, 2,....

4.5 Potential in a Disk 277

Knowing that λ

,wecaneasilyﬁndR(r). The equation for R becomes



+rR



−n

R =0, 0 < r < c ,

when the indicated differentiations are carried out. This is a Cauchy–Euler

equation, whose solutions are known to have the form R(r) = r

,whereα

is constant. Substituting R = r

, R



= αr

α−1

,andR



= α(α − 1)r

α−2

into it

leaves



α(α −1) +α −n



=0, 0 < r < c .

Because r

is not zero, the constant factor in parentheses must be zero — that

is, α =±n. The general solution of the differential equation is any combina-

tion of r

and r

−n

.Thelatter,however,isunboundedasr approaches zero,

so we discard that solution, retaining R

(r) = r

.Inthespecialcasen = 0, the

two solutions are the constant function 1 and ln(r). The logarithm is discarded

because of its behavior at r =0.

Now we reassemble our solution. The functions

·1 =1, r

cos(nθ), r

sin(nθ) (9)

are all solutions of the potential equation, so a general linear combination of

these solutions will also be a solution. Thus v(r,θ)may have the form

v(r,θ)= a

∞



n=1

cos(nθ)+

∞



n=1

sin(nθ). (10)

At the true boundary r = c, the boundary condition reads

v(c,θ)=a

∞



n=1



cos(nθ)+b

sin(nθ)



=f (θ), −π<θ≤ π.

This is a Fourier series problem, as in Section 1.1, solved by choosing

2π



−π

f (θ) dθ,

πc



−π

f (θ) cos(nθ)dθ, b

πc



−π

f (θ) sin(nθ)dθ. (11)

Example.

Consider the problem consisting of Eqs. (1)–(4) with

v(c,θ)=f (θ ) =



0, −π<θ<−π/2,

1, −π/2 <θ<π/2,

0,π/2 <θ<π.