Pecharsky V.K., Zavalij P.Y. Fundamentals of Powder Diffraction and Structural Characterization of Materials

Подождите немного. Документ загружается.

Fundamentals

diffraction

Ewald's

sphere

....

Reciprocal

lattice

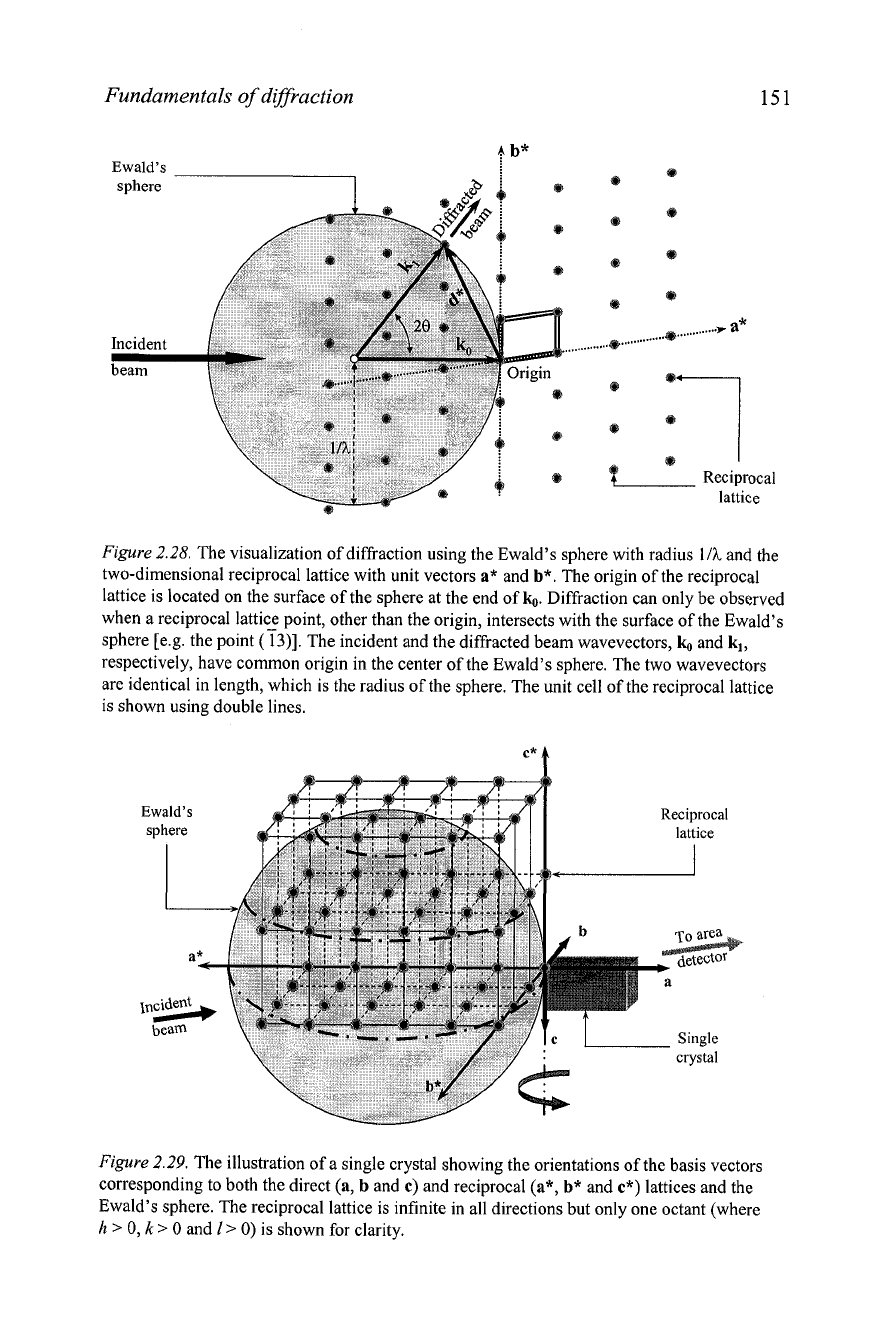
Figure

2.28.

The visualization of diffraction using the Ewald's sphere with radius

111

and the

two-dimensional reciprocal lattice with unit vectors

and

b*.

The origin of the reciprocal

lattice is located on the surface of the sphere at the end of

Diffraction can only be observed

when a reciprocal lattice point, other than the origin, intersects with the surface of the Ewald's

sphere [e.g. the point

(i3)].

The incident and the diffracted beam wavevectors,

and

kl,

respectively, have common origin in the center of the Ewald's sphere. The two wavevectors

are identical in length, which is the radius of the sphere. The unit cell of the reciprocal lattice

is shown using double lines.

Reciprocal

Figure

2.29.

The illustration of a single crystal showing the orientations of the basis vectors

corresponding to both the direct

(a,

and

and reciprocal

(a*,

and

c*)

lattices and the

Ewald's sphere. The reciprocal lattice is infinite in all directions but only one octant (where

and

is shown for clarity.

152

Chapter

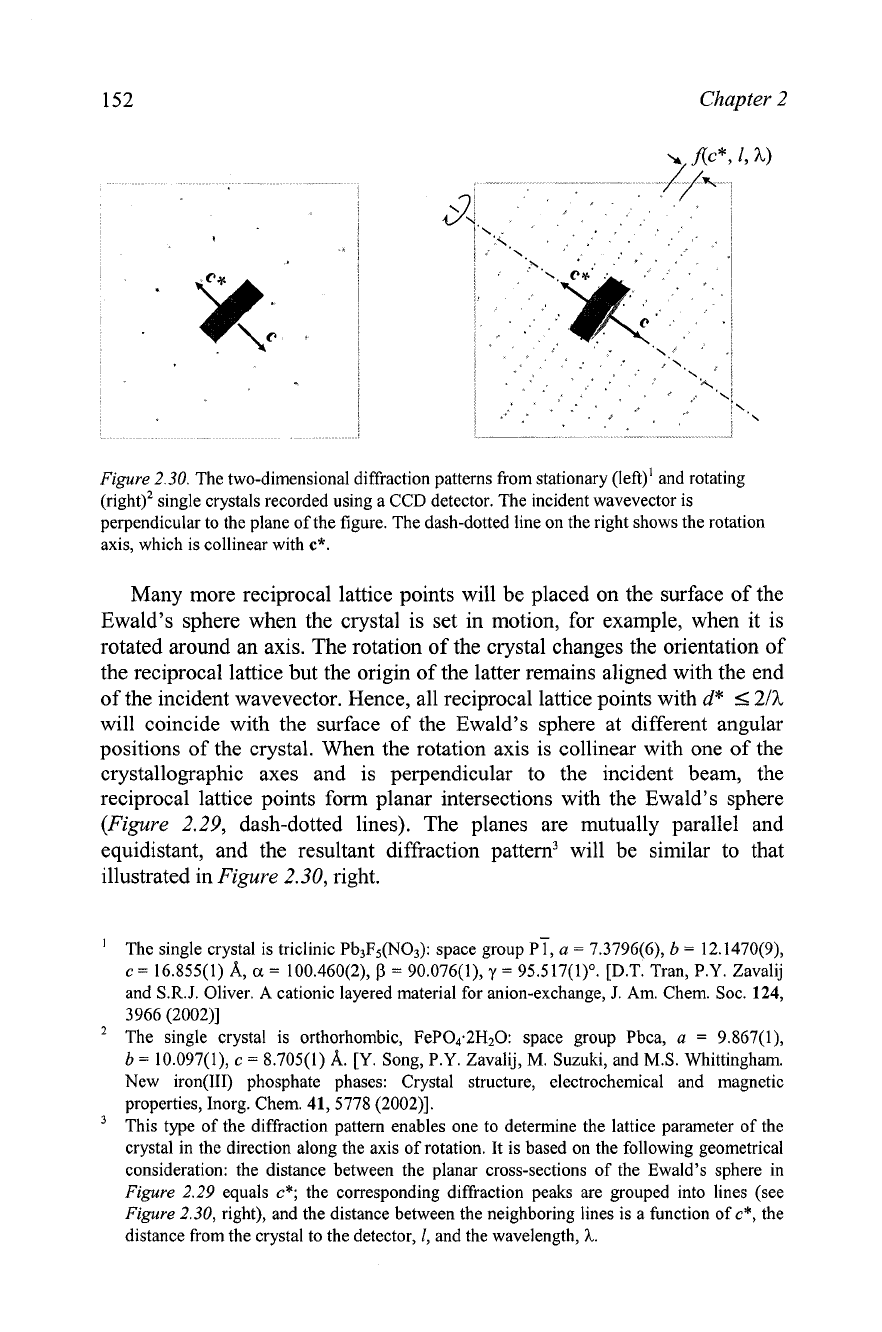
Figure

2.30.

The two-dimensional diffraction patterns from stationary (left)' and rotating

(right)' single crystals recorded using a CCD detector. The incident wavevector is

perpendicular to the plane of the figure. The dash-dotted line on the right shows the rotation

axis, which is collinear with

c*.

Many more reciprocal lattice points will be placed on the surface of the

Ewald's sphere when the crystal is set in motion, for example, when it is

rotated around an axis. The rotation of the crystal changes the orientation of

the reciprocal lattice but the origin of the latter remains aligned with the end

of the incident wavevector. Hence, all reciprocal lattice points with

I2/h

will coincide with the surface of the Ewald's sphere at different angular

positions of the crystal. When the rotation axis is collinear with one of the

crystallographic axes and is perpendicular to the incident beam, the

reciprocal lattice points form planar intersections with the Ewald's sphere

(Figure

2.29, dash-dotted lines). The planes are mutually parallel and

equidistant, and the resultant diffraction pattern3 will be similar to that

illustrated in

Figure

2.30,

right.

The single crystal is triclinic Pb3F5(N03): space group pi,

7.3796(6),

12.1470(9),

16.855(1)

100.460(2),

90.076(1),

95.517(1)". [D.T. Tran, P.Y. Zavalij

and S.R.J. Oliver. A cationic layered material for anion-exchange,

Am. Chem. Soc. 124,

3966 (2002)l

The single crystal is orthorhombic, FePO4.2H2O: space group Pbca,

9.867(1),

10.097(1),

8.705(1)

[Y. Song, P.Y. Zavalij,

Suzuki, and MS. Whittingham.

New iron(II1) phosphate phases: Crystal structure, electrochemical and magnetic

properties, Inorg. Chem. 41,5778 (2002)l.

This type of the diffraction pattern enables one to determine the lattice parameter of the

crystal in the direction along the axis of rotation. It is based on the following geometrical

consideration: the distance between the planar cross-sections of the Ewald's sphere in

Figure

2.29

equals

c*;

the corresponding diffraction peaks are grouped into lines (see

Figure

2.30,

right), and the distance between the neighboring lines is a function of

c*,

the

distance from the crystal to the detector,

and the wavelength,

Fundamentals of diffraction

153

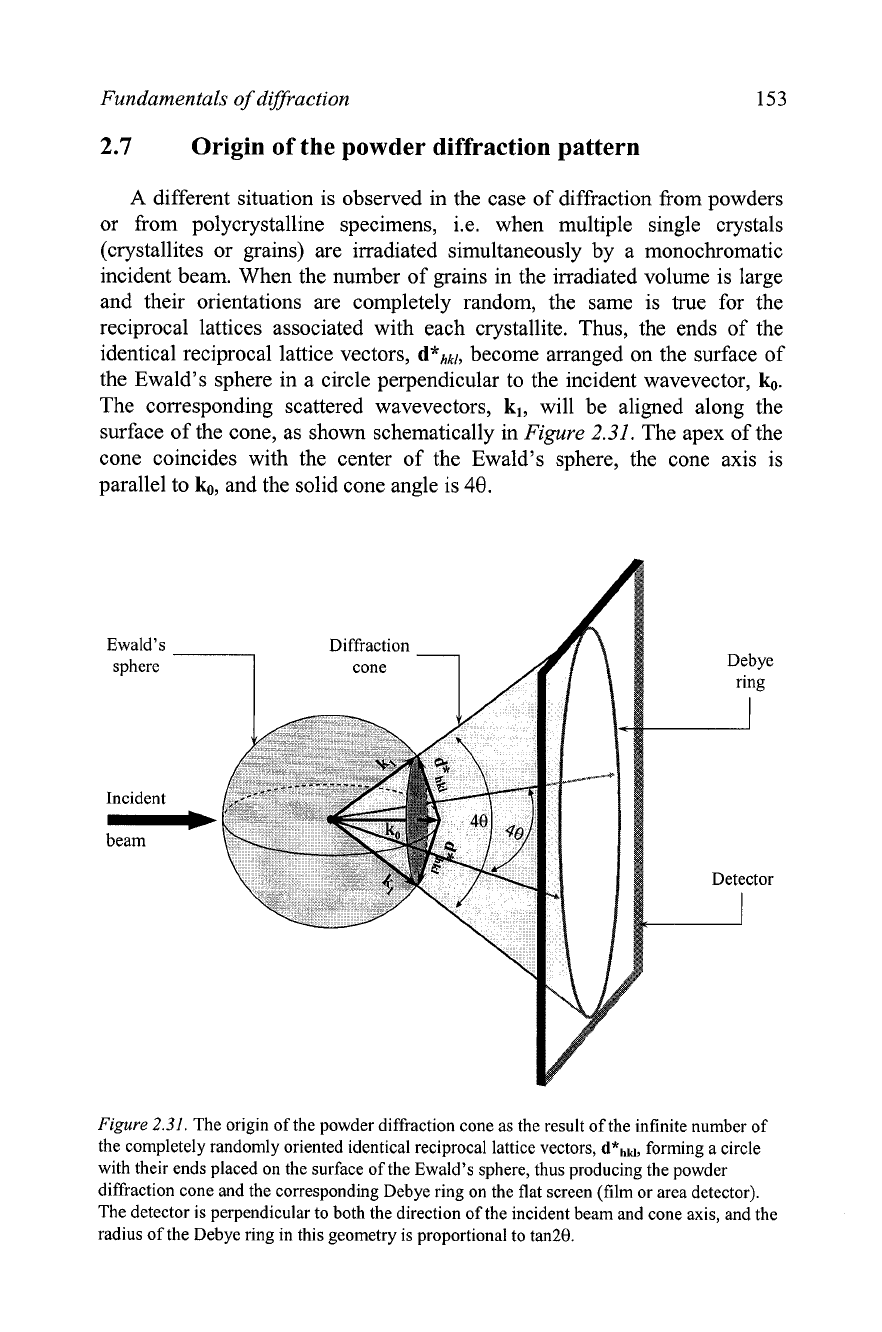
2.7 Origin of the powder diffraction pattern

different situation is observed in the case of diffraction from powders

or from polycrystalline specimens, i.e. when multiple single crystals

(crystallites or grains) are irradiated simultaneously by a monochromatic

incident beam. When the number of grains in the irradiated volume is large

and their orientations are completely random, the same is

.true for the

reciprocal lattices associated with each crystallite. Thus, the ends of the

identical reciprocal lattice vectors,

d*hkl,

become arranged on the surface of

the Ewald's sphere in a circle perpendicular to the incident wavevector,

k,,.

The corresponding scattered wavevectors,

kl,

will be aligned along the

surface of the cone, as shown schematically in

Figure

2.31.

The apex of the

cone coincides with the center of the Ewald's sphere, the cone axis is

parallel to

k,,,

and the solid cone angle is

48.

Ewald's

sphere

Incident

beam

Figure

2.31.

The origin of the powder diffraction cone as the result of the infinite number of

the completely randomly oriented identical reciprocal lattice vectors,

d*hkl,

forming a circle

with their ends placed on the surface of the Ewald's sphere, thus producing the powder

diffraction cone and the corresponding

Debye ring on the flat screen (film or area detector).

The detector is perpendicular to both the direction of the incident beam and cone axis, and the

radius of the Debye ring in this geometry is proportional to tan28.

154

Chapter

Assuming that the number of crystallites approaches infinity (the

randomness of their orientations has been postulated in the previous

paragraph), the density of the scattered wavevectors,

k,,

becomes constant

on the surface of the cone. The diffracted intensity will therefore, be constant

around the circumference of the cone base or, when measured by a planar

area detector as shown in

Figure

2.31, around the ring, which the cone base

forms with the plane of the detector. Similar rings but with different

intensities and diameters will be formed by other independent reciprocal

lattice vectors, and these are commonly known as the

Debye rings.'

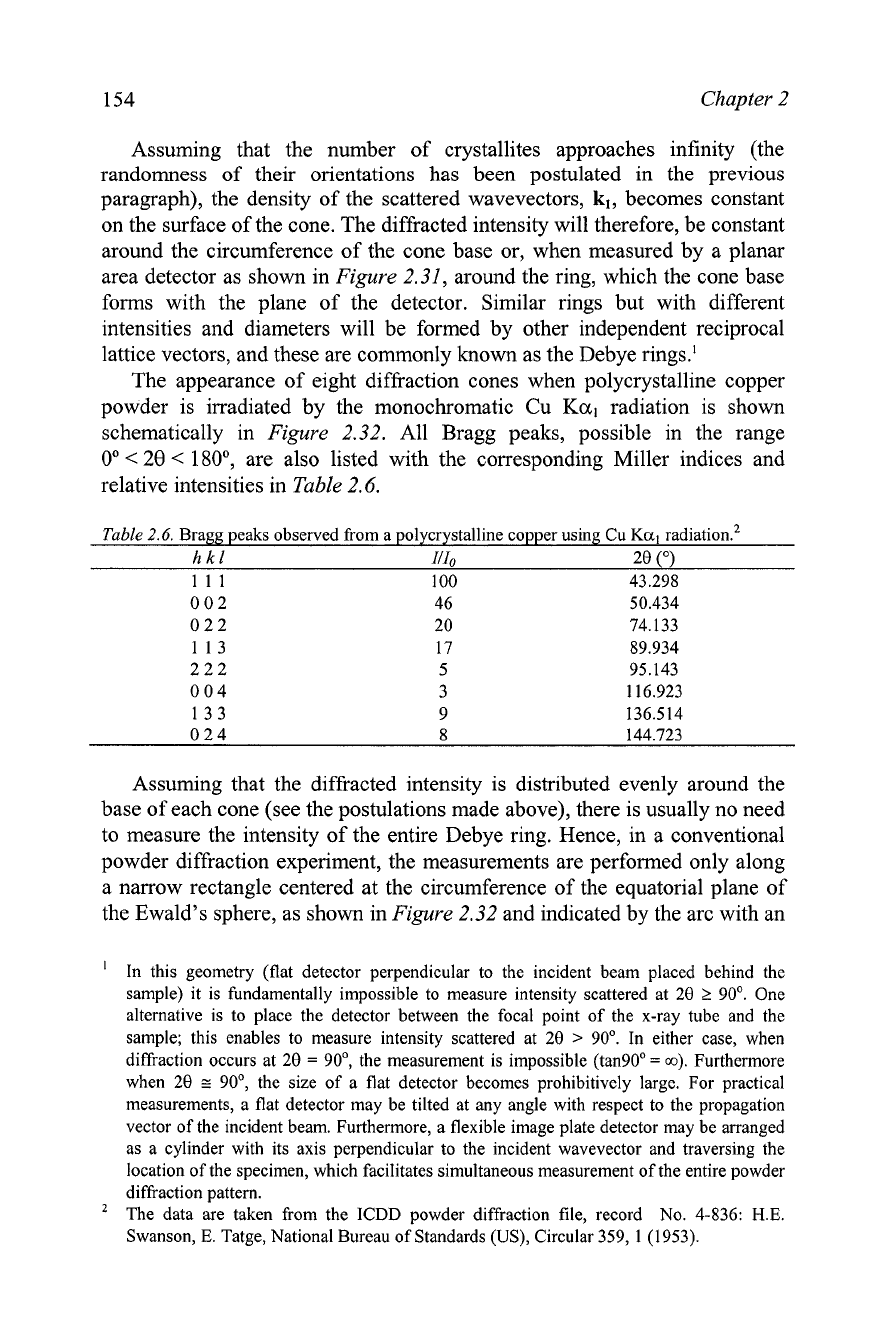
The appearance of eight diffraction cones when polycrystalline copper

powder is irradiated by the monochromatic Cu

Ka,

radiation is shown

schematically in

Figure

2.32. All Bragg peaks, possible in the range

180•‹, are also listed with the corresponding Miller indices and

relative intensities in

Table

2.6.

Table

2.6.

Bragg peaks observed from a polycrystalline copper using Cu

Kal

radiatiom2

hkl

IIIo

(0)

111 100 43.298

002 46 50.434

0 2

20 74.133

113 17 89.934

2 2 2 5 95.143

004 3 116.923

133 9 136.514

024

144.723

Assuming that the diffracted intensity is distributed evenly around the

base of each cone (see the postulations made above), there is usually no need

to measure the intensity of the entire

Debye ring. Hence, in a conventional

powder diffraction experiment, the measurements are performed only along

a narrow rectangle centered at the circumference of the equatorial plane of

the Ewald's sphere, as shown in

Figure

2.32 and indicated by the arc with an

In this geometry (flat detector perpendicular to the incident beam placed behind the

sample) it is fundamentally impossible to measure intensity scattered at

90'.

One

alternative is to place the detector between the focal point of the x-ray tube and the

sample; this enables to measure intensity scattered at

90'.

In either case, when

diffraction occurs at

90•‹,

the measurement is impossible

(tan90•‹

m).

Furthermore

when

90•‹,

the size of a flat detector becomes prohibitively large. For practical

measurements, a flat detector may be tilted at any angle with respect to the propagation

vector of the incident beam. Furthermore, a flexible image plate detector may be arranged

as a cylinder with its axis perpendicular to the incident wavevector and traversing the

location of the specimen, which facilitates simultaneous measurement of the entire powder

diffraction pattern.

The data are taken from the ICDD powder diffraction file, record No.

4-836:

H.E.

Swanson,

Tatge, National Bureau of Standards

(US),

Circular

359, 1 (1953).

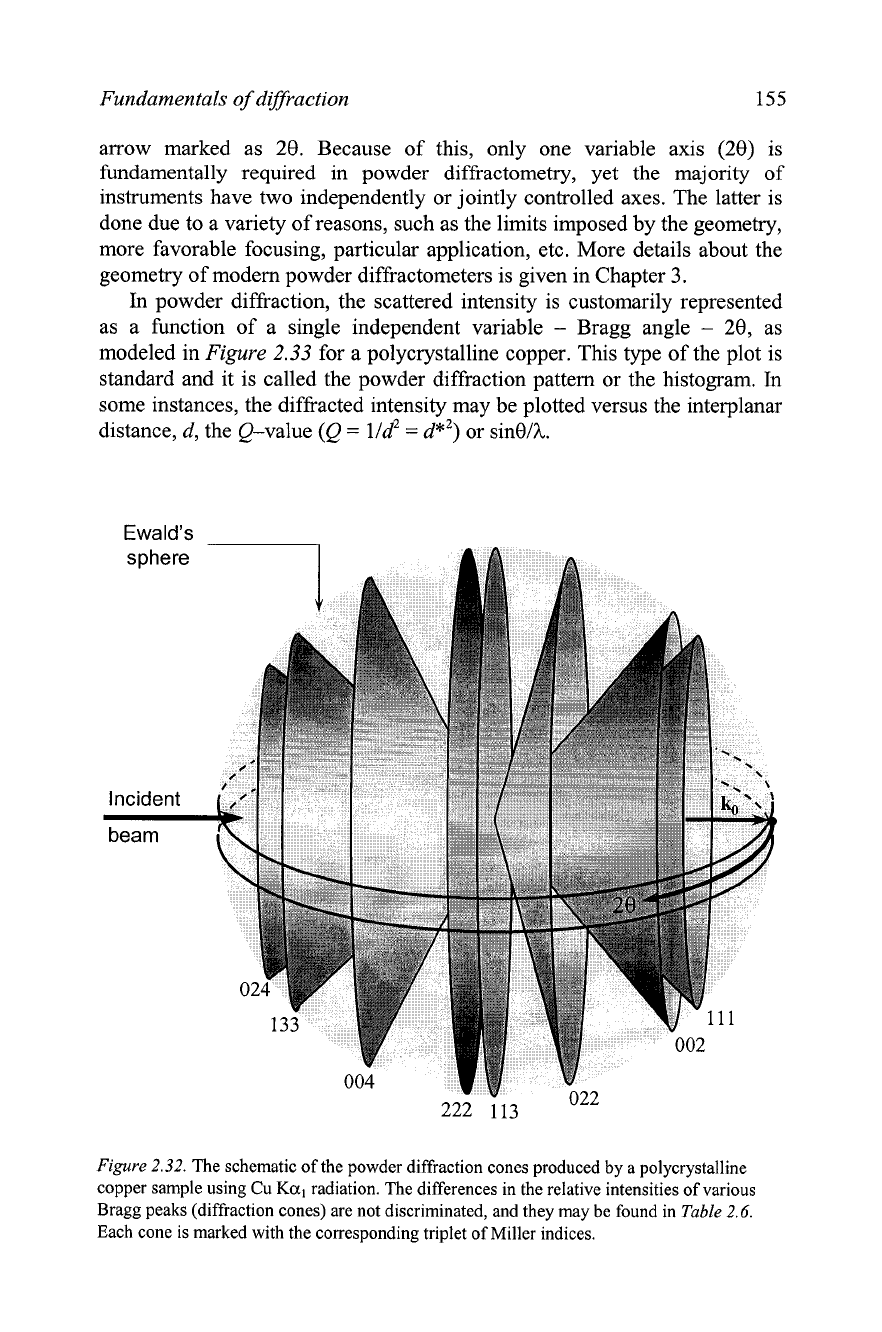
Fundamentals of diffraction 155

arrow marked as 20. Because of this, only one variable axis (20) is

fundamentally required in powder diffractometry, yet the majority of

instruments have two independently or jointly controlled axes. The latter is

done due to a variety of reasons, such as the limits imposed by the geometry,

more favorable focusing, particular application, etc. More details about the

geometry of modem powder diffractometers is given in Chapter

powder diffraction, the scattered intensity is customarily represented

as a function of a single independent variable

Bragg angle

20, as

modeled in Figure

2.33

for a polycrystalline copper. This type of the plot is

standard and it is called the powder diffraction pattern or the histogram.

some instances, the diffracted intensity may be plotted versus the interplanar

distance, d, the Q-value (Q

lld2

d*2) or sinelk.

Ewald's

sphere

Incident

beam

Figure

2.32.

The schematic of the powder diffraction cones produced by a polycrystalline

copper sample using Cu

Kal

radiation. The differences in the relative intensities of various

Bragg peaks (diffraction cones) are not discriminated, and they may

found in

Table

2.6.

Each cone is marked with the corresponding triplet of Miller indices.

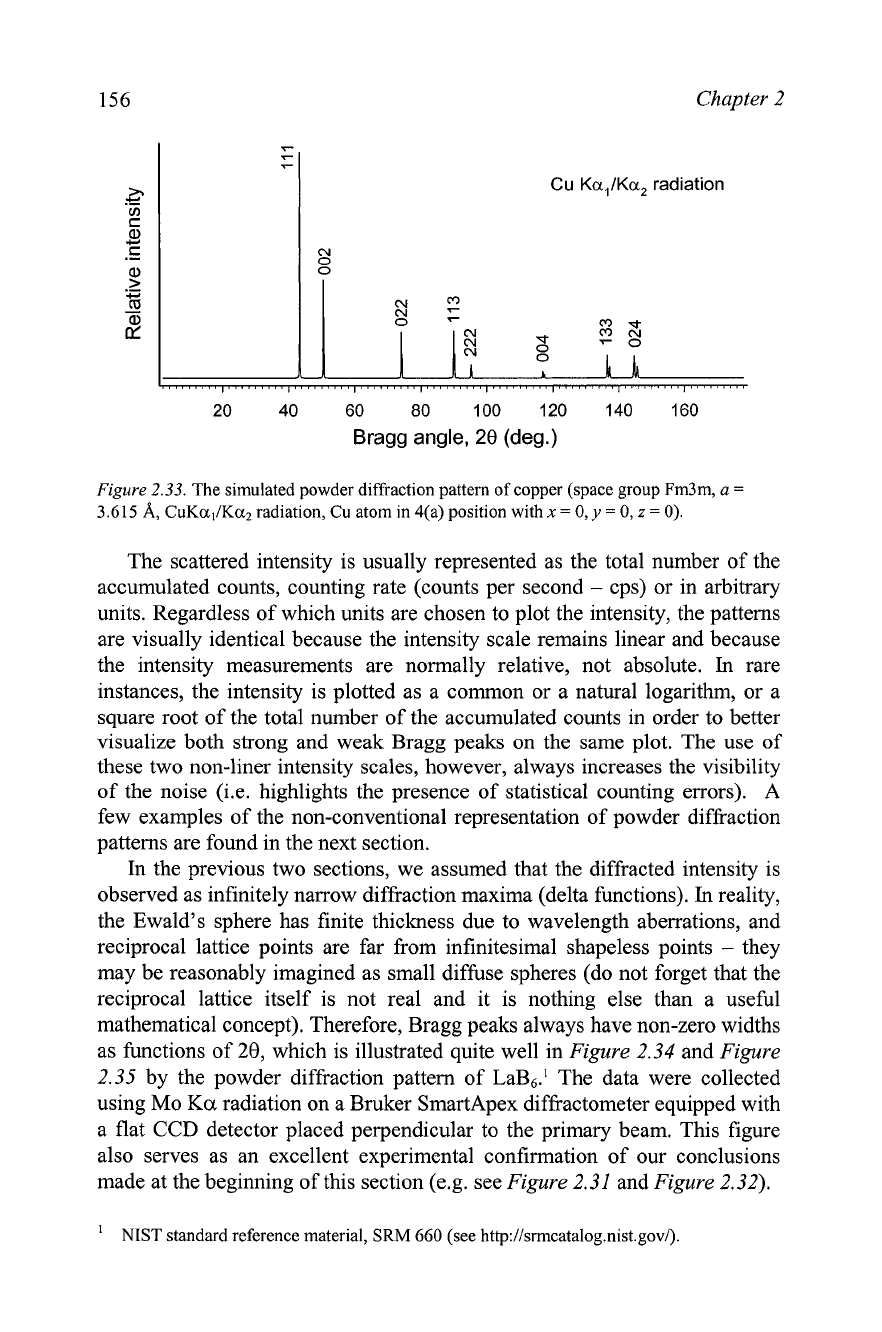
Chapter

Ka,lKa,

radiation

20 40 60 80 100 120 140 160

Bragg angle,

(deg.)

Figure

2.33.

The simulated powder diffraction pattern of copper (space group Fm3m,

3.615

CuKul/Ku2 radiation, Cu atom in 4(a) position with

0).

The scattered intensity is usually represented as the total number of the

accumulated counts, counting rate (counts per second

cps) or in arbitrary

units. Regardless of which units are chosen to plot the intensity, the patterns

are visually identical because the intensity scale remains linear and because

the intensity measurements are normally relative, not absolute.

rare

instances, the intensity is plotted as a common or a natural logarithm, or a

square root of the total number of the accumulated counts in order to better

visualize both strong and weak Bragg peaks on the same plot. The use

these two non-liner intensity scales, however, always increases the visibility

the noise (i.e. highlights the presence of statistical counting errors).

few examples of the non-conventional representation of powder diffraction

patterns are found in the next section.

the previous two sections, we assumed that the diffracted intensity is

observed as infinitely narrow diffraction maxima (delta functions).

reality,

the Ewald's sphere has finite thickness due to wavelength aberrations, and

reciprocal lattice points are far from infinitesimal shapeless points

they

may be reasonably imagined as small diffuse spheres (do not forget that the

reciprocal lattice itself is not real and it is nothing else than a useful

mathematical concept). Therefore, Bragg peaks always have non-zero widths

as functions of

28,

which is illustrated quite well in

Figure

2.34 and

Figure

2.35 by the powder diffraction pattern of La&.' The data were collected

using Mo

radiation on a Bruker SmartApex diffractometer equipped with

a flat

CCD

detector placed perpendicular to the primary beam. This figure

also serves as an excellent experimental confirmation of our conclusions

made at the beginning of this section (e.g. see

Figure

2.31 and

Figure

2.32).

NIST standard reference material, SRM

660

(see

http://srmcatalog.nist.gov/).

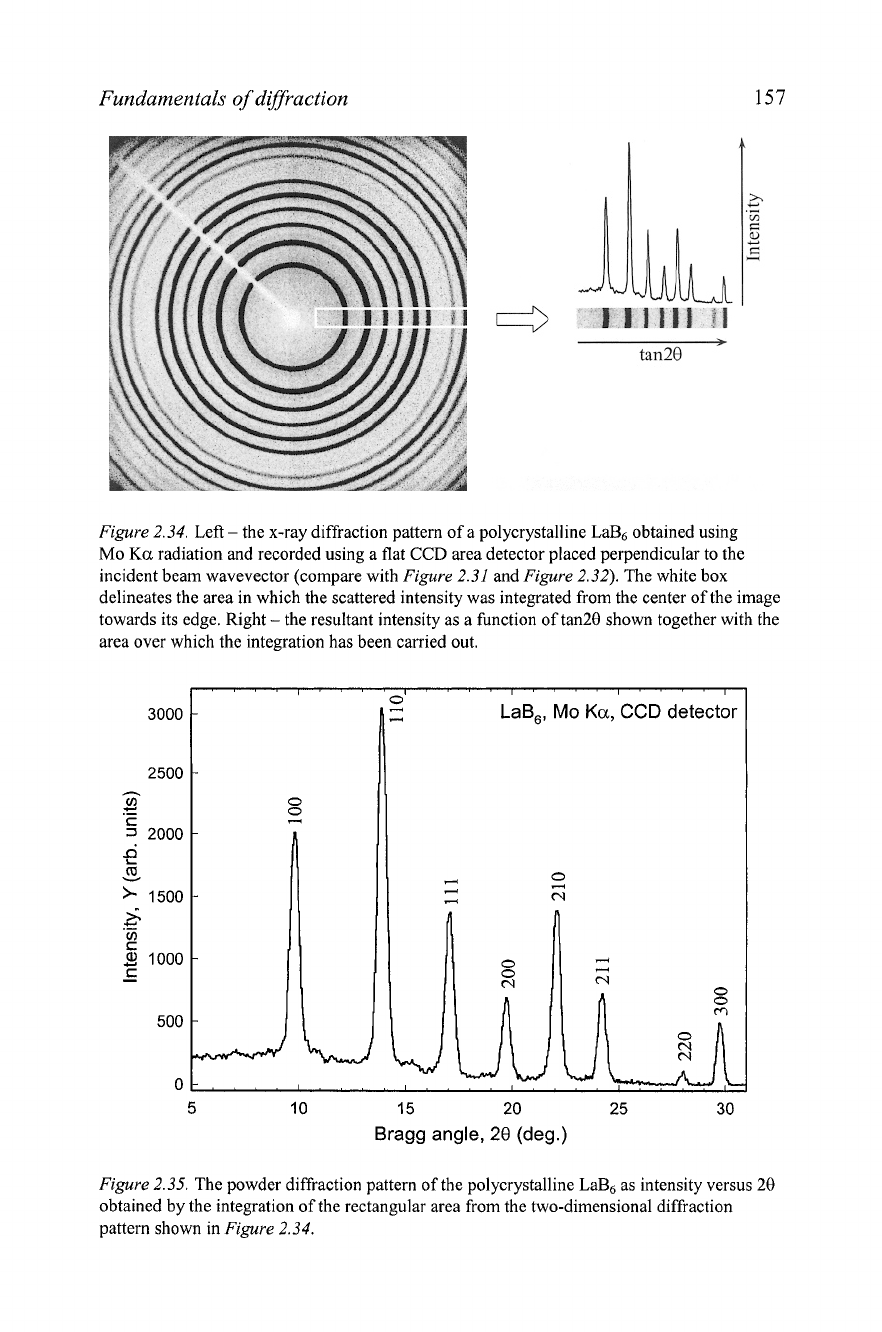
Fundamentals of diffraction

Figure

2.34.

Left

the x-ray diffraction pattern of a polycrystalline La& obtained using

radiation and recorded using a flat

CCD

area detector placed perpendicular to the

incident beam wavevector (compare with

Figure

2.31

and

Figure

2.32).

The white box

delineates the area in which the scattered intensity was integrated from the center of the image

towards its edge. Right

the resultant intensity as a function of tan28 shown together with the

area over which the integration has been carried out.

I'"'I'"'I

LaB,,

Ka,

CCD

detector

Bragg angle,

(deg.)

Figure

2.35.

The powder diffraction pattern of the polycrystalline La& as intensity versus 28

obtained by the integration of the rectangular area from the two-dimensional diffraction

pattern shown in

Figure

2.34.

158 Chapter 2

As shown in Figure 2.34, when diffraction cones, produced by the LaB6

powder, intersect with the flat detector placed perpendicularly to the incident

wavevector, they create a set of concentric

Debye rings. As in a typical

powder diffi-actometer, only a narrow band has been scanned and the result

of the integration is also shown in Figure 2.34 as the scattered intensity

versus tan29 (note, that the radial coordinate of the detector is tan29 and not

29). The resultant diffi-action pattern is shown in the standard format as

relative intensity versus

in Figure 2.35, where each Bragg peak is labeled

with the corresponding Miller indices.

It is worth noting that the diffractometer used in this experiment is a

single crystal diffractometer, which was not designed to take full advantage

of focusing of the scattered beam. As a result, the Bragg peaks shown in

Figure 2.35 are quite broad and the

Kalla2 doublet is unresolved even when

29 approaches

30'. As we will see in Chapter 3 (e.g. see Figure 3.37) a

much better resolution is possible in high resolution powder diffi-actometers,

where the doublet becomes resolved at much lower Bragg angles.

2.7.1

Representation of powder diffraction patterns

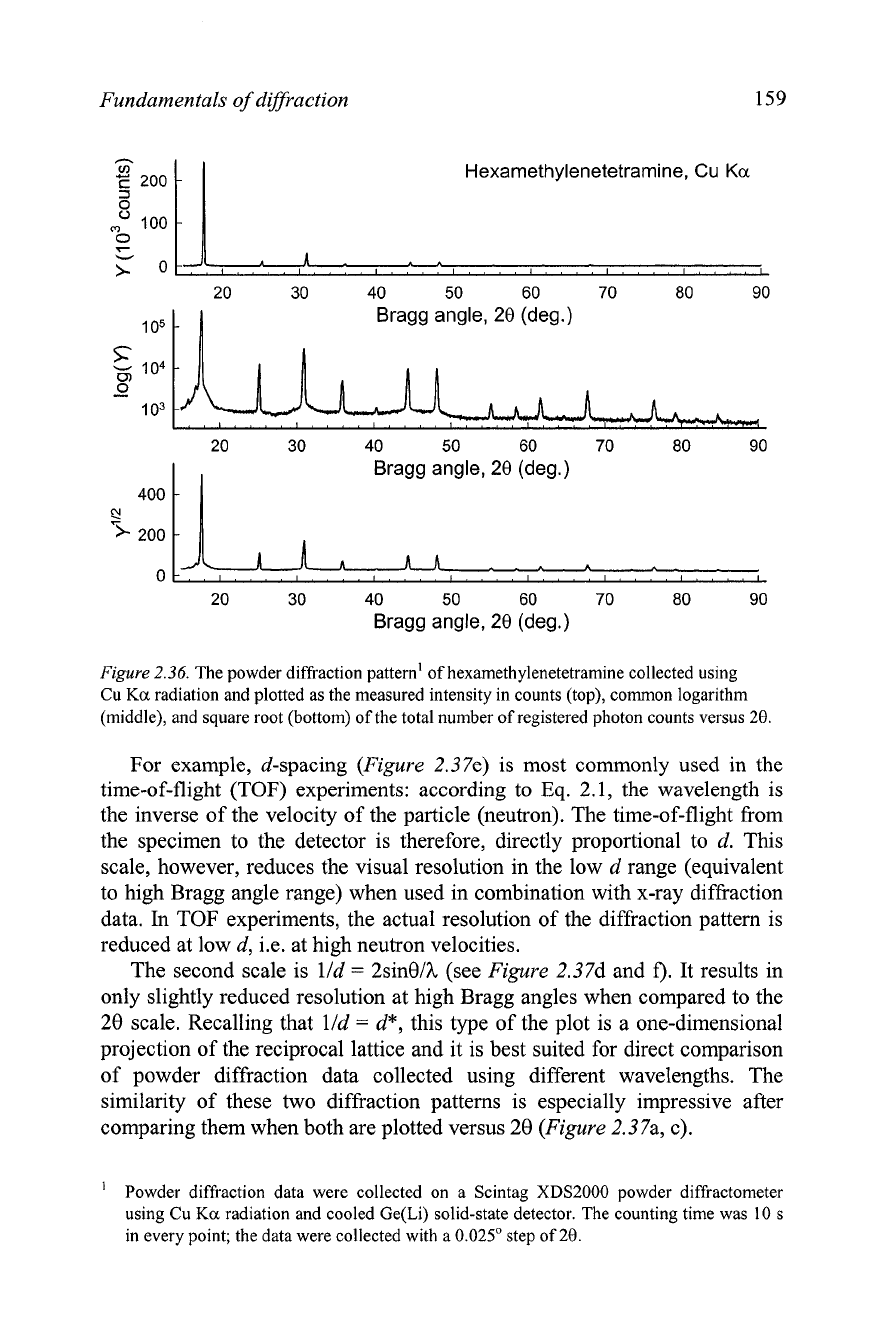
In a typical experiment the intensity, diffracted by a polycrystalline

sample, is measured as a function of Bragg angle, 29. Hence, powder

diffraction patterns are usually plotted in the form of the measured intensity,

Y, as the dependent variable versus the Bragg angle as the independent

variable; see Figure 2.36, top and Figure

2.37a, c. In rare instances, for

example when there are just a few very intense Bragg peaks and all others

are quite weak, or when it is necessary to directly compare diffraction

patterns collected from the same material using different wavelengths, the

scales of one or both axes may be modified for better viewing and easier

comparison.

When the first is true

(i.e. there are few extremely strong Bragg peaks

while all others are weak), the vertical axis can be calibrated as a logarithm

of intensity (Figure 2.36, middle) or its square root (Figure 2.36, bottom).

This changes the scale and enables better visualization of the low-intensity

features.

the example shown in Figure 2.36, the middle (logarithmic) plot

reveals all weak Bragg peaks in addition to the nonlinearity of the

background and the details of the intensity distribution around the bases of

the strongest peaks. The

Y'"

scale is equivalent to the plot of statistical errors

of the measured intensities (see Chapter 3, section 3.7.1), in addition to

better visualization of weak Bragg peaks.

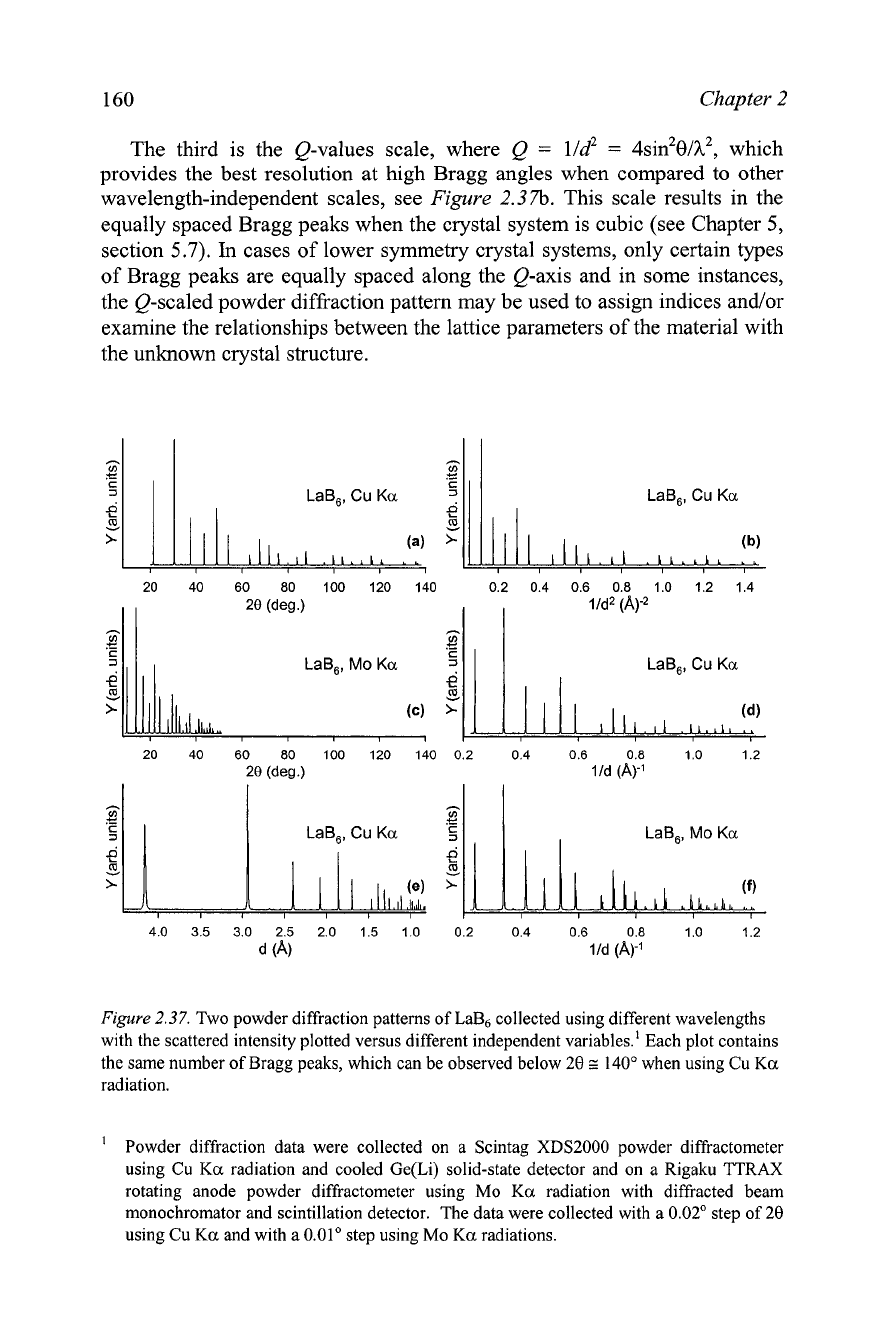
Various horizontal scales alternative to the Bragg angle, see Figure 2.37,

are usually wavelength-independent and their use is mostly dictated by

special circumstances.

Fundamentals of diffvaction 159

20 30 40 50 60 70 80 90

.Io5

Bragg angle, 28 (deg.)

20 30

50 60 70 80 90

Bragg angle, 29 (deg.)

200

20 30 40 50 60 70 80 90

Bragg angle, 28 (deg.)

Figure

2.36.

The powder diffraction pattern' of hexamethylenetetramine collected using

Cu Ka radiation and plotted as the measured intensity in counts (top), common logarithm

(middle), and square root (bottom) of the total number of registered photon counts versus

20.

For example, d-spacing (Figure 2.37e) is most commonly used in the

time-of-flight (TOF) experiments: according to Eq. 2.1, the wavelength is

the inverse of the velocity of the particle (neutron). The time-of-flight

from

the specimen to the detector is therefore, directly proportional to d. This

scale, however, reduces the visual resolution in the low d range (equivalent

to high Bragg angle range) when used in combination with x-ray diffraction

data.

TOF

experiments, the actual resolution of the diffraction pattern is

reduced at low d, i.e. at high neutron velocities.

The second scale is lld

2sin9lh (see Figure 2.37d and

f).

It results in

only slightly reduced resolution at high Bragg angles when compared to the

29 scale. Recalling that lld

d*, this type of the plot is a one-dimensional

projection of the reciprocal lattice and it is best suited for direct comparison

of powder diffraction data collected using different wavelengths. The

similarity of these two diffraction patterns is especially impressive after

comparing them when both are plotted versus 29 (Figure 2.3 7a, c).

Powder diffraction data were collected on a Scintag XDS2000 powder diffractometer

using Cu

radiation and cooled Ge(Li) solid-state detector. The counting time was 10 s

in every point; the data were collected with a 0.025' step of

20.

Chapter

The third is the Q-values scale, where Q

lld2

4~in~8/~~, which

provides the best resolution at high Bragg angles when compared to other

wavelength-independent scales, see

Figure

2.37b.

This scale results in the

equally spaced Bragg peaks when the crystal system is cubic (see Chapter

section

5.7).

cases of lower symmetry crystal systems, only certain types

of Bragg peaks are equally spaced along the Q-axis and in some instances,

the Q-scaled powder diffraction pattern may be used to assign indices

andor

examine the relationships between the lattice parameters of the material with

the unknown crystal structure.

20 40 60 80 100

120 140

0.2 0.4

(deg.)

0.6 0.8

1.0

1.2

lld

(A)-'

Figure

2.37.

Two powder diffraction patterns of La& collected using different wavelengths

with the scattered intensity plotted versus different independent variables.' Each plot contains

the same number of Bragg peaks, which can be observed below

140" when using Cu

radiation.

Powder diffraction data were collected on a Scintag XDS2000 powder diffractometer

using Cu

radiation and cooled Ge(Li) solid-state detector and on a Rigaku

TTRAX

rotating anode powder diffractometer using Mo

radiation with diffracted beam

monochromator and scintillation detector. The data were collected with a 0.02' step of

using Cu

and with a 0.01" step using Mo

radiations.