Moran M.J., Shapiro H.N. Fundamentals of Engineering Thermodynamics

Подождите немного. Документ загружается.

consisting of a system and that portion of the surroundings affected by the system as it

undergoes a process. Since all energy and mass transfers taking place are included within

the boundary of the enlarged system, the enlarged system is an isolated system.

An energy balance for the isolated system reduces to

¢E

isol

5 0 (6.29a)

because no energy transfers take place across its boundary. Thus, the energy of the

isolated system remains constant. Since energy is an extensive property, its value for

the isolated system is the sum of its values for the system and surroundings, respec-

tively, so Eq. 6.29a can be written as

¢E

stem

1 ¢E

surr

5 0 (6.29b)

In either of these forms, the conservation of energy principle places a constraint on

the processes that can occur. For a process to take place, it is necessary for the energy

of the system plus the surroundings to remain constant. However, not all processes

for which this constraint is satisfied can actually occur. Processes also must satisfy the

second law, as discussed next.

An entropy balance for the isolated system reduces to

¢S4

isol

1 s

isol

¢S

isol

5 s

isol

(6.30a)

where s

isol

is the total amount of entropy produced within the system and its sur-

roundings. Since entropy is produced in all actual processes, the only processes that

can occur are those for which the entropy of the isolated system increases. This is

known as the increase of entropy principle. The increase of entropy principle is some-

times considered an alternative statement of the second law.

Since entropy is an extensive property, its value for the isolated system is the sum of

its values for the system and surroundings, respectively, so Eq. 6.30a can be written as

¢S

stem

1 ¢S

surr

5 s

isol

(6.30b)

Notice that this equation does not require the entropy change to be positive for both

the system and surroundings but only that the sum of the changes is positive. In either

of these forms, the increase of entropy principle dictates the direction in which any

process can proceed: Processes occur only in such a direction that the total entropy

of the system plus surroundings increases.

We observed previously the tendency of systems left to themselves to undergo

processes until a condition of equilibrium is attained (Sec. 5.1). The increase of

entropy principle suggests that the entropy of an isolated system increases as the state

of equilibrium is approached, with the equilibrium state being attained when the

entropy reaches a maximum. This interpretation is considered again in Sec. 14.1, which

deals with equilibrium criteria.

Example 6.5 illustrates the increase of entropy principle.

6.8 Directionality of Processes 303

increase of entropy

principle

Quenching a Hot Metal Bar

c c c c EXAMPLE 6.5 c

A 0.8-lb metal bar initially at 19008R is removed from an oven and quenched by immersing it in a closed tank

containing 20 lb of water initially at 5308R. Each substance can be modeled as incompressible. An appropriate

constant specific heat value for the water is c

5 1.0 Btu/lb

8R, and an appropriate value for the metal is c

0.1 Btu/lb

8R, Heat transfer from the tank contents can be neglected. Determine (a) the final equilibrium tem-

perature of the metal bar and the water, in 8R, and (b) the amount of entropy produced, in Btu/8R.

c06UsingEntropy.indd Page 303 5/26/10 2:40:54 PM user-s146c06UsingEntropy.indd Page 303 5/26/10 2:40:54 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

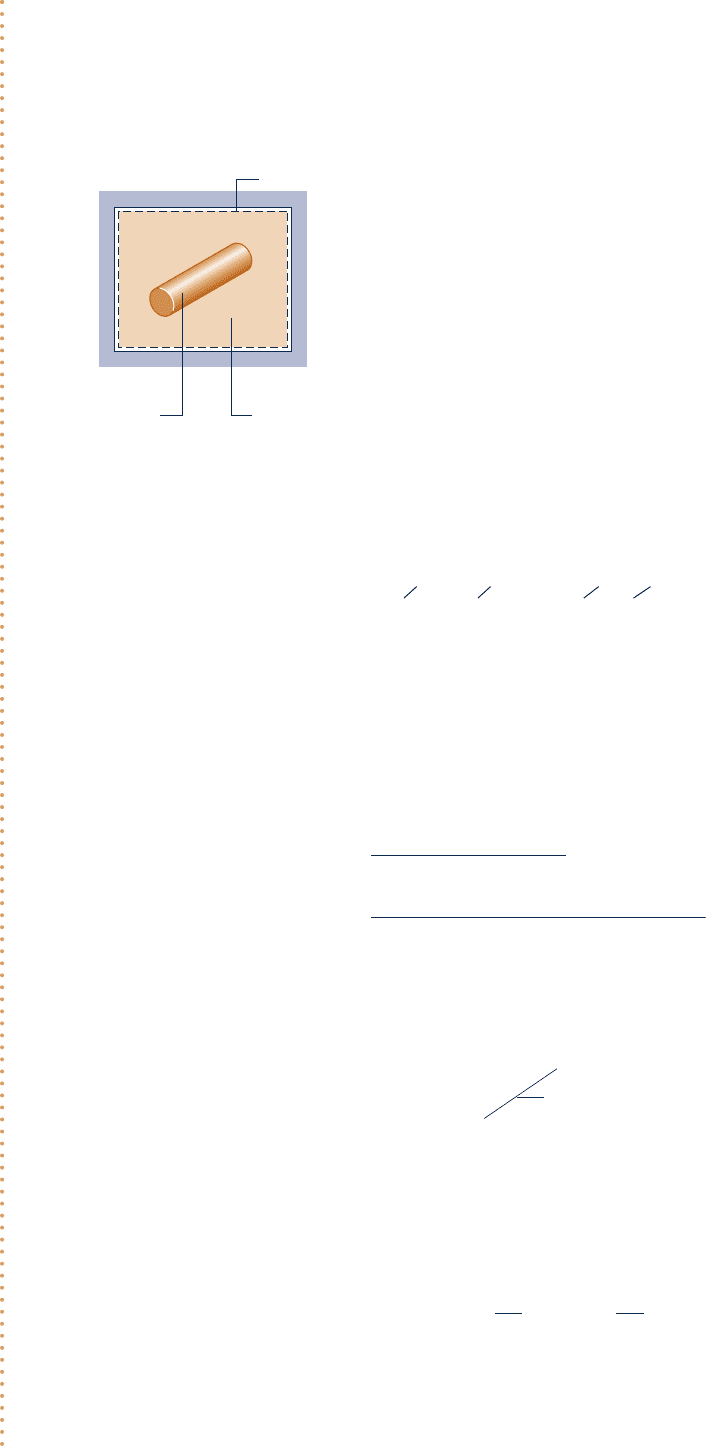
304 Chapter 6 Using Entropy

SOLUTION

Known:

A hot metal bar is quenched by immersing it in a closed tank containing water.

Find: Determine the final equilibrium temperature of the metal bar and the water, and the amount of entropy

produced.

Schematic and Given Data:

Analysis:

(a)

The final equilibrium temperature can be evaluated from an energy balance for the isolated system

¢KE

1 ¢PE

1 ¢U 5 Q

2 W

where the indicated terms vanish by assumptions 2 and 3. Since internal energy is an extensive property, its value for

the isolated system is the sum of the values for the water and metal, respectively. Thus, the energy balance becomes

¢U4

water

1 ¢U4

metal

5 0

Using Eq. 3.20a to evaluate the internal energy changes of the water and metal in terms of the constant specific heats

2 T

21 m

2 T

25 0

where T

is the final equilibrium temperature, and T

and T

are the initial temperatures of the water and metal,

respectively. Solving for T

and inserting values

1 m

21 m

120 lb2110215308R21 10.8 lb2119008R

120 lb211021 10.8 lb2

5 5358R

(b) The amount of entropy production can be evaluated from an entropy balance. Since no heat transfer occurs

between the isolated system and its surroundings, there is no accompanying entropy transfer, and an entropy

balance for the isolated system reduces to

¢S 5

1 s

Entropy is an extensive property, so its value for the isolated system is the sum of its values for the water and

the metal, respectively, and the entropy balance becomes

¢S4

water

1 ¢S4

metal

5 s

Evaluating the entropy changes using Eq. 6.13 for incompressible substances, the foregoing equation can be

written as

s 5 m

1 m

Engineering Model:

The metal bar and the water within the tank form a system, as

shown on the accompanying sketch.

2. There is no energy transfer by heat or work: The system is

isolated.

3. There is no change in kinetic or potential energy.

4. The water and metal bar are each modeled as incompressible

with known specific heats.

Fig. E6.5

System boundary

Water:

= 530°R

= 1.0 Btu/lb·°R

= 20 lb

Metal bar:

= 1900°R

= 0.1 Btu/lb·°R

= 0.8 lb

c06UsingEntropy.indd Page 304 5/26/10 5:20:26 PM user-s146c06UsingEntropy.indd Page 304 5/26/10 5:20:26 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

6.8.2

Statistical Interpretation of Entropy

Building on the increase of entropy principle, in this section we

introduce an interpretation of entropy from a microscopic perspec-

tive based on probability.

In statistical thermodynamics, entropy is associated with the notion

of microscopic disorder. From previous considerations we know that

in a spontaneous process of an isolated system, the system moves

toward equilibrium and the entropy increases. From the microscopic

viewpoint, this is equivalent to saying that as an isolated system moves

toward equilibrium our knowledge of the condition of individual par-

ticles making up the system decreases, which corresponds to an

increase in microscopic disorder and a related increase in entropy.

We use an elementary thought experiment to bring out some

basic ideas needed to understand this view of entropy. Actual micro-

scopic analysis of systems is more complicated than the discussion

given here, but the essential concepts are the same.

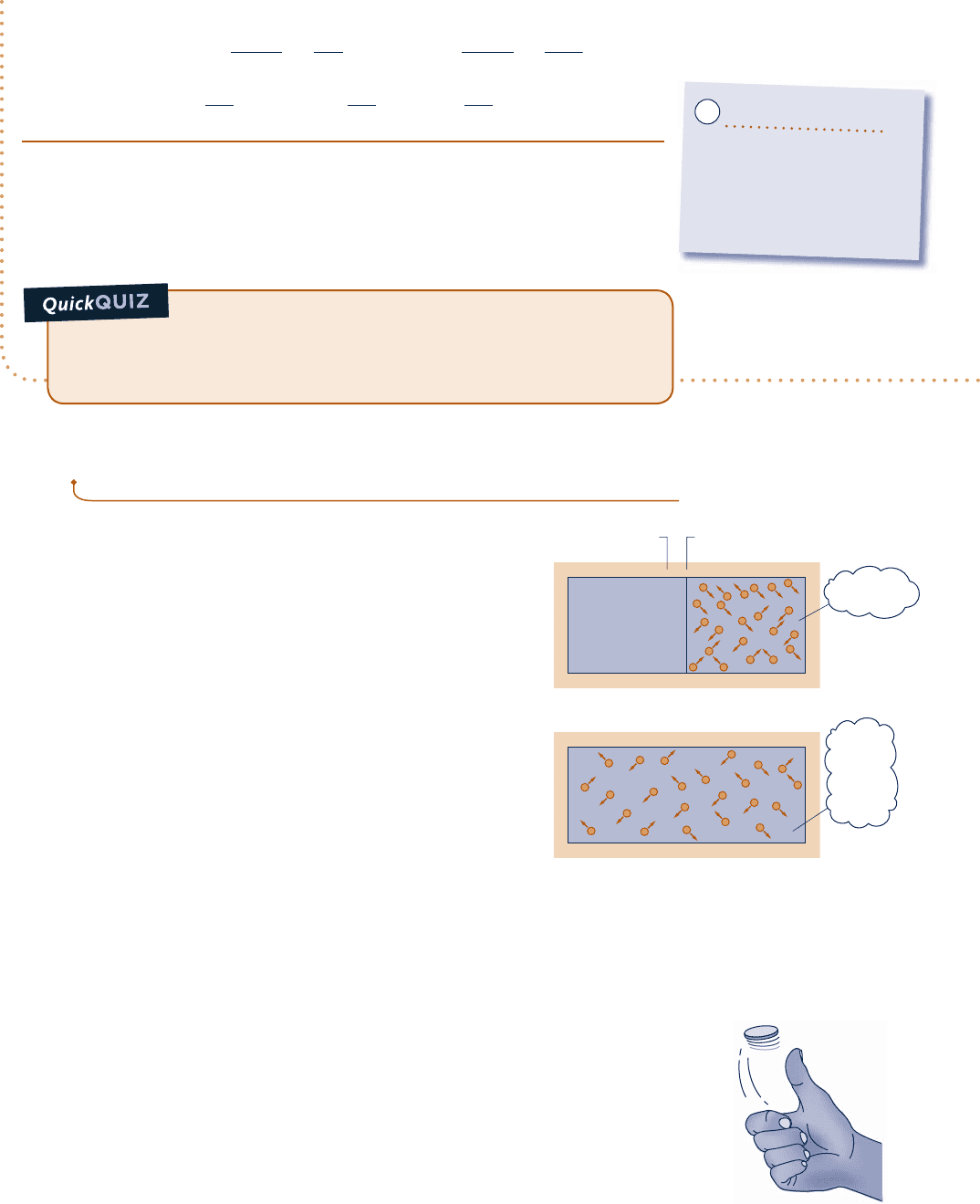
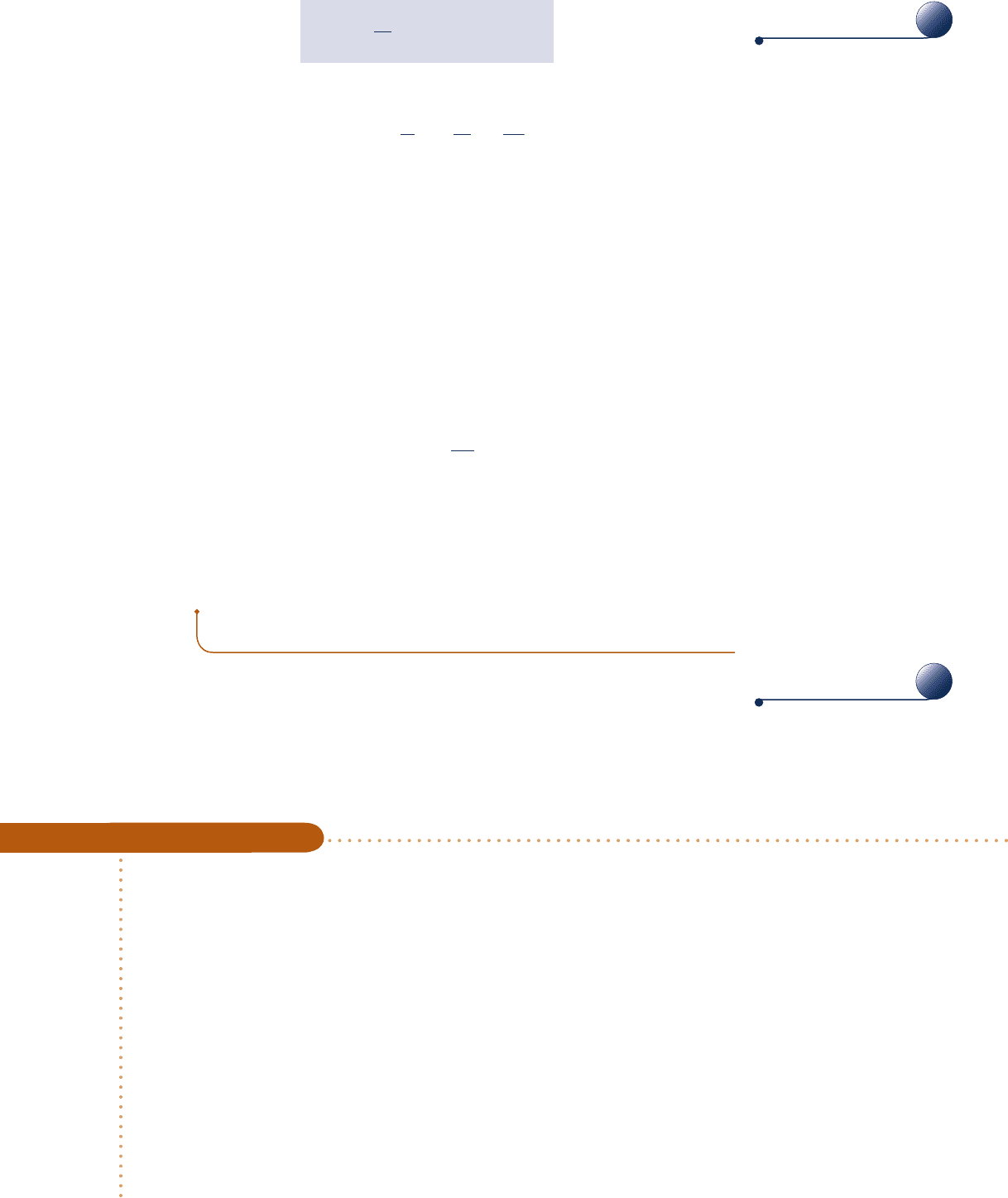
Consider N molecules initially contained in one half of the box

shown in Fig. 6.7a. The entire box is considered an isolated system.

We assume that the ideal gas model applies. In the initial condition,

the gas appears to be at equilibrium in terms of temperature, pressure,

and other properties. But, on the microscopic level the molecules are moving about

randomly. We do know for sure, though, that initially all molecules are on the right

side of the vessel.

Suppose we remove the partition and wait until equilibrium is reached again, as

in Fig. 6.7b. Because the system is isolated, the internal energy U does not change:

5 U

. Further, because the internal energy of an ideal gas depends on temperature

alone, the temperature is unchanged: T

5 T

. Still, at the final state a given molecule

has twice the volume in which to move: V

5 2V

. Just like a coin toss, the probability

that the molecule is on one side or the other is now ½, which is the same as the

volume ratio V

. In the final condition, we have less knowledge about where each

molecule is than we did originally.

We can evaluate the change in entropy for the process of Fig. 6.7 by applying

Eq. 6.17, expressed in terms of volumes and on a molar basis. The entropy change for

If the mass of the metal bar were 0.45 lb, determine the final

equilibrium temperature, in 8R, and the amount of entropy produced, in

Btu/8R, keeping all other given data the same. Ans. 5338R, 0.0557

Btu/8R.

Inserting values

s 5 120 lb2a1.0

Btu

lb ? 8R

b ln

535

530

1 10.8 lb2a0.1

Btu

lb ? 8R

b ln

535

1900

➊➋

5 a0.1878

Btu

b1 a20.1014

Btu

b5 0.0864

Btu

➊

The metal bar experiences a decrease in entropy. The entropy of the water

increases. In accord with the increase of entropy principle, the entropy of the

isolated system increases.

➋

The value of

is sensitive to roundoff in the value of T

Ability to…

❑

apply the closed system

energy and entropy balances.

❑

apply the incompressible

substance model.

✓

Skills Developed

6.8 Directionality of Processes 305

Fig. 6.7

N molecules a box.

(a)

(b)

, T

, V

, S

Insulation Partition

Initially

Evacuated

= U

= T

= 2V

> S

c06UsingEntropy.indd Page 305 7/1/10 8:54:09 AM user-s146c06UsingEntropy.indd Page 305 7/1/10 8:54:09 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

306 Chapter 6

Using Entropy

the constant-temperature process is

2 S

n 5 R ln1V

2 (6.31)

where n is the amount of substance on a molar basis (Eq. 1.8). Next, we consider how

the entropy change would be evaluated from a microscopic point of view.

Through more complete molecular modeling and statistical analysis, the total num-

ber of positions and velocities 2 microstates 2 available to a single molecule can be

calculated. This total is called the thermodynamic probability, w. For a system of N

molecules, the thermodynamic probability is w

. In statistical thermodynamics, entropy

is considered to be proportional to ln(w)

. That is, S ~ N ln(w). This gives the

Boltzmann relation

N 5 k ln w (6.32)

where the proportionality factor, k, is called Boltzmann’s constant.

Applying Eq. 6.32 to the process of Fig. 6.7, we get

2 S

N 5 k ln

2 k ln

5 k ln

(6.33)

Comparing Eqs. 6.31 and 6.33, the expressions for entropy change coincide when

k 5 nR

and w

5 V

. The first of these expressions allows Boltzmann’s constant

to be evaluated, giving k 5 1.3806 3 10

223

J/K. Also, since V

. V

and w

. w

, Eqs. 6.31

and 6.33 each predict an increase of entropy owing to entropy production during the

irreversible adiabatic expansion in this example.

From Eq. 6.33, we see that any process that increases the number of possible

microstates of a system increases its entropy and conversely. Hence, for an isolated

system, processes occur only in such a direction that the number of microstates avail-

able to the system increases, resulting in our having less knowledge about the condi-

tion of individual particles. Because of this concept of decreased knowledge, entropy

reflects the microscopic disorder of the system. We can then say that the only pro-

cesses an isolated system can undergo are those that increase the disorder of the

system. This interpretation is consistent with the idea of directionality of processes

discussed previously.

The notion of entropy as a measure of disorder is sometimes used in fields other

than thermodynamics. The concept is employed in information theory, statistics, biol-

ogy, and even in some economic and social modeling. In these applications, the term

entropy is used as a measure of disorder without the physical aspects of the thought

experiment used here necessarily being implied.

Some 135 years ago, renowned nineteenth-century

physicist J. C. Maxwell wrote, “. . . the second law is

. . . a statistical . . . truth, for it depends on the fact that

the bodies we deal with consist of millions of molecules . . . [Still]

the second law is continually being violated . . . in any suffi-

ciently small group of molecules belonging to a real body.”

Although Maxwell’s view was bolstered by theorists over the

years, experimental confirmation proved elusive. Then, in 2002,

experimenters reported they had demonstrated violations of the

second law: at the micron scale over time intervals of up to 2

seconds, entropy was consumed, not produced [see Phys. Rev.

Lett. 89, 050601 (2002)].

While few were surprised that experimental confirmation had

at last been achieved, some were surprised by implications of

the research for the twenty-first-century field of nanotechnology:

The experimental results suggest inherent limitations on nano-

machines. These tiny devices—only a few molecules in size—may

not behave simply as miniaturized versions of their larger coun-

terparts; and the smaller the device, the more likely its motion

and operation could be disrupted unpredictably. Occasionally

and uncontrollably, nanomachines may not perform as designed,

perhaps even capriciously running backward. Still, designers of

these machines will applaud the experimental results if they lead

to deeper understanding of behavior at the nanoscale.

“Breaking” the Second Law Has Implications for Nanotechnology

microstates

thermodynamic probability

Boltzmann relation

disorder

c06UsingEntropy.indd Page 306 5/26/10 2:41:03 PM user-s146c06UsingEntropy.indd Page 306 5/26/10 2:41:03 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

BIOCONNECTIONS Do living things violate the second law of thermody-

namics because they seem to create order from disorder? Living things are not iso-

lated systems as considered in the previous discussion of entropy and disorder.

Living things interact with their surroundings and are influenced by their surroundings. For

instance, plants grow into highly ordered cellular structures synthesized from atoms and

molecules originating in the earth and its atmosphere. Through interactions with their sur-

roundings, plants exist in highly organized states and are able to produce within them-

selves even more organized, lower entropy states. In keeping with the second law, states

of lower entropy can be realized within a system as long as the total entropy of the system

and its surroundings increases. The self-organizing tendency of living things is widely

observed and fully in accord with the second law.

6.9 Entropy Rate Balance for Control

Volumes

Thus far the discussion of the entropy balance concept has been restricted to the case

of closed systems. In the present section the entropy balance is extended to control

volumes.

Like mass and energy, entropy is an extensive property, so it too can be transferred

into or out of a control volume by streams of matter. Since this is the principal dif-

ference between the closed system and control volume forms, the control volume

entropy rate balance

can be obtained by modifying Eq. 6.28 to account for these

entropy transfers. The result is

1 s

(6.34)

rate of rates of rate of

entropy entropy entropy

change transfer production

where dS

/dt represents the time rate of change of entropy within the control volume.

The terms m

and m

account, respectively, for rates of entropy transfer accompany-

ing mass flow

into and out of the control volume. The term Q

represents the time

rate of heat transfer at the location on the boundary where the instantaneous tem-

perature is T

. The ratio Q

accounts for the accompanying rate of entropy transfer.

The term s

denotes the time rate of entropy production due to irreversibilities

within the control volume.

Integral Form of the Entropy Rate Balance

As for the cases of the control volume mass and energy rate balances, the entropy

rate balance can be expressed in terms of local properties to obtain forms that are

more generally applicable. Thus, the term S

(t), representing the total entropy associ-

ated with the control volume at time t, can be written as a volume integral

1t25

rs d

where r and s denote, respectively, the local density and specific entropy. The rate of

entropy transfer accompanying heat transfer can be expressed more generally as an

integral over the surface of the control volume

time rate of entropy

transfer accompanying

heat transfer

control volume entropy

rate balance

entropy transfer

accompanying mass flow

6.9 Entropy Rate Balance for Control Volumes 307

Entropy_Rate_Bal_CV

A.25 – Tabs a & b

c06UsingEntropy.indd Page 307 6/30/10 9:45:36 AM user-s146c06UsingEntropy.indd Page 307 6/30/10 9:45:36 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

308 Chapter 6 Using Entropy

where q

is the heat flux, the time rate of heat transfer per unit of surface area, through

the location on the boundary where the instantaneous temperature is T. The subscript

“b” is added as a reminder that the integrand is evaluated on the boundary of the

control volume. In addition, the terms accounting for entropy transfer accompanying

mass flow can be expressed as integrals over the inlet and exit flow areas, resulting

in the following form of the entropy rate balance

rs dV 5

dA 1

srV

1 s

(6.35)

where V

denotes the normal component in the direction of flow of the velocity

relative to the flow area. In some cases, it is also convenient to express the entropy

production rate as a volume integral of the local volumetric rate of entropy produc-

tion within the control volume. The study of Eq. 6.35 brings out the assumptions

underlying Eq. 6.34. Finally, note that for a closed system the sums accounting for

entropy transfer at inlets and exits drop out, and Eq. 6.35 reduces to give a more

general form of Eq. 6.28.

6.10 Rate Balances for Control Volumes

at Steady State

Since a great many engineering analyses involve control volumes at steady state, it is

instructive to list steady-state forms of the balances developed for mass, energy, and

entropy. At steady state, the conservation of mass principle takes the form

(4.6)

The energy rate balance at steady state is

0 5 Q

2 W

1 gz

(4.18)

Finally, the steady-state form of the entropy rate balance is obtained by reducing

Eq. 6.34 to give

0 5

1 s

(6.36)

These equations often must be solved simultaneously, together with appropriate prop-

erty relations.

Mass and energy are conserved quantities, but entropy is not conserved. Equation 4.6

indicates that at steady state the total rate of mass flow into the control volume equals

the total rate of mass flow out of the control volume. Similarly, Eq. 4.18 indicates

that the total rate of energy transfer into the control volume equals the total rate of

energy transfer out of the control volume. However, Eq. 6.36 requires that the rate

at which entropy is transferred out must exceed the rate at which entropy enters, the

difference being the rate of entropy production within the control volume owing to

irreversibilities.

6.10.1

One-Inlet, One-Exit Control Volumes at Steady State

Since many applications involve one-inlet, one-exit control volumes at steady state, let

us also list the form of the entropy rate balance for this important case. Thus, Eq. 6.36

reduces to read

steady-state entropy

rate balance

c06UsingEntropy.indd Page 308 5/26/10 2:41:05 PM user-s146c06UsingEntropy.indd Page 308 5/26/10 2:41:05 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

0 5

1 m

2 s

21 s

(6.37)

Or, on dividing by the mass flow rate m

and rearranging

2 s

(6.38)

The two terms on the right side of Eq. 6.38 denote, respectively, the rate of entropy

transfer accompanying heat transfer and the rate of entropy production within the

control volume, each per unit of mass flowing through the control volume. From Eq. 6.38

it can be concluded that the entropy of a unit of mass passing from inlet to exit can

increase, decrease, or remain the same. Furthermore, because the value of the second

term on the right can never be negative, a decrease in the specific entropy from inlet

to exit can be realized only when more entropy is transferred out of the control

volume accompanying heat transfer than is produced by irreversibilities within the

control volume. When the value of this entropy transfer term is positive, the specific

entropy at the exit is greater than the specific entropy at the inlet whether internal

irreversibilities are present or not. In the special case where there is no entropy

transfer accompanying heat transfer, Eq. 6.38 reduces to

2 s

(6.39)

Accordingly, when irreversibilities are present within the control volume, the entropy

of a unit of mass increases as it passes from inlet to exit. In the limiting case in which

no irreversibilities are present, the unit mass passes through the control volume with

no change in its entropy—that is, isentropically.

6.10.2

Applications of the Rate Balances to Control Volumes

at Steady State

The following examples illustrate the use of the mass, energy, and entropy balances

for the analysis of control volumes at steady state. Carefully note that property rela-

tions and property diagrams also play important roles in arriving at solutions.

In Example 6.6, we evaluate the rate of entropy production within a turbine oper-

ating at steady state when there is heat transfer from the turbine.

6.10 Rate Balances for Control Volumes at Steady State 309

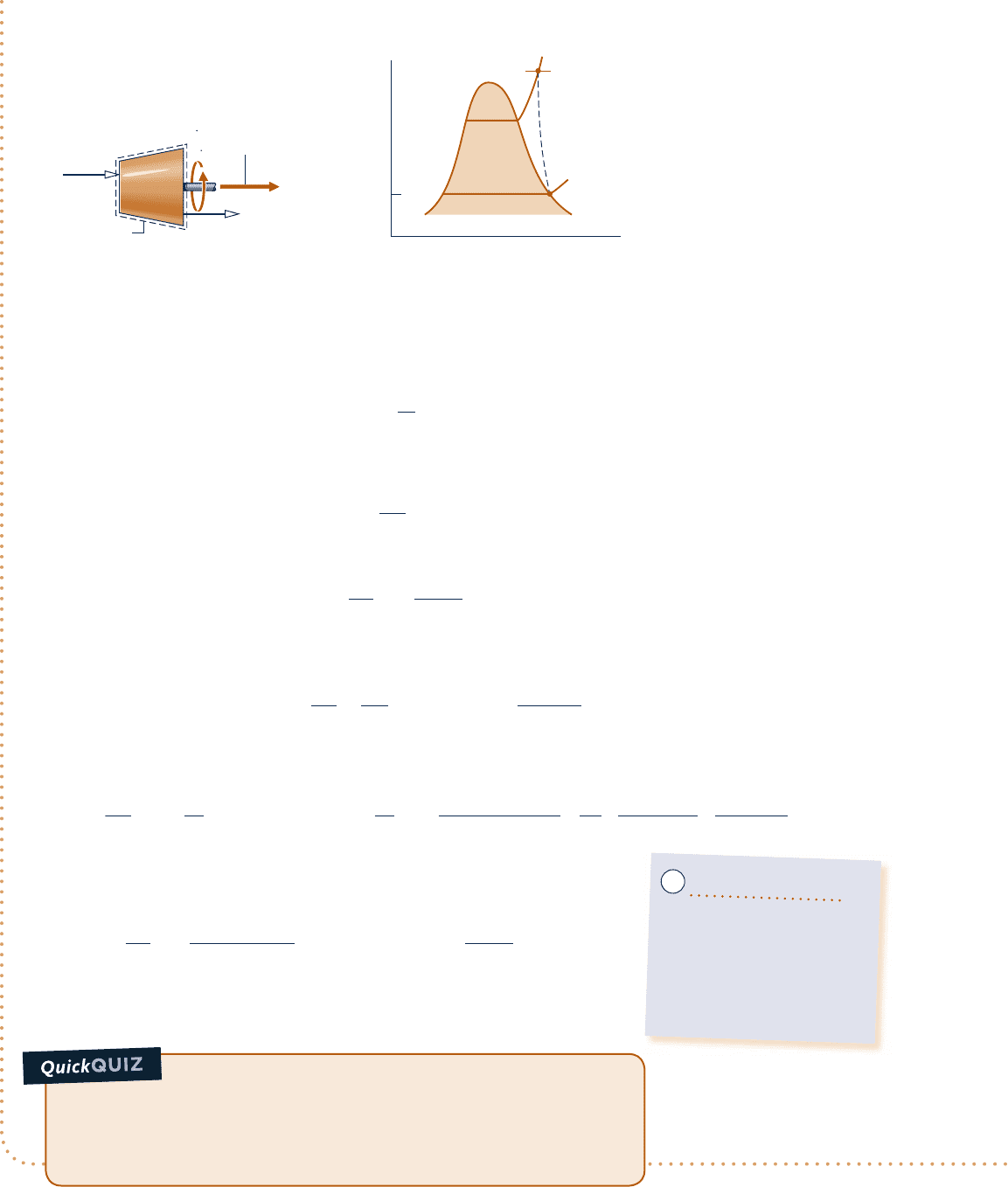
Determining Entropy Production in a Steam Turbine

c c c c EXAMPLE 6.6 c

Steam enters a turbine with a pressure of 30 bar, a temperature of 4008C, and a velocity of 160 m/s. Saturated

vapor at 1008C exits with a velocity of 100 m/s. At steady state, the turbine develops work equal to 540 kJ per kg

of steam flowing through the turbine. Heat transfer between the turbine and its surroundings occurs at an aver-

age outer surface temperature of 350 K. Determine the rate at which entropy is produced within the turbine per kg

of steam flowing, in kJ/kg

K. Neglect the change in potential energy between inlet and exit.

SOLUTION

Known:

Steam expands through a turbine at steady state for which data are provided.

Find: Determine the rate of entropy production per kg of steam flowing.

Entropy_Rate_Bal_CV

A.25 – Tab c

Turbine

A.19 – Tab d

c06UsingEntropy.indd Page 309 6/30/10 11:30:09 AM user-s146c06UsingEntropy.indd Page 309 6/30/10 11:30:09 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

310 Chapter 6 Using Entropy

Analysis: To determine the entropy production per unit mass flowing through the turbine, begin with mass and

entropy rate balances for the one-inlet, one-exit control volume at steady state:

5 m

0 5

1 m

2 m

1 s

Since heat transfer occurs only at T

5 350 K, the first term on the right side of the entropy rate balance reduces

to Q

. Combining the mass and entropy rate balances

0 5

1 m

2 s

21 s

where m

is the mass flow rate. Solving for s

1 1s

2 s

The heat transfer rate, Q

, required by this expression is evaluated next.

Reduction of the mass and energy rate balances results in

1 1h

2 h

21 a

2 V

where the potential energy change from inlet to exit is dropped by assumption 3. From Table A-4 at 30 bar,

4008C, h

5 3230.9 kJ/kg, and from Table A-2, h

5 h

(1008C) 5 2676.1 kJ/kg. Thus

5 540

1 12676.1 2 3230.92a

b1 c

11002

2 11602

1 N

1 kg ? m

1 kJ

N ? m

5 540 2 554.8 2 7.8 5222.6 kJ

From Table A-2, s

5 7.3549 kJ/kg

K, and from Table A-4, s

5 6.9212 kJ/

K. Inserting values into the expression for entropy production

222.6 kJ

350 K

1 17.3549 2 6.92122

kg ? K

5 0.0646 1 0.4337 5 0.498 kJ

? K

If the boundary were located to include the turbine and a por-

tion of the immediate surroundings so heat transfer occurs at the tem-

perature of the surroundings, 293 K, determine the rate at which entropy

is produced within the enlarged control volume, in kJ/K per kg of steam

flowing, keeping all other given data the same. Ans. 0.511 kJ/kg

Ability to…

❑

apply the control volume

mass, energy and entropy

rate balances.

❑

retrieve property data for

water.

✓

Skills Developed

Engineering Model:

The control volume shown

on the accompanying

sketch is at steady state.

2. Heat transfer from the

turbine to the surroundings

occurs at a specified average

outer surface temperature.

3. The change in potential

energy between inlet and

exit can be neglected.

= 30 bar

= 400°C

= 160 m/s

Surroundings

at 293 K

= 100°C

Saturated vapor

= 100 m/s

–––

= 540 kJ/kg

= 350 K

400°C

30 bar

100°C

Fig. E6.6

Schematic and Given Data:

c06UsingEntropy.indd Page 310 5/26/10 2:41:06 PM user-s146c06UsingEntropy.indd Page 310 5/26/10 2:41:06 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

In Example 6.7, the mass, energy, and entropy rate balances are used to evaluate

a performance claim for a device producing hot and cold streams of air from a single

stream of air at an intermediate temperature.

6.10 Rate Balances for Control Volumes at Steady State 311

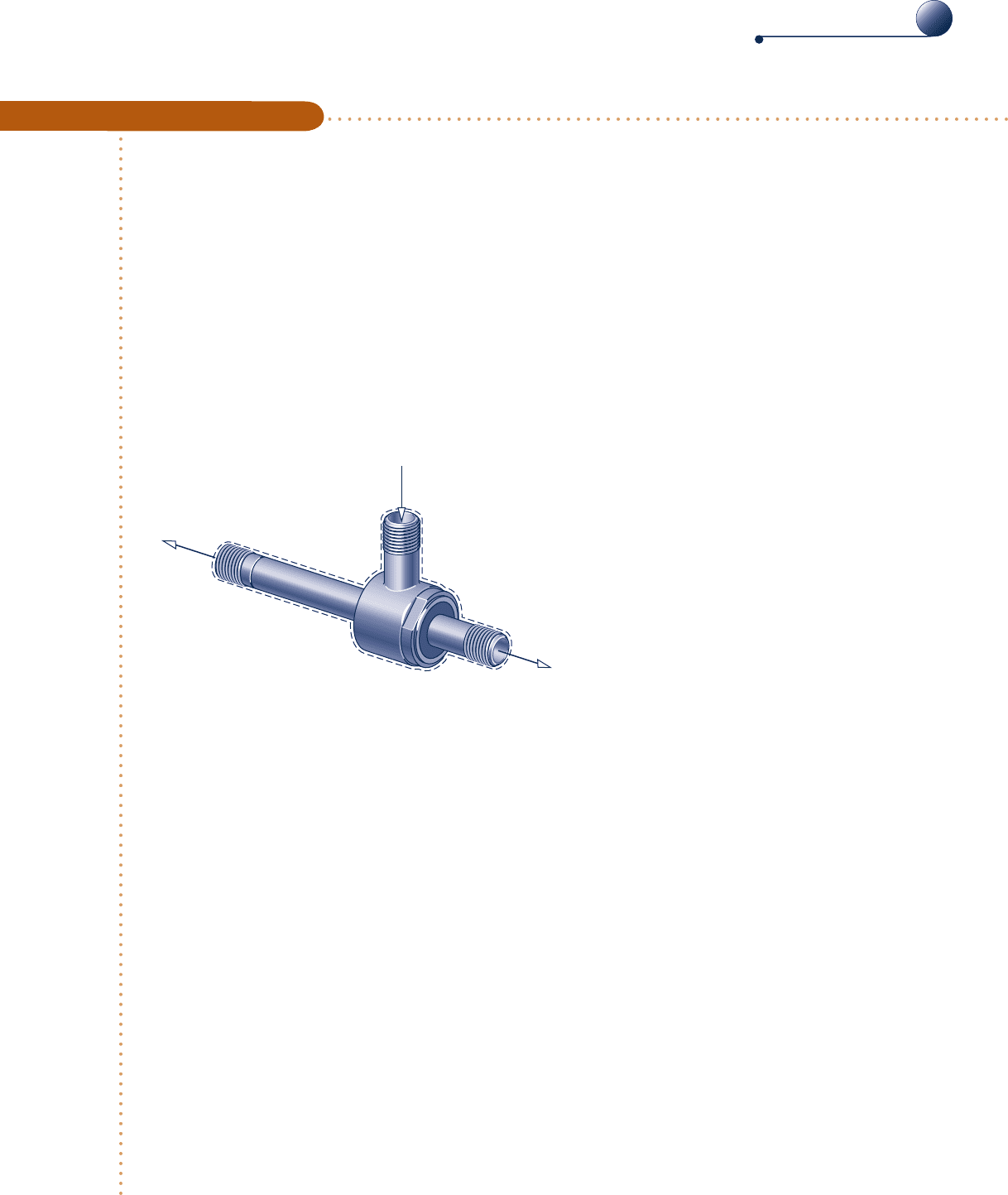
Evaluating a Performance Claim

c c c c EXAMPLE 6.7 c

An inventor claims to have developed a device requiring no energy transfer by work, W

, or heat transfer, yet able

to produce hot and cold streams of air from a single stream of air at an intermediate temperature. The inventor

provides steady-state test data indicating that when air enters at a temperature of 708F and a pressure of 5.1 atm,

separate streams of air exit at temperatures of 0 and 1758F, respectively, and each at a pressure of 1 atm. Sixty per-

cent of the mass entering the device exits at the lower temperature. Evaluate the inventor’s claim, employing the

ideal gas model for air and ignoring changes in the kinetic and potential energies of the streams from inlet to exit.

SOLUTION

Known:

Data are provided for a device that at steady state produces hot and cold streams of air from a single

stream of air at an intermediate temperature without energy transfers by work or heat.

Find: Evaluate whether the device can operate as claimed.

Schematic and Given Data:

= 70°F

= 5.1 atm

= 0°F

= 1 atm

= 175°F

= 1 atm

Inlet

Cold outlet

Hot outlet

Fig. E6.7

Analysis: For the device to operate as claimed, the conservation of mass and energy principles must be satisfied.

The second law of thermodynamics also must be satisfied; and in particular the rate of entropy production cannot

be negative. Accordingly, the mass, energy and entropy rate balances are considered in turn.

With assumptions 1–3, the mass and energy rate balances reduce, respectively, to

5 m

1 m

0 5 m

2 m

Since m

5 0.6m

, it follows from the mass rate balance that m

5 0.4m

. By combining the mass and energy

rate balances and evaluating changes in specific enthalpy using constant c

, the energy rate balance is also satis-

fied. That is

0 5

1 m

2 m

5 m

2 h

1 m

2 h

5 0.4m

2 T

1 0.6m

2 T

5 0.4

2 T

1 0.6

2 T

5 0.4

2105

1 0.6

➋

Engineering Model:

The control volume shown on the

accompanying sketch is at steady state.

2. For the control volume, W

5 0 and Q

5 0.

3. Changes in the kinetic and potential

energies from inlet to exit can be ignored.

4. The air is modeled as an ideal gas with

constant c

5 0.24 Btu/lb

8R.

➊

Heat_Exchanger

A.22 – Tab d

c06UsingEntropy.indd Page 311 6/30/10 11:24:49 AM user-s146c06UsingEntropy.indd Page 311 6/30/10 11:24:49 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

312 Chapter 6 Using Entropy

Accordingly, with the given data the conservation of mass and energy principles are satisfied.

Since no significant heat transfer occurs, the entropy rate balance at steady state reads

0 5

1 m

2 m

1 s

Combining the mass and entropy rate balances

0 5

1 m

2 m

1 s

5 m

2 s

1 m

2 s

1 s

5 0.4m

2 s

1 0.6m

2 s

1 s

Solving for s

and using Eq. 6.22 to evaluate changes in specific entropy

5 0.4 cc

2 R ln

d1 0.6 cc

2 R ln

5 0.4

0.24

Btu

lb ? 8R

635

530

1.986

28.97

Btu

lb ? 8R

5.1

1 0.6

0.24

Btu

lb ? 8R

460

530

1.986

28.97

Btu

lb ? 8R

5.1

5 0.1086

lb ? 8R

Thus, the second law of thermodynamics is also satisfied.

➎ On the basis of this evaluation, the inventor’s claim does not violate principles of thermodynamics.

➊ Since the specific heat c

of air varies little over the temperature interval from 0 to 1758F, c

can be taken as

constant. From Table A-20E, c

5 0.24 Btu/lb

8R.

➋ Since temperature differences are involved in this calculation, the temperatures can be either in 8R or 8F.

➌ In this calculation involving temperature ratios, the temperatures are in 8R.

Temperatures in 8F should not be used.

➍ If the value of the rate of entropy production had been negative or zero, the

claim would be rejected. A negative value is impossible by the second law and

a zero value would indicate operation without irreversibilities.

➎ Such devices do exist. They are known as vortex tubes and are used in indus-

try for spot cooling.

If the inventor would claim that the hot and cold streams exit

the device at 5.1 atm, evaluate the revised claim, keeping all other given

data the same. Ans. Claim invalid.

Ability to…

❑

apply the control volume

mass, energy and entropy

rate balances.

❑

apply the ideal gas model

with constant c

✓

Skills Developed

➌

➍

In Example 6.8, we evaluate and compare the rates of entropy production for

three components of a heat pump system. Heat pumps are considered in detail in

Chap. 10.

Compressor

A.20 – Tab d

Throttling_Dev

A.23 – Tab d

c06UsingEntropy.indd Page 312 6/30/10 11:24:23 AM user-s146c06UsingEntropy.indd Page 312 6/30/10 11:24:23 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New