Moran M.J., Shapiro H.N. Fundamentals of Engineering Thermodynamics
Подождите немного. Документ загружается.


576 Chapter 12 Ideal Gas Mixture and Psychrometric Applications
where p
N
2
2 atm is the initial pressure of the nitrogen and p
O
2
1 atm is the initial pressure of the oxygen. Combining
results and reducing
Substituting values
(c) Reducing the closed system form of the entropy balance
where the entropy transfer term drops out for the adiabatic mixing process. The initial entropy of the system, S
1
, is the sum
of the entropies of the gases at the respective initial states
The final entropy of the system, S
2
, is the sum of the entropies of the individual components, each evaluated at the final mix-
ture temperature and the partial pressure of the component in the mixture
Collecting the last three equations
Evaluating the change in specific entropy of each gas in terms of a constant specific heat this becomes
The required values for can be found by adding to the values found previously (Eq. 3.45)
Since the total number of moles of mixture n 0.79 0.21 1.0, the mole fractions of the two gases are y
N
2
0.79 and
y
O
2
0.21.
Substituting values into the expression for gives
Entropy is produced when different gases, initially at different temperatures and pressures, are followed to mix.
5.0 kJ/°K
0.21
kmol c29.30
kJ
kmol
#
K
ln
a
261°K
300°K
b 8.314
kJ
kmol
#
K
ln
a
10.212
11.62 bars2
1 bar
bd
s 0.79
kmol c29.13
kJ
kmol
#
K
ln
a
261°K
250°K
b 8.314
kJ
kmol
#
K
ln
a
10.792
11.62 bars2
2 bars
bd
c
p,N
2
29.13
kJ
kmol
#
K
c
p,O
2
29.30
kJ
kmol
#
°K
c
v
Rc
p
n
O
2
ac
p,O
2
ln
T
2
T
O
2
R ln
y
O
2
p
2
p
O
2
b
s n
N
2
ac
p,N
2
ln
T
2
T
N
2
R ln
y
N
2
p
2
p
N
2
b
c
p
,
n
O
2
3s
O
2
1T
2
, y
O
2
p
2
2 s
O
2
1T
O
2
, p
O
2
24
s n
N
2
3s
N
2
1T
2
, y
N
2
p
2
2 s
N
2
1T
N
2
, p
N
2
24
S
2
n
N
2
s
N
2
1T
2
, y
N
2
p
2
2 n
O
2
s
O
2
1T
2
, y
O
2
p
2
2
S
1
n
N
2
s
N
2
1T
N
2
, p
N
2
2 n
O
2
s
O
2
1T
O
2
, p
O
2
2
S
2
S
1
2
1
a
dQ
T
b
b
0
s
1.62 bars
p
2
11.0 kmol2
1261°K2
c
10.79
kmol2 1250°K2
2 bars
10.21
kmol2 1300°K2
1 bar
d
p
2
1n
N
2
n
O
2
2
T
2
a
n
N
2
T
N
2
p
N
2
n
O
2
T
O
2
p
O
2
b
❶
❶
12.4 Analyzing Systems Involving Mixtures 577
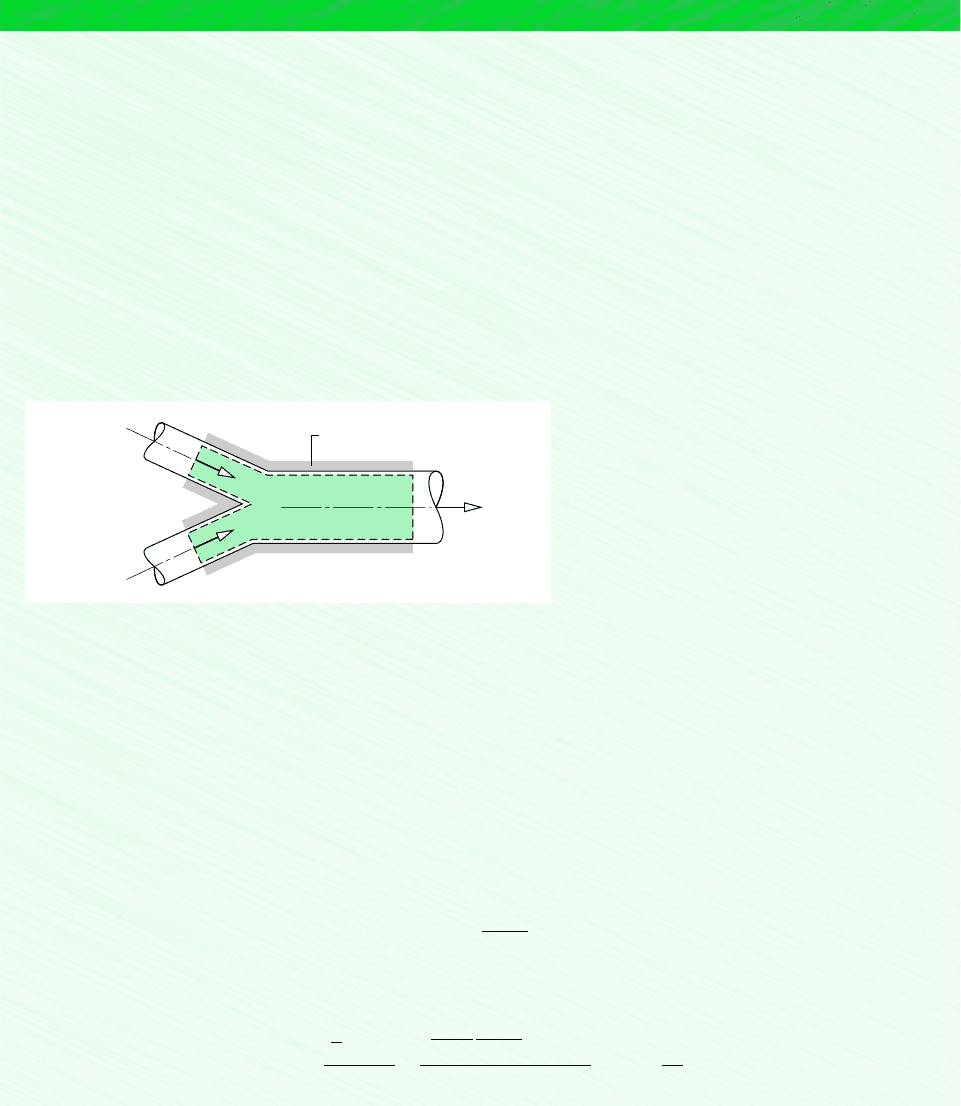
In the next example, we consider a control volume at steady state where two incoming
streams form a mixture. A single stream exits.
EXAMPLE 12.6 Adiabatic Mixing of Two Streams
At steady state, 100 m
3
/min of dry air at 32C and 1 bar is mixed adiabatically with a stream of oxygen (O
2
) at 127C and
1 bar to form a mixed stream at 47C and 1 bar. Kinetic and potential energy effects can be ignored. Determine (a) the mass
flow rates of the dry air and oxygen, in kg/min, (b) the mole fractions of the dry air and oxygen in the exiting mixture, and
(c) the time rate of entropy production, in
SOLUTION
Known: At steady state, 100 m
3
/min of dry air at 32C and 1 bar is mixed adiabatically with an oxygen stream at 127C
and 1 bar to form a mixed stream at 47C and 1 bar.
Find: Determine the mass flow rates of the dry air and oxygen, in kg/min, the mole fractions of the dry air and oxygen in
the exiting mixture, and the time rate of entropy production, in
Schematic and Given Data:
kJ/K
#
min.
kJ/K
#
min.
3
Mixed stream
Insulation
1
2
T
3
= 47°C
p
3
= 1 bar
T
2
= 127°C
p
2
= 1 bar
T
1
p
1
(AV)
1
= 32°C
= 1 bar
= 100 m
3
/min
Air
Oxygen
Figure E12.6
Assumptions:
1. The control volume identified by the dashed line on the accompanying figure operates at steady state.
2. No heat transfer occurs with the surroundings.
3. Kinetic and potential energy effects can be ignored, and
4. The entering gases can be regarded as ideal gases. The exiting mixture can be regarded as an ideal gas mixture.
5. The dry air is treated as a pure component.
Analysis:
(a) The mass flow rate of the dry air entering the control volume can be determined from the given volumetric flow rate (AV)
1
where v
a1
is the specific volume of the air at 1. Using the ideal gas equation of state
v
a1
1R
M
a
2T
1
p
1
a
8314
28.97
N
#
m
kg
#
K
b
1305 K2
10
5
N/m
2
0.875
m
3
kg
m
#
a1
1AV2
1
v
a1
W
#
cv
0.
578 Chapter 12 Ideal Gas Mixture and Psychrometric Applications
The mass flow rate of the dry air is then
The mass flow rate of the oxygen can be determined using mass and energy rate balances. At steady state, the amounts of
dry air and oxygen contained within the control volume do not vary. Thus, for each component individually it is necessary
for the incoming and outgoing mass flow rates to be equal. That is
Using assumptions 1–3 together with the foregoing mass flow rate relations, the energy rate balance reduces to
where and denote the mass flow rates of the dry air and oxygen, respectively. The enthalpy of the mixture at the exit
is evaluated by summing the contributions of the air and oxygen, each at the mixture temperature. Solving for
The specific enthalpies can be obtained from Tables A-22 and A-23. Since Table A-23 gives enthalpy values on a molar
basis, the molecular weight of oxygen is introduced into the denominator to convert the molar enthalpy values to a mass
basis
(b) To obtain the mole fractions of the dry air and oxygen in the exiting mixture, first convert the mass flow rates to molar
flow rates using the respective molecular weights
where denotes molar flow rate. The molar flow rate of the mixture is the sum
The mole fractions of the air and oxygen are, respectively
(c) For the control volume at steady state, the entropy rate balance reduces to
The specific entropy of each component in the exiting ideal gas mixture is evaluated at its partial pressure in the mixture and
at the mixture temperature. Solving for
s
#
m
#
a
3s
a
1T
3
, y
a
p
3
2 s
a
1T
1
, p
1
24 m
#
o
3s
o
1T
3
, y
o
p
3
2 s
o
1T
2
, p
2
24
s
#
0 m
#
a
s
a
1T
1
, p
1
2 m
#
o
s
o
1T
2
, p
2
2 3m
#
a
s
a
1T
3
, y
a
p
3
2 m
#
o
s
o
1T
3
, y
o
p
3
24 s
#
y
a
n
#
a
n
#
3.95
4.67
0.846
and
y
o
n
#
o
n
#
0.72
4.67
0.154
n
#
n
#
a
n
#
o
3.95 0.72 4.67 kmol/min
n
#
n
#
n
#
o
m
#
o
M
o
23.1
kg/min
32 kg/kmol
0.72 kmol/min
n
#
a
m
#
a
M
a
114.29
kg/min
28.97 kg/kmol
3.95
kmol/min
23.1
kg
min
m
#
o
1114.29
kg/min21320.29 kJ/kg 305.22 kJ/kg2
a
1
32 kg/kmol
b
111,711 kJ/kmol 9,325 kJ/kmol2
m
#
o
m
#
a
c
h
a
1T
3
2 h
a
1T
1
2
h
o
1T
2
2 h
o
1T
3
2
d
m
#
o
m
#
o
m
#
a
0 m
#
a
h
a
1T
1
2 m
#
o
h
o
1T
2
2 3m
#
a
h
a
1T
3
2 m
#
o
h
o
1T
3
24
m
#
o2
m
#
o3
1oxygen2
m
#
a1
m
#
a3
1dry air2
m
#
a1
100
m
3
/min
0.875 m
3
/kg
114.29
kg
min
❶

12.5 Introducing Psychrometric Principles 579
Since p
1
p
3
, the specific entropy change of the dry air is
The terms are evaluated from Table A-22. Similarly, since p
2
p
3
, the specific entropy change of the oxygen is
The terms are evaluated from Table A-23. Note the use of the molecular weights M
a
and M
o
in the last two equations to
obtain the respective entropy changes on a mass basis.
The expression for the rate of entropy production becomes
Substituting values
This calculation is based on dry air modeled as a pure component (assumption 5). However, since O
2
is a component of
dry air (Table 12.1), the actual mole fraction of O
2
in the exiting mixture is greater than given here.
Entropy is produced when different gases, initially at different temperatures, are allowed to mix.
17.42
kJ
K
#
min
a
23.1
kg/min
32 kg/kmol
b
c207.112
kJ
kmol
#
K
213.765
kJ
kmol
#
K
a8.314
kJ
kmol
#
K
b ln 0.154 d
s
#
a114.29
kg
min
b c1.7669
kJ
kg
#
K
1.71865
kJ
kg
#
K
a
8.314
28.97
kJ
kg
#
K
b ln 0.846 d
s
#
m
#
a
cs°
a
1T
3
2 s°
a
1T
1
2
R
M
a
ln y
a
d
m
#
o
M
o
3s°
o
1T
3
2 s°
o
1T
2
2 R ln y
o
4
s
°
o
s
o
1T
3
, y
o
p
3
2 s
o
1T
2
, p
2
2
1
M
o
3s °
o
1T
3
2 s °
o
1T
2
2 R ln y
o
4
s°
a
s°
a
1T
3
2 s°
a
1T
1
2
R
M
a
ln y
a
s
a
1T
3
, y
a
p
3
2 s
a
1T
1
, p
1
2 s°
a
1T
3
2 s°
a
1T
1
2
R
M
a
ln
y
a
p
3
p
1
❶
❷
❷
PSYCHROMETRIC APPLICATIONS
The remainder of this chapter is concerned with the study of systems involving mixtures of
dry air and water vapor. A condensed water phase also may be present. Knowledge of the
behavior of such systems is essential for the analysis and design of air-conditioning devices,
cooling towers, and industrial processes requiring close control of the vapor content in air.
The study of systems involving dry air and water is known as psychrometrics.
12.5 Introducing Psychrometric Principles
The object of the present section is to introduce some important definitions and principles
used in the study of systems involving of dry air and water.
12.5.1 Moist Air
The term moist air refers to a mixture of dry air and water vapor in which the dry air is
treated as if it were a pure component. As can be verified by reference to appropriate property
psychrometrics
moist air
580 Chapter 12 Ideal Gas Mixture and Psychrometric Applications
data, the overall mixture and each mixture component behave as ideal gases at the states un-
der present consideration. Accordingly, for the applications to be considered, the ideal gas
mixture concepts introduced previously apply directly.
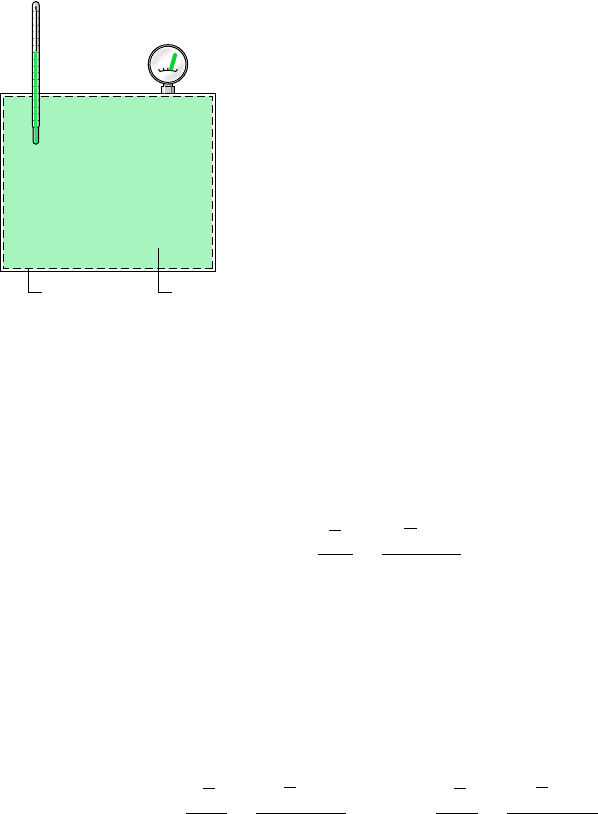
Shown in Fig. 12.3 is a closed system consisting of moist air occupying a volume V at
mixture pressure p and mixture temperature T. The overall mixture is assumed to obey the
ideal gas equation of state. Thus
(12.40)
where n, m, and M denote the moles, mass, and molecular weight of the mixture,
respectively, and n mM. Each mixture component is considered to act as if it existed
alone in the volume V at the mixture temperature T while exerting a part of the pressure.
The mixture pressure is the sum of the partial pressures of the dry air and the water vapor:
p p
a
p
v
.
Using the ideal gas equation of state, the partial pressures p
a
and p
v
of the dry air and
water vapor are, respectively
(12.41a)
where n
a
and n
v
denote the moles of dry air and water vapor, respectively; m
a
, m
v
, M
a
, and
M
v
are the respective masses and molecular weights. The amount of water vapor present is
normally much less than the amount of dry air. Accordingly, the values of n
v
, m
v
, and p
v
are
small relative to the corresponding values of n
a
, m
a
, and p
a
.
Forming ratios with Eqs. 12.40 and 12.41a, we get the following alternative expressions
for p
a
and p
v
(12.41b)
where y
a
and y
v
are the mole fractions of the dry air and water vapor, respectively.
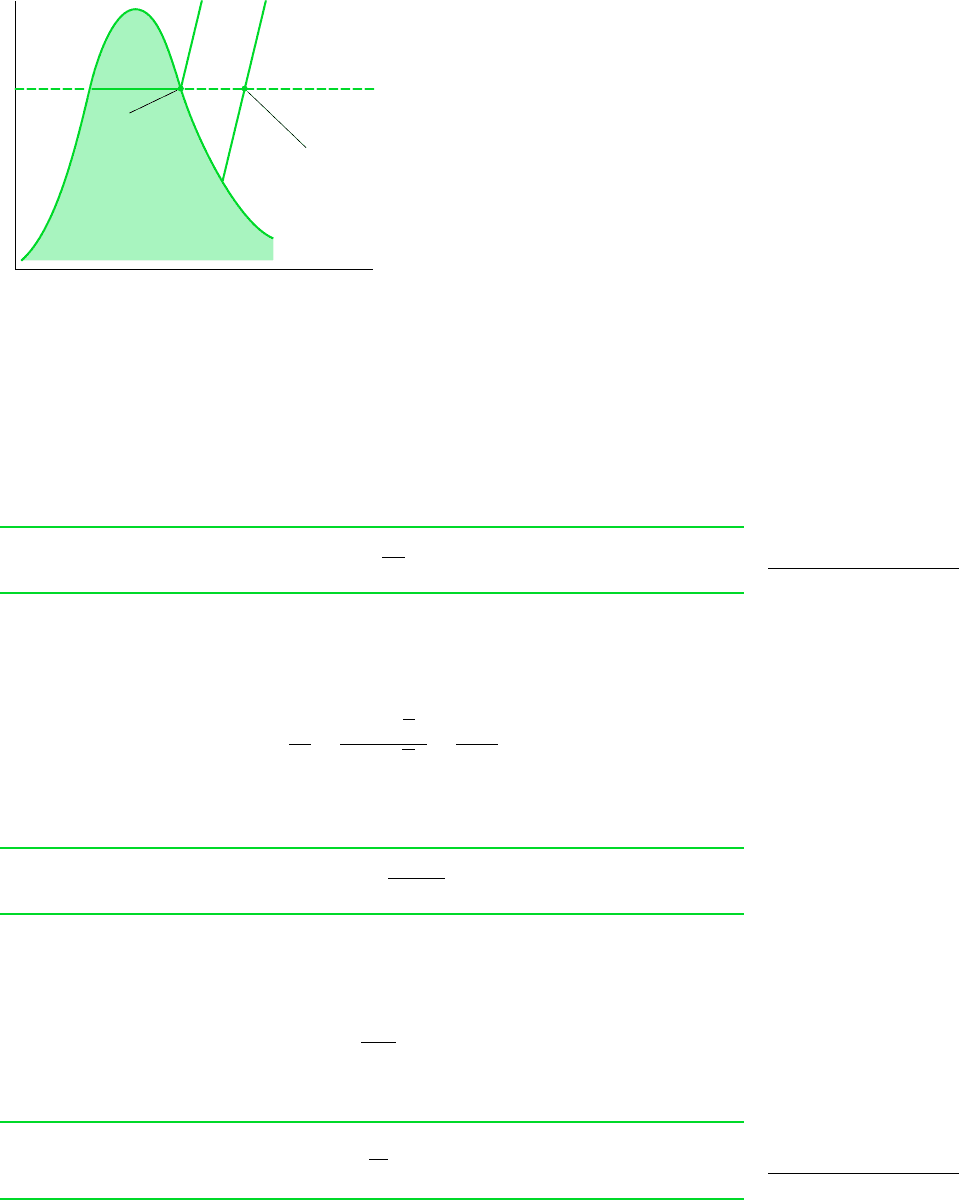
A typical state of water vapor in moist air is shown in Fig. 12.4. At this state, fixed by
the partial pressure p
v
and the mixture temperature T, the vapor is superheated. When the
partial pressure of the water vapor corresponds to the saturation pressure of water at the mix-
ture temperature, p
g
of Fig. 12.4, the mixture is said to be saturated. Saturated air is a mixture
of dry air and saturated water vapor. The amount of water vapor in moist air varies from zero
in dry air to a maximum, depending on the pressure and temperature, when the mixture is
saturated.
p
a
y
a
p,
p
v
y
v
p
p
a
n
a
RT
V
m
a
1R
M
a
2T
V
,
p
v
n
v
RT
V
m
v
1R
M
v
2T
V
p
nR
T
V
m1R
M2T
V
Volume = V
dry air
water vapor
mixture
n
a
, m
a
:
n
v
, m
v
:
n, m:
Pressure = p
Temperature = T
Boundary
Figure 12.3 Mixture of dry air and water vapor.
saturated air
12.5 Introducing Psychrometric Principles 581
12.5.2 Humidity Ratio, Relative Humidity, and
Mixture Enthalpy
A given moist air sample can be described in a number of ways. The mixture can be described
in terms of the moles of dry air and water vapor present or in terms of the respective mole
fractions. Alternatively, the mass of dry air and water vapor, or the respective mass fractions,
can be specified. The composition also can be indicated by means of the humidity ratio ,
defined as the ratio of the mass of the water vapor to the mass of dry air
(12.42)
The humidity ratio is sometimes referred to as the specific humidity.
The humidity ratio can be expressed in terms of partial pressures and molecular weights
by solving Eqs. 12.41a for m
a
and m
v
, respectively, and substituting the resulting expressions
into Eq. 12.42 to obtain
Introducing p
a
p p
v
and noting that the ratio of the molecular weight of water to that
of dry air is approximately 0.622, this expression can be written as
(12.43)
Moist air also can be described in terms of the relative humidity , defined as the ratio
of the mole fraction of water vapor y
v
in a given moist air sample to the mole fraction y
v,sat
in a saturated moist air sample at the same mixture temperature and pressure
Since p
v
y
v
p and p
g
y
v,sat
p, the relative humidity can be expressed as
(12.44)
The pressures in this expression for the relative humidity are labeled on Fig. 12.4.
f
p
v
p
g
b
T, p
f
y
v
y
v,sat
b
T, p
v 0.622
p
v
p p
v
v
m
v
m
a
M
v
p
v
V
RT
M
a
p
a
V
RT
M
v
p
v
M
a
p
a
v
m
v
m
a
T
v
p
g
p
v
Mixture
temperature
Typical state of the
water vapor in moist air
State of the
water vapor in a
saturated mixture
Figure 12.4 T–v diagram for water
vapor in an air–water mixture.
humidity ratio
relative humidity
582 Chapter 12 Ideal Gas Mixture and Psychrometric Applications
The humidity ratio and relative humidity can be measured. For laboratory measurements
of humidity ratio, a hygrometer can be used in which a moist air sample is exposed to suit-
able chemicals until the moisture present is absorbed. The amount of water vapor is deter-
mined by weighing the chemicals. Continuous recording of the relative humidity can be
accomplished by means of transducers consisting of resistance- or capacitance-type sensors
whose electrical characteristics change with relative humidity.
EVALUATING H, U, AND S. The values of H, U, and S for moist air modeled as an ideal
gas mixture can be found by adding the contribution of each component at the condition at
which the component exists in the mixture. For example, the enthalpy H of a given moist air
sample is
(12.45)
Dividing by m
a
and introducing the humidity ratio gives the mixture enthalpy per unit mass
of dry air
(12.46)
The enthalpies of the dry air and water vapor appearing in Eq. 12.46 are evaluated at the
mixture temperature. An approach similar to that for enthalpy also applies to the evaluation
of the internal energy of moist air.
Reference to steam table data or a Mollier diagram for water shows that the enthalpy of
superheated water vapor at low vapor pressures is very closely given by the saturated vapor
value corresponding to the given temperature. Hence, the enthalpy of the water vapor h
v
in
Eq. 12.46 can be taken as h
g
at the mixture temperature. That is
(12.47)
This approach is used in the remainder of the chapter. Enthalpy data for water vapor as an
ideal gas from Table A-23 are not used for h
v
because the enthalpy datum of the ideal gas
tables differs from that of the steam tables. These different datums can lead to error when
studying systems that contain both water vapor and a liquid or solid phase of water. The
enthalpy of dry air, h
a
, can be obtained from Table A-22, however, because air is a
gas at all states under present consideration and is closely modeled as an ideal gas at
these states.
When evaluating the entropy of moist air, the contribution of each component is deter-
mined at the mixture temperature and the partial pressure of the component in the mixture.
Using Eq. 6.19, it can be shown that the specific entropy of the water vapor is given by
s
v
(T, p
v
) s
g
(T ) R ln , where s
g
is the specific entropy of saturated vapor at temperature
T from the steam tables and is the relative humidity.
USING COMPUTER SOFTWARE. Property functions for moist air are listed under the
Properties menu of Interactive Thermodynamics: IT. Functions are included for humidity ra-
tio, relative humidity, specific enthalpy and entropy as well as other psychrometric properties
introduced later. The methods used for evaluating these functions correspond to the methods
discussed in this chapter, and the values returned by the computer software agree closely with
those obtained by hand calculations with table data. The use of IT for psychrometric evaluations
is illustrated in examples later in the chapter.
h
v
h
g
1T 2
H
m
a
h
a
m
v
m
a
h
v
h
a
vh
v
H H
a
H
v
m
a
h
a
m
v
h
v
Temperature
Sensing element
Relative
humidity
mixture enthalpy
12.5 Introducing Psychrometric Principles 583
12.5.3 Modeling Moist Air in Equilibrium with Liquid Water
Thus far, our study of psychrometrics has been conducted as an application of the ideal gas
mixture principles introduced in the first part of this chapter. However, many systems of in-
terest are composed of a mixture of dry air and water vapor in contact with a liquid (or solid)
water phase. To study these systems requires additional considerations.
Shown in Fig. 12.5 is a vessel containing liquid water, above which is a mixture of water
vapor and dry air. If no interactions with the surroundings are allowed, liquid will evaporate
until eventually the gas phase becomes saturated and the system attains an equilibrium state.
For many engineering applications, systems consisting of moist air in equilibrium with a
liquid water phase can be described simply and accurately with the following idealizations:
The dry air and water vapor behave as independent ideal gases.
The equilibrium between the liquid phase and the water vapor is not significantly
disturbed by the presence of the air.
The partial pressure of the water vapor equals the saturation pressure of water
corresponding to the temperature of the mixture: p
v
p
g
(T ).
Similar considerations apply for systems consisting of moist air in equilibrium with a solid
water phase. The presence of the air actually alters the partial pressure of the vapor from the
saturation pressure by a small amount whose magnitude is calculated in Sec. 14.6.
12.5.4 Evaluating the Dew Point Temperature
A significant aspect of the behavior of moist air is that partial condensation of the water va-
por can occur when the temperature is reduced. This type of phenomenon is commonly en-
countered in the condensation of vapor on windowpanes and on pipes carrying cold water.
The formation of dew on grass is another familiar example.
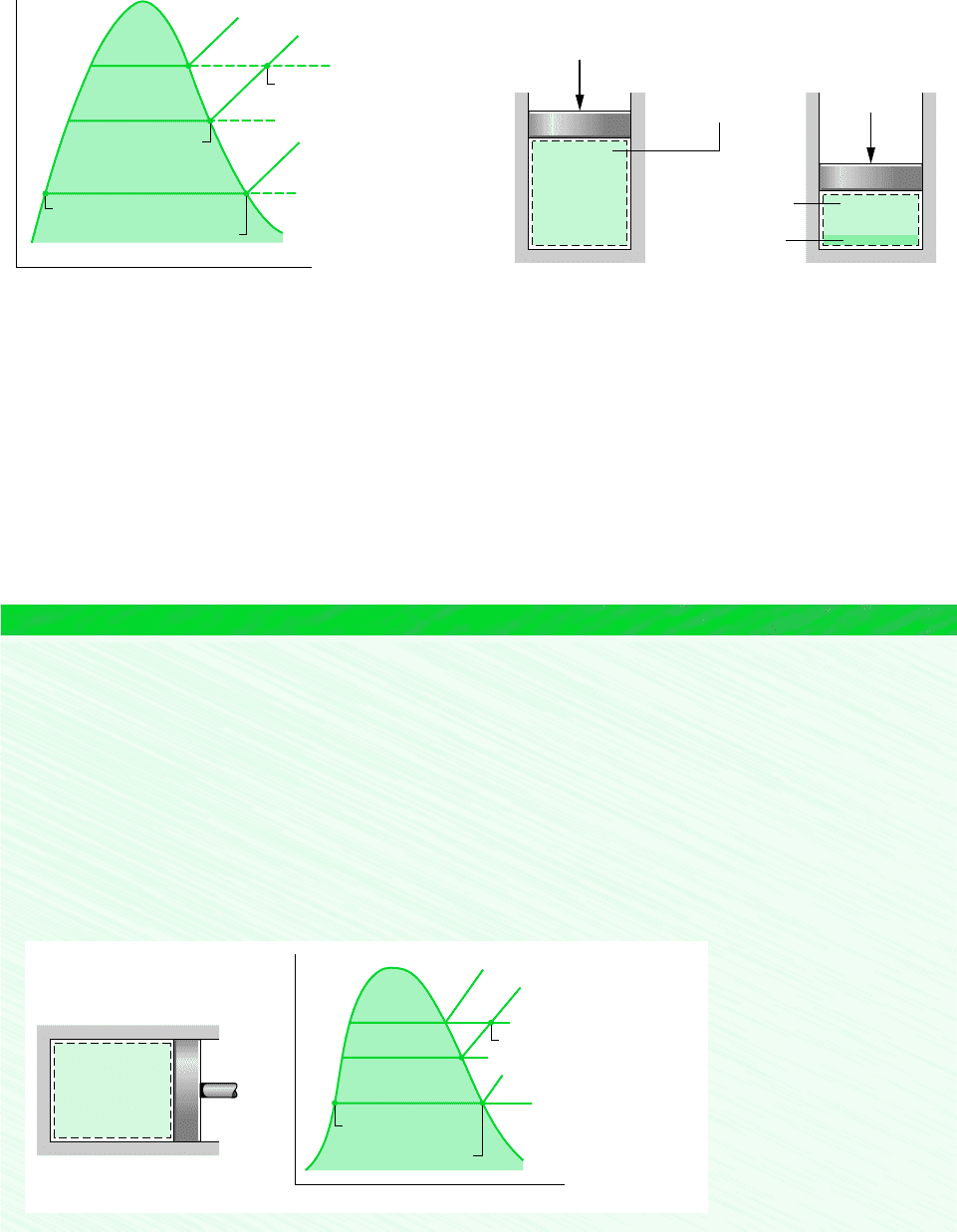
To study such condensation, consider a closed system consisting of a sample of moist air
that is cooled at constant pressure, as shown in Fig. 12.6. The property diagram given on
this figure locates states of the water vapor. Initially, the water vapor is superheated at state 1.
In the first part of the cooling process, both the system pressure and the composition of the
moist air would remain constant. Accordingly, since p
v
y
v
p, the partial pressure of the wa-
ter vapor would remain constant, and the water vapor would cool at constant p
v
from state 1
to state d, called the dew point. The saturation temperature corresponding to p
v
is called the
dew point temperature. This temperature is labeled on Fig. 12.6.
In the next part of the cooling process, the system would be cooled below the dew point
temperature and some of the water vapor initially present would condense. At the final state,
the system would consist of a gas phase of dry air and water vapor in equilibrium with a liq-
uid water phase. The vapor that remains can be regarded as saturated at the final temperature,
System boundary
Liquid water
Gas phase: Dry air and
water vapor
Figure 12.5 System consisting of moist air in contact
with liquid water.
dew point temperature
584 Chapter 12 Ideal Gas Mixture and Psychrometric Applications
state 2 of Fig. 12.6, with a partial pressure equal to the saturation pressure p
g2
corresponding
to this temperature. The condensate would be a saturated liquid at the final temperature, state 3
of Fig. 12.6. Note that the partial pressure of the water vapor at the final state, p
g2
, is less than
the initial value, p
v1
. Owing to condensation, the partial pressure decreases because the amount
of water vapor present at the final state is less than at the initial state. Since the amount of
dry air is unchanged, the mole fraction of water vapor in the moist air also decreases.
In the next two examples, we illustrate the use of psychrometric properties introduced
thus far. The examples consider, respectively, cooling moist air at constant pressure and at
constant volume.
T
v
p
g1
p
v1
< p
g1
p
g2
< p
v1
p
p
Initial temperature
Final temperature
Dew point temperature
Initial state
of the water vapor
Dew point
1
d
3
2
Condensate
Final state
of the water vapor
Dry air and
superheated vapor
at the initial temper-
ature
Air and saturated vapor
at final temperature
Condensate:
saturated liquid
at final temperature
Final
state
Initial
state
Figure 12.6 States of water for moist air cooled at constant mixture pressure.
EXAMPLE 12.7 Cooling Moist Air at Constant Pressure
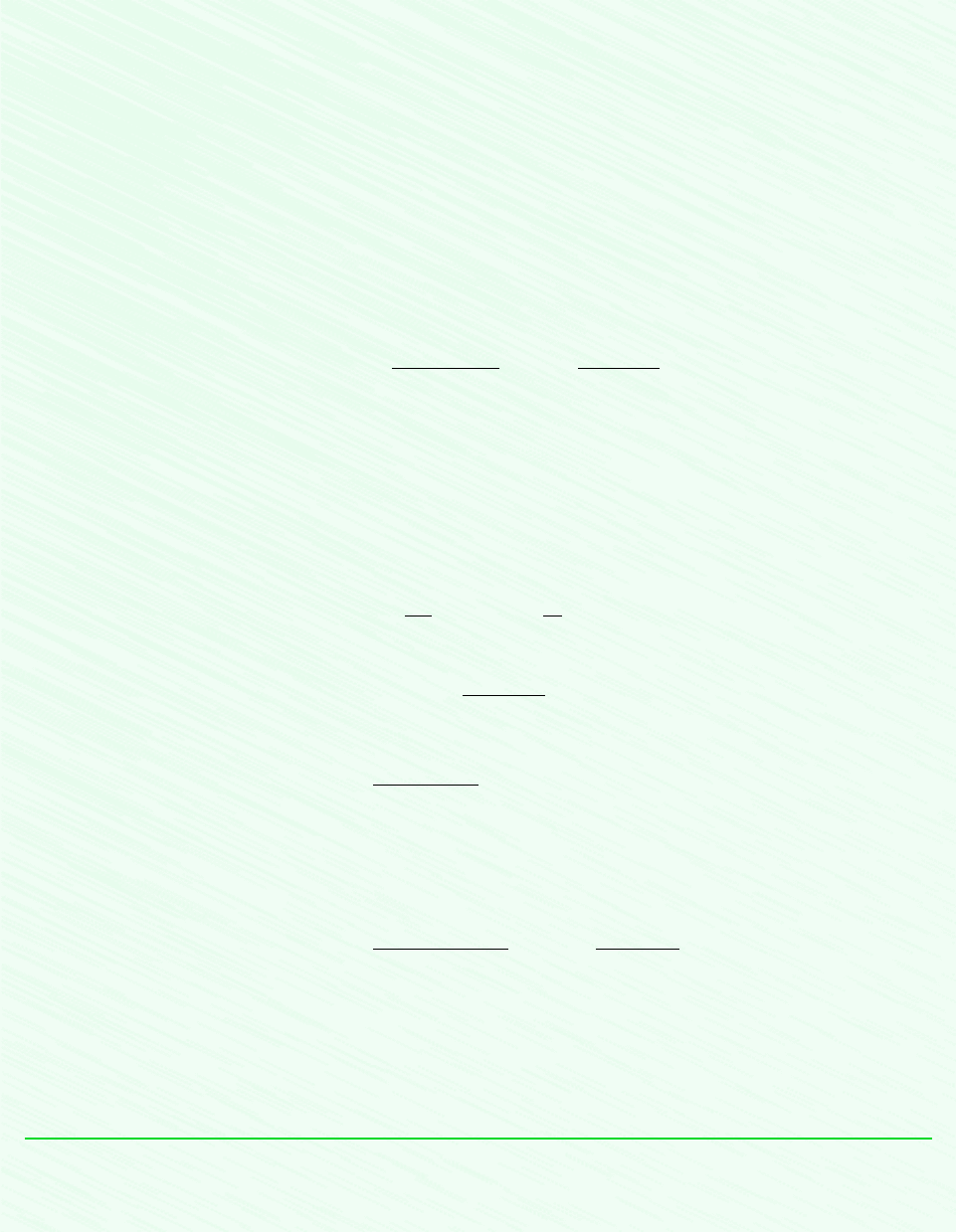
A 1 kg sample of moist air initially at 21C, 1 bar, and 70% relative humidity is cooled to 5C while keeping the pressure
constant. Determine (a) the initial humidity ratio, (b) the dew point temperature, in C, and (c) the amount of water vapor that
condenses, in kg.
SOLUTION
Known: A 1 kg sample of moist air is cooled at a constant mixture pressure of 1 bar from 21 to 5C. The initial relative
humidity is 70%.
Find: Determine the initial humidity ratio, the dew point temperature, in C, and the amount of water vapor that condenses,
in kg.
Schematic and Given Data:
T
v
p
g1
= 0.02487 bar
p
g2
= .00872 bar
p
v1
= 0.01741 bar
Dewpoint temperature = 15.3C
21C
5C
Initial state of vapor
Condensate
Final state
of vapor
m
T
1
1
T
2
= 1 kg
= 21C
= 70%
= 5C
φ
Figure E12.7
12.5 Introducing Psychrometric Principles 585
Assumptions:
1. The 1-kg sample of moist air is taken as the closed system. The system pressure remains constant at 1 bar.
2. The gas phase can be treated as an ideal gas mixture. Each mixture component acts as an ideal gas existing alone in the
volume occupied by the gas phase at the mixture temperature.
3. When a liquid water phase is present, the water vapor exists as a saturated vapor at the system temperature. The liquid
present is a saturated liquid at the system temperature.
Analysis:
(a) The initial humidity ratio can be evaluated from Eq. 12.43. This requires the partial pressure of the water vapor, p
v1
, which
can be found from the given relative humidity and p
g
from Table A-2 at 70F as follows
Inserting values in Eq. 12.43
(b) The dew point temperature is the saturation temperature corresponding to the partial pressure, p
v1
. Interpolation in
Table A-2 gives T 15.3C. The dew point temperature is labeled on the accompanying property diagram.
(c) The amount of condensate, m
w
, equals the difference between the initial amount of water vapor in the sample, m
v1
, and
the final amount of water vapor, m
v2
. That is
To evaluate m
v1
, note that the system initially consists of 1 lb of dry air and water vapor, so 1 lb m
a
m
v1
, where m
a
is the mass of dry air present in the sample. Since
1
m
v1
m
a
, m
a
m
v1
1
. With this we get
Solving for m
v1
Inserting the value of
1
determined in part (a)
The mass of dry air present is then m
a
1 0.0109 0.9891 kg (dry air).
Next, let us evaluate m
v2
. With assumption 3, the partial pressure of the water vapor remaining in the system at the final
state is the saturation pressure corresponding to 5C: p
g
0.00872 bar. Accordingly, the humidity ratio after cooling is found
from Eq. 12.43 as
The mass of the water vapor present at the final state is then
Finally, the amount of water vapor that condenses is
The amount of water vapor present in a typical moist air mixture is considerably less than the amount of dry air present.
At the final state, the quality of the two-phase liquid–vapor mixture of water is x 0.00530.0109 0.47 (47%). The
relative humidity of the gas phase is 100%.
m
w
m
v1
m
v2
0.0109 0.0053 0.0056 kg 1condensate2
m
v2
v
2
m
a
10.00542 10.98912 0.0053 kg 1vapor2
v
2
0.622 a
.00872
1.01325 .00872
b 0.0054
kg 1vapor2
kg 1dry air2
m
v1
1
kg
11
0.0112 1
0.0109 kg 1vapor2
m
v1
1
kg
11
v
1
2 1
1
kg
m
v1
v
1
m
v1
m
v1
a
1
v
1
1b
m
w
m
v1
m
v2
v
1
0.622 a
0.2542
14.7 0.2542
b 0.011
kg 1vapor2
kg 1dry air2
p
v1
fp
g
10.72 10.02487 bar2 0.01741 bar
❶
❷
❶
❷
