Jost J. Partial Differential Equations
Подождите немного. Документ загружается.


6.3 The Energy Inequality and the Relation with the Heat Equation 147
Since the points (y
1
,y
2
,y
3
)and(y
1
,y
2
, −y
3
) yield the same contributions,
we obtain
u(x
1
,x
2
,t)=
1
2π
B(x,t)
g(y)
"
t
2
−|x − y|
2
dy
+
∂
∂t
⎛
⎝
1
2π
B(x,t)
f(y)
"
t
2
−|x − y|
2
dy
⎞
⎠
,
where x =(x
1
,x
2
), y =(y
1
,y
2
), and the ball B(x, t) now is the two-
dimensional one.
Thevaluesofu at (x, t) now depend on the values on the whole disk
B(x, t) and not only on its boundary ∂B(x, t).
A reference for Sections 6.1 and 6.2 is F. John [10].
6.3 The Energy Inequality and the Relation with the
Heat Equation
Let u be a solution of the wave equation
u
tt
(x, t) − Δu(x, t)=0 forx ∈ R
d
,t>0. (6.3.1)
We define the energy norm of u as follows:
E(t):=
1
2
R
d
u
t
(x, t)
2
+
d
i=1
u
x
i
(x, t)
2
dx. (6.3.2)
We have
dE
dt
=
R
d
u
t
u
tt
+
d
i=1
u
x
i
u
x
i
t
dx
=
R
d
u
t
(u
tt
− Δu)+
d
i=1
(u
t
u
x
i
)
x
i
dx
=0
(6.3.3)
if u(x, t) = 0 for sufficiently large |x| (where that may depend on t, so that this
computation may be applied to solutions of (6.3.1) with compactly supported
initial values).
In this manner, it is easy to show the following result about the region of
dependency of a solution of (6.3.1), partially generalizing the corresponding
results of Section 6.2 to arbitrary dimensions:
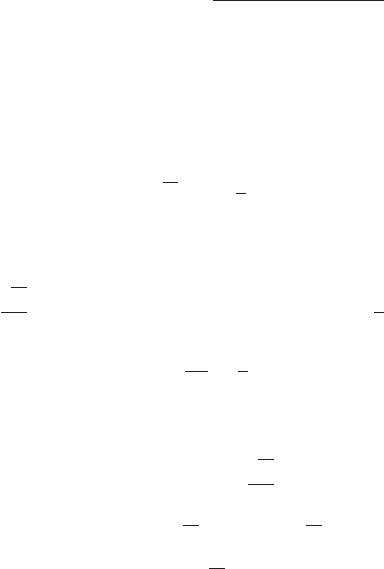
148 6. The Wave Equation
Theorem 6.3.1: Let u be a solution of (6.3.1) with
u(x, 0) = f(x),u
t
(x, 0) = 0, (6.3.4)
and let K := supp f
:=
{x ∈ R
d
: f(x) =0}
be compact. Then
u(x, t)=0 for dist(x, K) >t. (6.3.5)
Proof: We show that f(y) = 0 for all y ∈ B(x, T ) implies u(x, T ) ≥ 0, which
is equivalent to our assertion. We put
E(t):=
1
2
B(x,T −t)
u
2
t
+
d
i=1
u
2
y
i
dy (6.3.6)
and obtain as in (6.3.3) (cf. (1.1.1))
d
E
dt
=
B(x,T −t)
u
t
u
tt
+
u
y
i
u
y
i
t
dy −
1
2
∂B(x,T −t)
u
2
t
+
u
2
y
i
do(y)
=
∂B(x,T −t)
u
t
∂u
∂ν
−
1
2
u
2
t
+
u
2
y
i
do(y).
By the Schwarz inequality, the integrand is nonpositive, and we conclude that
d
E
dt
≤ 0fort>0.
Since by assumption
E(0) = 0 and E is nonnegative, necessarily
E(t) = 0 for all t ≤ T,
and hence
u(y, t)=0 for |x − y|≤T − t,
so that
u(x, T )=0
as desired.
Theorem 6.3.2: As in Theorem 6.3.1, let u be a solution of the wave equa-
tion with initial values
u(x, 0) = f(x) with compact support
and
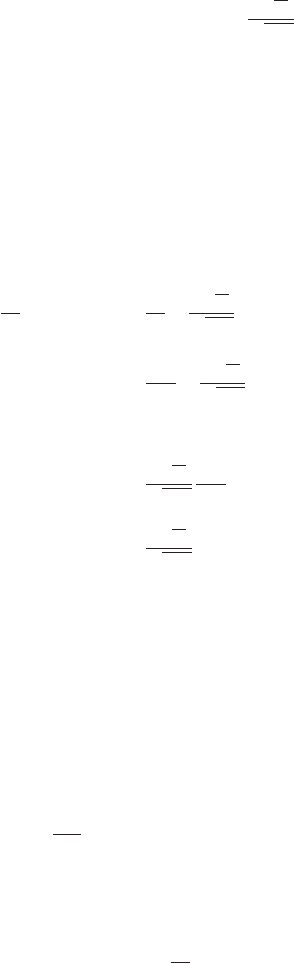
6.3 The Energy Inequality and the Relation with the Heat Equation 149
u
t
(x, 0) = 0.
Then
v(x, t):=
∞
−∞
e
−
s
2
4t
√
4πt
u(x, s)ds
yields a solution of the heat equation
v
t
(x, t) − Δv(x, t)=0 for x ∈ R
d
,t>0
with initial values
v(x, 0) = f (x).
Proof: That u solves the heat equation is seen by differentiating under the
integral
∂
∂t
v(x, t)=
∞
−∞
∂
∂t
e
−
s
2
4t
√
4πt
u(x, s)ds
=
∞
−∞
∂
2
∂s
2
e
−
s
2
4t
√
4πt
u(x, s)ds
(since the kernel solves the heat equation)
=
∞
−∞
e
−
s
2
4t
√
4πt
∂
2
∂s
2
u(x, s)ds
=
∞
−∞
e
−
s
2
4t
√
4πt
Δ
x
u(x, s)ds
(since u solves the wave equation)
= Δv(x, t),
where we omit the detailed justification of interchanging differentiation and
integration here. Then v(x, 0) = u(x, 0) = f(x) follows as in Section 4.1.
Summary
In the present chapter we have studied the wave equation
∂
2
∂t
2
u(x, t) − Δu(x, t)=0 forx ∈ R
d
,t>0
with initial data
u(x, 0) = f(x),
∂
∂t
u(x, 0) = g(x).
150 6. The Wave Equation
In contrast to the heat equation, there is no gain of regularity compared
to the initial data, and in fact, for d>1, there may even occur a loss of
regularity.
As was the case with the Laplace equation, mean value constructions are
important for the wave equation, and they permit us to reduce the wave
equation for d>1 to the Darboux equation for the mean values, which is
hyperbolic as well but involves only one spatial coordinate.
The propagation speed for the wave equation is finite, in contrast to the
heat equation. The effect of perturbations sets in sharply, and in odd dimen-
sions greater than 1, it also terminates sharply (Huygens principle).
The energy
E(t)=
R
d
|u
t
(x, t)|
2
+ |∇
x
u(x, t)|
2
dx
is constant in time.
By a certain time averaging, a solution of the wave equation yields a
solution of the heat equation.
Exercises
6.1 We consider the wave equation in one space dimension,
u
tt
− u
xx
=0 for0<x<π,t>0,
with initial data
u(x, 0) =
∞
n=1
α
n
sin nx, u
t
(x, 0) =
∞
n=1
β
n
sin nx
and boundary values
u(0,t)=u(π,t) = 0 for all t>0.
Represent the solution as a Fourier series
u(x, t)=
∞
n=1
γ
n
(t)sinnx
and compute the coefficients γ
n
(t).
6.2 Consider the equation
u
t
+ cu
x
=0
for some function u(x, t), x, t ∈ R,wherec is constant. Show that u is
constant along any line
Exercises 151
x − ct = const = ξ,
and thus the general solution of this equation is given as
u(x, t)=f (ξ)=f(x − ct)
where the initial values are u(x, 0) = f(x). Does this differential equation
satisfy the Huygens principle?
6.3 We consider the general quasilinear PDE for a function u(x, y)oftwo
variables,
au
xx
+2bu
xy
+ cu
yy
= d,
where a, b, c, d are allowed to depend on x, y, u, u
x
,andu
y
. We consider
the curve γ(s)=(ϕ(s),ψ(s)) in the xy-plane, where we wish to prescribe
the function u and its first derivatives:
u = f (s),u
x
= g(s),u
y
= h(s)forx = ϕ(s),y = ψ(s).
Show that for this to be possible, we need the relation
f
(s)=g(s)ϕ
(s)+h(s)ψ
(s).
For the values of u
xx
,u
xy
,u
yy
along γ, compute the equations
ϕ
u
xx
+ ψ
u
xy
= g
,
ϕ
u
xy
+ ψ
u
yy
= h
.
Conclude that the values of u
xx
,u
xy
,andu
yy
along γ are uniquely de-
termined by the differential equations and the data f,g,h (satisfying the
above compatibility conditions), unless
aψ
2
− 2bϕ
ψ
+ cϕ
2
=0
along γ. If this latter equation holds, γ is called a characteristic curve for
the solution u of our PDE au
xx
+2bu
xy
+ cu
yy
= d. (Since a, b, c, d may
depend on u and u
x
,u
y
, in general it depends not only on the equation,
but also on the solution, which curves are characteristic.) How is this
existence of characteristic curves related to the classification into elliptic,
hyperbolic, and parabolic PDEs discussed in the introduction? What are
the characteristic curves of the wave equation u
tt
− u
xx
=0?
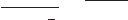
7. The Heat Equation, Semigroups, and
Brownian Motion
7.1 Semigroups
We first want to reinterpret some of our results about the heat equation. For
that purpose, we again consider the heat kernel of R
d
, which we now denote
by p(x, y, t),
p(x, y, t)=
1
(4πt)
d
2
e
−
|x−y|
2
4t
. (7.1.1)
For a continuous and bounded function f : R
d
→ R, by Lemma 4.2.1
u(x, t)=
R
d
p(x, y, t)f(y)dy (7.1.2)
then solves the heat equation
Δu(x, t) − u
t
(x, t)=0. (7.1.3)
For t>0, and letting C
0
b
denote the class of bounded continuous functions,
we define the operator
P
t
: C
0
b
(R
d
) → C
0
b
(R
d
)
via
(P
t
f)(x)=u(x, t), (7.1.4)
with u from (7.1.2). By Lemma 4.2.2
P
0
f := lim
t→0
P
t
f = f; (7.1.5)
i.e., P
0
is the identity operator. The crucial point is that we have for any
t
1
,t
2
≥ 0,
P
t
1
+t
2
= P
t
2
◦ P
t
1
. (7.1.6)
Written out, this means that for all f ∈ C
0
b
(R
d
),
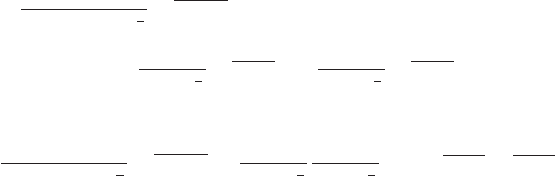
154 7. The Heat Equation, Semigroups, and Brownian Motion
R
d
1
(4π (t
1
+ t
2
))
d
2
e
−
|x−y|
2
4(t
1
+t
2
)
f(y) dy
=
R
d
1
(4πt
2
)
d
2
e
−
|x−z|
2
4t
2
R
d
1
(4πt
1
)
d
2
e
−
|z−y|
2
4t
1
f(y) dy dz. (7.1.7)
This follows from the formula
1
(4π (t
1
+ t
2
))
d
2
e
−
|x−y|
2
4(t
1
+t
2
)
=
1
(4πt
2
)
d
2
1
(4πt
1
)
d
2
R
d
e
−
|x−z|
2
4t
2
e
−
|z−y|
2
4t
1
dz,
(7.1.8)
which can be verified by direct computation (cf. also Exercise 4.3).
There exists, however, a deeper and more abstract reason for (7.1.6):
P
t
1
+t
2
f(x) is the solution at time t
1
+ t
2
of the heat equation with initial
values f.Attimet
1
, this solution has the value P
t
1
f(x). On the other hand,
P
t
2
(P
t
1
f)(x) is the solution at time t
2
of the heat equation with initial values
P
t
1
f. Since by Theorem 4.1.2, the solution of the heat equation is unique
within the class of bounded functions, and the heat equation is invariant
under time translations, it must lead to the same result starting at time 0
with initial values P
t
1
f and considering the solution at time t
2
, or starting
at time t
1
with value P
t
1
f and considering the solution at time t
1
+ t
2
,since
the time difference is the same in both cases. This reasoning is also valid
for the initial value problem because solutions here are unique as well, by
Corollary 4.1.1. We have the following result:
Theorem 7.1.1: Let Ω ⊂ R
d
be bounded and of class C
2
,andletg : ∂Ω →
R be continuous. For any f ∈ C
0
b
(Ω),welet
P
Ω,g,t
f(x)
be the solution of the initial value problem
Δu − u
t
=0 in Ω × (0, ∞),
u(x, t)=g(x) for x ∈ ∂Ω, (7.1.9)
u(x, 0) = f(x) for x ∈ Ω.
We then have
P
Ω,g,0
f = lim
t0
P
Ω,g,t
f = f for all f ∈ C
0
(Ω), (7.1.10)
P
Ω,g,t
1
+t
2
= P
Ω,g,t
2
◦ P
Ω,g,t
1
. (7.1.11)
Corollary 7.1.1: Under the assumptions of Theorem 7.1.1, we have for all
t
0
≥ 0 and for all f ∈ C
0
b
(Ω),
P
Ω,g,t
0
f = lim
tt
0
P
Ω,g,t
f.

7.2 Infinitesimal Generators of Semigroups 155
We wish to cover the phenomenon just exhibited by a general definition:
Definition 7.1.1: Let B be a Banach space, and for t>0,letT
t
: B → B
be continuous linear operators with
(i) T
0
=Id;
(ii) T
t
1
+t
2
= T
t
2
◦ T
t
1
for all t
1
,t
2
≥ 0;
(iii) lim
t→t
0
T
t
v = T
t
0
v for all t
0
≥ 0 and all v ∈ B.
Then the family {T
t
}
t≥0
is called a continuous semigroup (of operators).
A different and simpler example of a semigroup is the following: Let B be
the Banach space of bounded, uniformly continuous functions on [0, ∞). For
t ≥ 0, we put
T
t
f(x):=f(x + t). (7.1.12)
Then all conditions of Definition 7.1.1 are satisfied. Both semigroups (for
the heat semigroup, this follows from the maximum principle) satisfy the
following definition:
Definition 7.1.2: A continuous semigroup {T
t
}
t≥0
of continuous linear op-
erators of a Banach space B with norm ·is called contracting if for all
v ∈ B and all t ≥ 0,
T
t
v≤v. (7.1.13)
(Here, continuity of the semigroup means continuous dependence of the op-
erators T
t
on t.)
7.2 Infinitesimal Generators of Semigroups
If the initial values f(x)=u(x, 0) of a solution u of the heat equation
u
t
(x, t) − Δu(x, t) = 0 (7.2.1)
are of class C
2
, we expect that
lim
t0
u(x, t) − u(x, 0)
t
= u
t
(x, 0) = Δu(x, 0) = Δf(x), (7.2.2)
or with the notation
u(x, t)=P
t
f(u)
of the previous section,
lim
t0
1
t
(P
t
− Id)f = Δf. (7.2.3)
We want to discuss this in more abstract terms and verify the following
definition:

156 7. The Heat Equation, Semigroups, and Brownian Motion
Definition 7.2.1: Let {T
t
}
t≥0
be a continuous semigroup on a Banach space
B.Weput
D(A):=
v ∈ B : lim
t0
1
t
(T
t
− Id)v exists
⊂ B (7.2.4)
and call the linear operator
A : D(A) → B,
defined as
Av := lim
t0
1
t
(T
t
− Id)v, (7.2.5)
the infinitesimal generator of the semigroup {T
t
}.
Then D(A) is nonempty, since it contains 0.
Lemma 7.2.1: For al l v ∈ D(A) and all t ≥ 0, we have
T
t
Av = AT
t
v. (7.2.6)
Thus A commutes with all the T
t
.
Proof: For v ∈ D(A), we have
T
t
Av = T
t
lim
τ0
1
τ
(T
τ
− Id)v
= lim
τ0
1
τ
(T
t
T
τ
− T
t
)v (since T
t
is continuous and linear)
= lim
τ0
1
τ
(T
τ
T
t
− T
t
)v (by the semigroup property)
= lim
τ0
1
τ
(T
τ
− Id)T
t
v
= AT
t
v.
In particular, if v ∈ D(A), then so is T
t
v. In that sense, there is no loss of
regularity of T
t
v when compared with v (= T
0
v).
In the sequel, we shall employ the notation
J
λ
v :=
∞
0
λe
−λs
T
s
vds for λ>0 (7.2.7)
for a contracting semigroup {T
t
}. The integral here is a Riemann integral
for functions with values in some Banach space. The standard definition of
the Riemann integral as a limit of step functions easily generalizes to the
