Hungerford T.W., Shaw D.J. Contemporary Precalculus: A Graphing Approach
Подождите немного. Документ загружается.


In Exercises 35–40, express the sum in notation.
35. 1 2 3 4 5 6 7 8 9 10 11
36. 1
1
2
2
3
3
4
4
5
5
37.
2
1
7
2
1
8
2
1
9
2
1
10
2
1
11
2
1
12
2
1
13
38. (6)
11
(6)
12
(6)
13
(6)
14
(6)
15
39. 2
2
2
2
2
3
3
2
4
4
2
5
5
2
6
6
2
7
7
40. 2
3
2
4
3
5
4
6
5
7
6
8
7
9
8
1
9
0
In Exercises 41–50, find the sum.
41.
7
k1
k 42.
5
k1
(k)
43.
4
i1
(i
2
1) 44.
10
i1
6
45.
5
i1
3i 46.
4
i1
2
1
i
47.
16
n1
(2n 3) 48.
75
n1
(1)
n
(3n 1)
49.
36
n15
(n
2
8) 50.
25
k0
(2k
2
5k 1)
In Exercises 51–54, find the third and the sixth partial sums of
the sequence.
51. {n
2
5n 2} 52. {(2n 3n
2
)
2
}
53. {(1)
n1
5} 54. {2
n
(2 n
2
)}
n0
In Exercises 55–58, express the given sum in S notation and
find the sum.
55.
1
3
1
5
1
7
1
9
1
1
1
1
1
3
56. 2 1
4
5
5
7
2
3
1
7
1
1
8
3
57.
1
8
2
9
1
3
0
1
4
1
1
5
2
58.
3
2
5
5
4
7
7
8
9
9
1
6
11
11
3
2
13
13
6
4
15
15
12
8
17
In Exercises 59–64, use a calculator to approximate the
required term or sum.
59. a
12
where a
n
1
1
n
n
60. a
50
where a
n
ln
n
2
n
61. a
102
where a
n
3
n
n
3
2
n
2
2
n
5n
1
62. a
125
where a
n
n
n
63.
14
k1
k
1
2
64.
22
n8
1
n
836 CHAPTER 12 Discrete Algebra
In Exercises 65–66, use a recursively defined sequence to find
the answer, as in Example 9.
65. Suppose you take out a car loan for $9500 at 5% annual in-
terest for 48 months. Your monthly payment is $218.78.
How much do you owe after one year (12 payments)? After
three years?
66. Lisa Chow is buying a condo. She takes out an $80,000
mortgage for 30 years at 6% annual interest. Her monthly
payment is $479.64.
(a) How much does she owe after one year (12 payments)?
(b) How much interest does Lisa pay during the first five
years? [Hint: The interest paid is the difference between
her total payments and the amount of the loan paid off
after five years
($80,000 remaining balance).]
(c) After 15 years, Lisa sells the condo and pays off the re-
maining mortgage balance. How much does she pay?
67. The number of bachelor’s degrees (in thousands) awarded
in year n is approximated by the sequence {a
n
}, where a
n
25.3n 1250 and n 0 corresponds to 2000.*
(a) Approximately how many bachelor’s degrees were
awarded in 2004 and in 2007?
(b) Approximately how many bachelor’s degrees will be
awarded between 2004 and 2009 (inclusive)?
68. The number of hours the average person spends listening to
satellite radio in year n is approximated by the sequence
{b
n
}, where n 1 corresponds to 2001 and b
n
945.54
73.57 ln n.* Round your final answers (not your calcula-
tions) to the following questions to the nearest hour.
(a) For how many hours did the average person listen to
satellite radio in 2002 and 2006?
(b) How much total time did the average person spend lis-
tening to satellite radio from 2001 to 2005 (inclusive)?
69. Consumer spending per person (in dollars) on video games
in year n is approximately c
n
.16n
2
2.7n 25.1, where
n 0 corresponds to 2000.*
(a) What was per person spending in 2005 and in 2007?
(b) How much was spent per person from 2000 to 2007
(inclusive)?
70. Book sales (in billions of dollars) in the United States are
approximated by the sequence {d
n
}, where d
n
1.89n
42.09 and n 4 corresponds to 2004.
†
(a) What were the sales in 2007?
(b) What was the total spent on books from 2001 to 2005?
*Based on data and projections from the Statistical Abstract of the
United States: 2007.
†
Based on data and projections from the Book Industry Study Group,
Inc.

THINKERS
Exercises 71–75 deal with prime numbers. A positive integer
greater than 1 is prime if its only positive integer factors are
itself and 1. For example, 7 is prime because its only factors
are 7 and 1, but 15 is not prime because it has factors other
than 15 and 1 (namely, 3 and 5).
71. (a) Let {a
n
} be the sequence of prime integers in their usual
ordering. Verify that the first ten terms are 2, 3, 5, 7, 11,
13, 17, 19, 23, 29.
(b) Find a
17
, a
18
, a
19
, a
20
.
In Exercises 72–75 find the first five terms of the sequence.
72. a
n
is the nth prime integer larger than 10. [Hint: a
1
11.]
73. a
n
is the square of the nth prime integer.
74. a
n
is the number of prime integers less than n.
75. a
n
is the largest prime integer less than 5n.
Exercises 76–82 deal with the Fibonacci sequence {a
n
} that
was discussed in Example 6.
76. Leonardo Fibonacci discovered the sequence in the thir-
teenth century in connection with this problem: A rabbit
colony begins with one pair of adult rabbits (one male, one
female). Each adult pair produces one pair of babies (one
SECTION 12.2 Arithmetic Sequences 837
male, one female) every month. Each pair of baby rabbits
becomes adult and produces the first offspring at age two
months. Assuming that no rabbits die, how many adult pairs
of rabbits are in the colony at the end of n months (n 1, 2,
3, . . .)? [Hint: It may be helpful to make up a chart listing
for each month the number of adult pairs, the number of
one-month-old pairs, and the number of baby pairs.]
77. (a) List the first 10 terms of the Fibonacci sequence.
(b) List the first 10 partial sums of the sequence.
(c) Do the partial sums follow an identifiable pattern?
78. Verify that every positive integer less than or equal to 15 can
be written as a sum of Fibonacci numbers, with none used
more than once. For example, 12 1 3 8 and 1, 3, and
8 are Fibonacci numbers.
79. Verify that 5(a
n
)
2
4(1)
n
is always a perfect square for n
1,2,...,10.
80. Verify that (a
n
)
2
a
n1
a
n1
(1)
n1
for n 2, . . . , 10.
81. Show that
k
n1
a
n
a
k2
1. [Hint: a
1
a
3
a
2
;
a
2
a
4
a
3
; etc.]
82. Show that
k
n1
a
2n1
a
2k
, that is, the sum of the first k
odd-numbered terms is the kth even-numbered term. [Hint:
a
3
a
4
a
2
; a
5
a
6
a
4
; etc.]
12.2 Arithmetic Sequences
■ Identify arithmetic sequences.
■ Find terms and a formula for an arithmetic sequence.
■ Find partial sums of arithmetic sequences.
An arithmetic sequence (sometimes called an arithmetic progression) is a
sequence in which the difference between each term and the preceding one is
always the same constant.
EXAMPLE 1
In the sequence 3, 8, 13, 18, 23, 28, . . . the difference between each term and the
preceding one is always 5. So this is an arithmetic sequence. ■
EXAMPLE 2
Which of the following sequences are arithmetic?
(a) 14, 10, 6, 2, 2, 6, 10, 14, . . .
(b) 7, 7
2
, 7
3
, 7
4
, 7
5
, . . .
(c) log 5, log 5
2
, log 5
3
, log 5
4
, log 5
5
, . . .
Section Objectives
SOLUTION
(a) The difference between each term and the preceding one is 4.
10 14 4 2 2 4,
6 10 4 6 (2) 4,
2 6 4 10 (6) 4,
and so on. Hence, the sequence is arithmetic.
(b) Note that
7
2
7 49 7 42, but 7
3
7
2
343 49 294.
Since the difference between consecutive terms is not always the same, this
sequence is not arithmetic.
(c) Using the Power Law for logarithms (Section 5.4), we see that
log 5
2
log 5 2 log 5 log 5 log 5,
log 5
3
log 5
2
3 log 5 2 log 5 log 5,
log 5
4
log 5
3
4 log 5 3 log 5 log 5,
and so on. The difference between each term and the preceding one is always
log 5 .69897, so the sequence is arithmetic. ■
If {a
n
} is an arithmetic sequence, then for each n 2, the term preceding
a
n
is a
n1
, and the difference a
n
a
n1
is some constant—call it d. Therefore,
a
n
a
n1
d or, equivalently,
The number d is called the common difference of the arithmetic sequence.
EXAMPLE 3
If {a
n
} is an arithmetic sequence with a
1
3 and a
2
4.5, find the common dif-
ference and list the first eight terms.
SOLUTION The common difference is
d a
2
a
1
4.5 3 1.5.
Therefore, the sequence begins 3, 4.5, 6, 7.5, 9, 10.5, 12, 13.5, . . . ■
EXAMPLE 4
Show that the sequence {7 4n} is arithmetic and find the common difference.
838 CHAPTER 12 Discrete Algebra
Arithmetic
Sequences
In an arithmetic sequence {a
n
},
a
n
a
n1
d
for some constant d and all n 2.
SOLUTION The sequence is arithmetic because for each n 2,
a
n
a
n1
(7 4n) [7 4(n 1)]
(7 4n) (7 4n 4) 4.
Therefore, the common difference is d 4. ■
If {a
n
} is an arithmetic sequence with common difference d, then for each
n 2, we know that a
n
a
n1
d. Applying this fact repeatedly shows that
a
2
a
1
d
a
3
a
2
d (a
1
d) d a
1
2d
a
4
a
3
d (a
1
2d) d a
1
3d
a
5
a
4
d (a
1
3d) d a
1
4d,
and in general, we have the following.
EXAMPLE 5
Find the nth term of the arithmetic sequence with first term 5 and common dif-
ference 3.
SOLUTION Since a
1
5 and d 3, the formula in the preceding box shows
that
a
n
a
1
(n 1)d 5 (n 1)3 3n 8. ■
EXAMPLE 6
What is the 45th term of the arithmetic sequence whose first three terms are
5, 9, 13?
SOLUTION The first three terms show that a
1
5 and that the common dif-
ference d is 4. Applying the formula in the box with n 45, we have
a
45
a
1
(45 1)d 5 (44)4 181. ■
EXAMPLE 7
If {a
n
} is an arithmetic sequence with a
6
57 and a
10
93, find a
1
and a formula
for a
n
.
SOLUTION Apply the formula a
n
a
1
(n 1)d with n 6 and n 10.
a
6
a
1
(6 1)d and a
10
a
1
(10 1)d
57 a
1
5d 93 a
1
9d
a
1
57 5da
1
93 9d.
SECTION 12.2 Arithmetic Sequences 839
nth Term of an
Arithmetic Sequence
In an arithmetic sequence {a
n
} with common difference d,
a
n
a
1
(n 1)d for every n 1.

These two expressions for a
1
must be equal.
57 5d 93 9d
4d 36
d 9.
Substituting d 9 in either of the equations above shows that a
1
12.
a
1
57 5(9) 12 or a
1
93 9(9) 12.
So the formula for a
n
is
a
n
a
1
(n 1)d 12 (n 1)9 9n 3. ■
PARTIAL SUMS
It’s easy to compute partial sums of arithmetic sequences by using the following
formulas.
Proof Let S denote the kth partial sum a
1
a
2
a
k
. For reasons that
will become apparent later, we shall calculate the number 2S.
2S S S (a
1
a
2
a
k
) (a
1
a
2
a
k
).
Now we rearrange the terms on the right by grouping the first and last terms
together, then the first and last of the remaining terms, and so on.
2S (a
1
a
k
) (a
2
a
k1
) (a
3
2
k2
) (a
k
a
1
).
Since adjacent terms of the sequence differ by d, we have
a
2
a
k1
(a
1
d) (a
k
d) a
1
a
k
.
Using this fact, we have
a
3
a
k2
(a
2
d) (a
k1
d) a
2
a
k1
a
1
a
k
.
Continuing in this manner, we see that every pair in the sum for 2S is equal to
a
1
a
k
. Therefore,
2S (a
1
a
k
) (a
2
a
k1
) (a
3
a
k2
) (a
k
a
1
)
(a
1
a
k
) (a
1
a
k
) (a
1
a
k
) (a
1
a
k
)(k terms)
k(a
1
a
k
).
840 CHAPTER 12 Discrete Algebra
Partial Sums of an
Arithmetic Sequence
If {a
n
} is an arithmetic sequence with common difference d, then for each
positive integer k, the kth partial sum can be found by using either of these
formulas.
1.
k
n1
a
n
2
k
(a
1
a
k
)or
2.
k
n1
a
n
ka
1
k(k
2
1)
d.
Dividing both sides of this last equation by 2 shows that S
2
k
(a
1
a
k
). This
proves the first formula. To obtain the second one, note that
a
1
a
k
a
1
[a
1
(k 1)d] 2a
1
(k 1)d.
Substituting the right side of this equation in the first formula for S shows that
S
2
k
(a
1
a
k
)
2
k
[2a
1
(k 1)d] ka
1
k(k
2
1)
d.
This proves the second formula. ■
EXAMPLE 8
Find the 12th partial sum of the arithmetic sequence that begins 8, 3, 2,
7, . . . .
SOLUTION We first note that the common difference d is 5. Since a
1
8
and d 5, the second formula in the box with k 12 shows that
12
n1
a
n
12(8)
12(
2
11)
5 96 330 234. ■
EXAMPLE 9
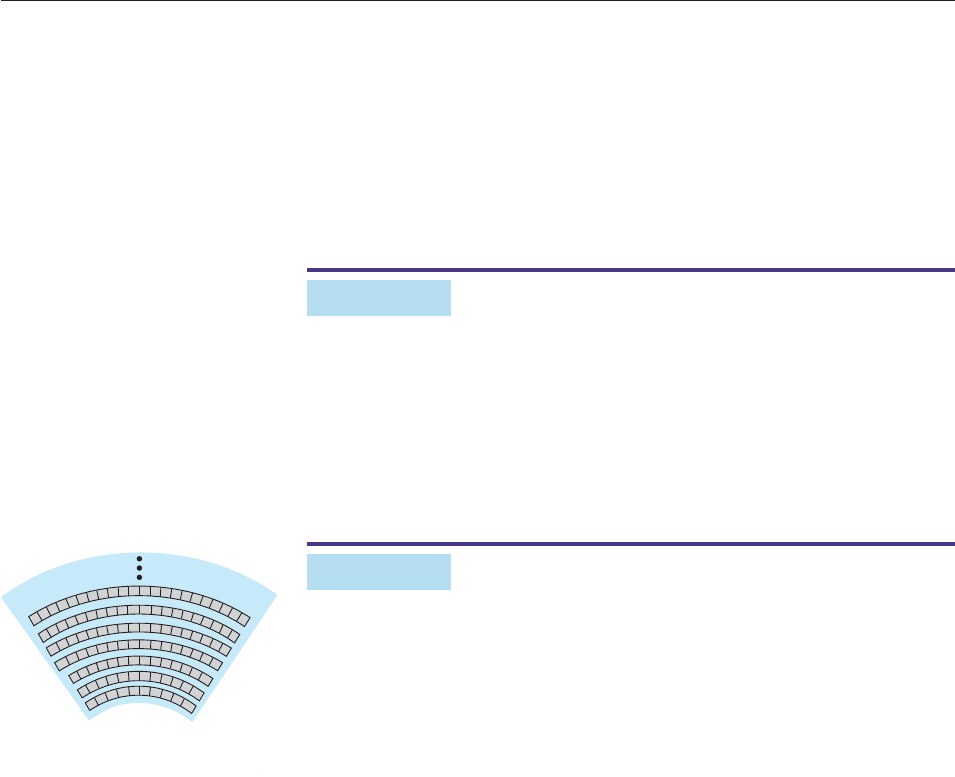
A corner section of a stadium has 28 rows of seats. There are 10 seats in the
first row, 12 in the second row, 14 in the third row, and so on, as indicated in
Figure 12–12.
(a) How many seats are in the 20th row?
(b) How many seats are in the entire section?
SOLUTION
(a) Let a
n
be the number of seats in row n. Then a
1
10, a
2
12, a
3
14, and
so on. Thus, we have an arithmetic sequence, with common difference d 2.
According to the box before Example 5, the nth term of the sequence is
a
n
a
1
(n 1)d
a
n
10 (n 1)2 8 2n.
The number of seats in the 20th row is
a
20
8 2(20) 48.
(b) The total number of seats in all 28 rows is the 28th the partial sum of the
sequence {a
n
}, which is given by the second formula in the box on page 840.
k
n1
a
n
ka
1
k(k
2
1)
d.
Here k 28, a
1
10, and d 2, so
28
n1
a
n
28(10)
28(28
2
1)
2
280 28(27) 1036 seats. ■
SECTION 12.2 Arithmetic Sequences 841
Figure 12–12

EXAMPLE 10
Find the sum of all multiples of 3 from 3 to 333.
SOLUTION The answer can be found in two ways.
Algebraic Method: This sum is a partial sum of the arithmetic sequence 3, 6,
9, 12, . . . . Since this sequence can be written in the form
3
1, 3
2, 3
3, 3
4, 3
5, 3
6, . . . ,
we see that 333 3
111 is the 111th term. The 111th partial sum of this
sequence can be found by using the first formula in the box with k 111, and
a
1
3, and a
111
333.
111
n1
a
n
11
2
1
(3 333)
11
2
1
(336) 18,648.
Calculator Method: Note that the nth term of the sequence 3, 6, 9, . . . is 3n.
Consequently, we can compute the 111th partial sum as in Figure 12–13. ■
EXAMPLE 11
If the starting salary for a job is $20,000 and you get a $2000 raise at the begin-
ning of each subsequent year, what will your salary be during the tenth year? How
much will you earn during the first ten years?
SOLUTION Your yearly salary rates form a sequence: 20,000, 22,000, 24,000,
26,000, and so on. It is an arithmetic sequence with a
1
20,000 and d 2000.
Your tenth-year salary is
a
10
a
1
(10 1)d 20,000 9
2000 $38,000.
Your ten-year total earnings are the tenth partial sum of the sequence.
1
2
0
(a
1
a
10
)
1
2
0
(20,000 38,000) 5(58,000) $290,000. ■
842 CHAPTER 12 Discrete Algebra
EXERCISES 12.2
In Exercises 1–8, determine whether the sequence is arithmetic
or not. If it is, find the common difference.
1. 1, 3, 5, 7, 9, . . . 2.
1
3
,
2
3
,
3
3
,
4
3
,
5
3
, . . .
3. 1, 2, 4, 8, 16, . . . 4. 9, 6, 3, 0, . . .
5. log 1, log 2, log 4, log 8, log 16, . . .
6. log 3, log 6, log 9, log 12, log, 15, . . .
7.
1
3
,
4
6
,
1
9
5
,
3
1
2
2
,
5
1
5
5
,
8
1
4
8
, . . .
8.
1
2
,
3
4
, 1,
3
4
,
1
2
,
1
4
, . . .
In Exercises 9–16, write the first five terms of the sequence
whose nth term is given. Use them to decide whether the
sequence is arithmetic. If it is, list the common difference.
9. a
n
5 4n 10. b
n
n
5
4
11. c
n
(1)
n
12. d
n
2n
1
n
13. a
n
2 (1)
n
n 14. c
n
1
n
3
15. a
n
e
ln n
16. b
n
3
7n
2
Figure 12–13

SECTION 12.2 Arithmetic Sequences 843
In Exercises 17–24, show that the sequence is arithmetic and
find its common difference.
17. {3 2n} 18. {1.5 1.5n}
19.
4
n
3
20.
3
n
2
21.
5
2
3n
22.
p
2
n
23. {c 2n}(c constant)
24. {2b 3nc}(b, c constants)
In Exercises 25–32, the first term a
1
and the common difference
d of an arithmetic sequence are given. Find the fifth term and
the formula for the nth term.
25. a
1
5, d 2 26. a
1
4, d 5
27. a
1
4, d
1
4
28. a
1
6, d
2
3
29. a
1
10, d
1
2
30. a
1
p, d
1
5
31. a
1
8, d .1 32. a
1
.1, d 8
In Exercises 33–40, use the given information about the arith-
metic sequence with common difference d to find a
1
and a for-
mula for a
n
.
33. a
4
12, d 2 34. a
7
8, d 3
35. a
3
3, d 5 36. a
4
5, d 5
37. a
2
4, a
6
32 38. a
7
6, a
12
4
39. a
5
0, a
9
6 40. a
5
3, a
9
18
In Exercises 41–48, find the kth partial sum of the arithmetic
sequence {a
n
} with common difference d.
41. k 6, a
1
2, d 5 42. k 8, a
1
2
3
, d
4
3
43. k 7, a
1
3
4
, d
1
2
44. k 9, a
1
4, d
1
2
45. k 6, a
1
4, a
6
14 46. k 10, a
1
0, a
10
30
47. k 9, a
1
6, a
9
24 48. k 8, a
1
6, a
8
13
In Exercises 49–54, find the sum.
49.
20
n1
(3n 4) 50.
25
n1
n
4
5
51.
30
n1
3
n
2
52.
35
n1
2n
8
4
53.
40
n1
n
6
3
54.
30
n1
4
3
6n
55. The sequence in which
a
n
per capita amount spent on health services and
supplies in year n,
with n 1 corresponding to 1999, is approximately arith-
metic.*
(a) If the per capita amount was $4154 in 1999 and $5864
in 2004, find a formula for a
n
.
(b) Use the sequence to estimate the per capita amount in
2005 and 2008.
56. The sequence in which
b
n
remaining life expectancy of a man at age n
is approximately arithmetic.
†
(a) Use the fact that a man’s remaining life expectancy is
60.6 years at age 15 and 51.2 years at age 25 to find a
formula for b
n
.
(b) Determine the remaining life expectancy of a man at
these ages: 20, 22, 30 and 40.
57. A lecture hall has six seats in the first row, eight in the sec-
ond, ten in the third, and so on, through row 12. Rows 12
through 20 (the last row) all have the same number of seats.
Find the number of seats in the lecture hall.
58. A monument is constructed by first laying a row of
60 bricks at ground level. A second row, with two fewer
bricks, is centered on that; a third row, with two fewer bricks,
is centered on the second; and so on. The top row contains 10
bricks. How many bricks are there in the monument?
59. A ladder with nine rungs is to be built, with the bottom rung
24 inches wide and the top rung 18 inches wide. If the
lengths of the rungs decrease uniformly from bottom to top,
how long should each of the seven intermediate rungs be?
60. Find the first eight numbers in an arithmetic sequence in
which the sum of the first and seventh term is 40 and the
product of the first and fourth terms is 160.
61. Find the sum of all the even integers from 2 to 100.
62. Find the sum of all the integer multiples of 7 from 7 to 700.
63. Find the sum of the first 200 positive integers.
64. Find the sum of the positive integers from 101 to 200
(inclusive). [Hint: What’s the sum from 1 to 100? Use it and
Exercise 63.]
65. A business makes a $10,000 profit during its first year. If the
yearly profit increases by $7500 in each subsequent year,
what will the profit be in the tenth year and what will the
total profit for the first 10 years be?
66. If a man’s starting salary is $24,000 and he receives a $1000
increase every six months, what will his salary be during the
last six months of the sixth year? How much will he earn
during the first six years?
*U.S. Centers for Medicare and Medicaid Services
†
National Center for Health Statistics
844 CHAPTER 12 Discrete Algebra
67. The sequence with
a
n
with n 1 corresponding to 1994, is approximately arith-
metic.*
(a) If sales were $136.4 billion in 1994 and $217.4 billion
in 2003, find the formula for a
n
.
(b) Estimate holiday sales in 2005.
(c) Find the holiday sales from 2000 to 2005.
68. Let {b
n
} be the sequence that gives U.S. personal income in
year n (in trillions of dollars), where n 1 corresponds to
2000. This sequence is approximately arithmetic.
†
(a) If personal income was $8.4 trillion in 2000 and
$9.7 trillion in 2004, find a formula for b
n
.
(b) Estimate personal income in 2005.
(c) Find the total personal income from 2000 to 2005.
holiday retail sales in year n
(in billions of dollars),
69. Let n 1 correspond to 1981. The arithmetic sequence in
which
average yearly earnings of a production
c
n
worker in manufacturing in year n
has c
1
$15,828.80 and c
19
$28,932.80.*
(a) Find a formula for c
n
.
(b) Find the total earnings of an average production worker
from 1981 to 2005.
70. Let n 1 correspond to 2001. The arithmetic sequence in
which
d
n
hours per person spent on the Internet in year n
has d
1
136 and d
6
205.
†
(a) Find a formula for d
n
.
(b) How many hours per person were spent on the Internet
in 2007?
(c) Find the total number of hours per person on the Inter-
net from 2001 to 2005.
*Based on data from the U.S. Bureau of Labor Statistics.
†
Based on data and projections in Statistical Abstract of the United
States: 2006.
*Based on data from the U.S. Department of Commerce.
†
Based on data from the U.S. Bureau of Economic Analysis.
12.3 Geometric Sequences
■ Identify geometric sequences.
■ Find terms and a formula for a geometric sequence.
■ Find partial sums of geometric sequences.
■ Use geometric sequences to solve applied problems.
A geometric sequence (sometimes called a geometric progression) is a
sequence in which the quotient of each term and the preceding one is the same
constant r. This constant r is called the common ratio of the geometric sequence.
EXAMPLE 1
The sequence 3, 9, 27, . . . 3
n
, . . . is geometric with common ratio 3. For instance,
a
2
/a
1
9/3 3 and a
3
/a
2
27/9 3. If 3
n
is any term (n 2), then the
preceding term is 3
n1
, and
3
3
n
n
1
3
3
n
3
n
1
1
3. ■
EXAMPLE 2
Which of the following sequences are geometric?
(a)
1
2
,
2
3
,
3
4
,
4
5
,
5
6
, . . . (b) e, e
3
, e
5
, e
7
, e
9
, . . . (c)
2
5
n
Section Objectives
SOLUTION
(a) Note that
a
a
2
1
2
1
/
/
3
2
2
3
2
1
4
3
, but
a
a
3
2
3
2
/
/
4
3
3
4
3
2
9
8
.
Since the quotient of consecutive terms is not always the same, this sequence
is not geometric.
(b) Compute the quotient of each term and the preceding one.
a
a
2
1
e
e
3
e
2
,
a
a
4
3
e
e
5
7
e
2
,
a
a
3
2
e
e
5
3
e
2
,
a
a
5
4
e
e
9
7
e
2
,
and so on. The consecutive quotients are always the number e
2
, so the
sequence is geometric with common ratio e
2
.
(c) For each n 2, the term preceding a
n
is a
n1
. Here,
a
n
2
5
n
and a
n1
2
n
5
1
,
so
a
a
n
n
1
5
5
/
/
2
2
n
n
1
2
5
n
2
n
5
1
2
2
n
n
1
1
2
.
Since the quotient of each term and the preceding one is always 1/2, the
sequence is geometric with common ratio 1/2. ■
Suppose {a
n
} is a geometric sequence with common ratio r. For each n 2,
the quotient of the term a
n
and the preceding one is
a
a
n
n
1
r. Multiplying both
sides of the equation by a
n1
produces this fact.
Applying this last formula for n 2, 3, 4, . . . , we have
a
2
ra
1
,
a
3
ra
2
r(ra
1
) r
2
a
1
,
a
4
ra
3
r(r
2
a
1
) r
3
a
1
,
a
5
ra
4
r(r
3
a
1
) r
4
a
1
,
and in general we have the following.
SECTION 12.3 Geometric Sequences 845
Geometric
Sequences
In a geometric sequence {a
n
} with common ratio r,
a
n
ra
n1
for each n 2.
nth Term of a
Geometric Sequence
If {a
n
} is a geometric sequence with common ratio r, then for all n 1,
a
n
r
n1
a
1
.
