Helms L.L. Potential Theory

Подождите немного. Документ загружается.

228 5 Dirichlet Problem for Unbounded Regions

function u such that u(x) < lim inf

y→x,y∈Λ

u(y)=+∞. It can be assumed

that u is nonnegative on a ball B containing x and, in fact, that u = G

where μ is a measure on B.Sinceu = G

μ diﬀers from the Newtonian

(logarithmic) potential U

by a harmonic function that is continuous at x,

itcanbeassumedthat

u(y)=U

(y)=



u(y, z) dμ(z)

where u(y, z)=u

(z). Extend μ to R

by putting μ(R

∼ B) = 0. The map

τ induces a measure μτ

−1

on the Borel subsets M ⊂ R

by the equation

(μτ

−1

)(M)=μ(τ

−1

(M)). Consider

μτ

−1

(y)=



u(y, z) d(μτ

−1

)(z).

Applying the transformation τ,

μτ

−1

(y)=



u(y, τz) dμ(z).

Therefore,

μτ

−1

(τy)=



u(τy,τz) dμ(z).

Since |τy − τz|≤|y − z|,u(τy,τz) ≥ u(y,z)andU

μτ

−1

(τy) ≥ U

(y). Note

that U

μτ

−1

is superharmonic with

μτ

−1

(τx)=



u(τx,τz) dμ(z)=



u(x, z) dμ(z)=U

(x) < +∞.

Since |τx− τy| = |x − y| for all y,

lim

z→τx,z∈τ (Λ)

μτ

−1

(z) = lim

τy→τx,y∈Λ

μτ

−1

(τy) ≥ lim

y→x,y∈Λ

(y)=+∞.

Thus, U

μτ

−1

(τx) < lim

z→x,z∈τ (Λ)

μτ

−1

(z)andτ(Λ)isthinatτx by

Theorem 5.6.4.

5.7 Thinness and Regularity

Regularity of boundary points relative to the Dirichlet problem can be

characterized in terms of thinness. The latter will be characterized in terms

of regularized reduced functions ﬁrst.

5.7 Thinness and Regularity 229

Deﬁnition 5.7.1 An extended real-valued function u deﬁned on the open

set Ω peaks at x ∈ Ω if u(x) > sup

y∈Ω∼O

u(y) for every neighborhood O

of x.

If Ω is Greenian, there is no problem in ﬁnding a positive superharmonic

function u that peaks at x ∈ Ω,namely,u = G

(x, ·).

Theorem 5.7.2 Let Ω be a Greenian set and let u be a positive superhar-

monic function that peaks at x ∈ Ω.AsetΛ ⊂ Ω is thin at x if and only if

(x) <u(x).

Proof: Note that Λ is thin at x if and only if Λ ∼{x} is thin at x.By

Corollary 4.6.4,

Λ∼{x}

. It therefore can be assumed that x ∈ Λ,in

which case

(x)=R

(x). It will be shown now that R

(x) <u(x) implies

that Λ is thin at x.SinceΛ is thin at x if x is not a limit point of Λ,itcanbe

assumed that x is a limit point of Λ.SinceR

(x) <u(x), there is a positive

superharmonic function v on Ω that majorizes u on Λ such that v(x) <u(x).

Therefore,

lim inf

y→x,y∈Λ

v(y) ≥ lim inf

y→x,y∈Λ

u(y) ≥ u(x) >v(x)

and Λ is thin at x by Theorem 5.6.4. Suppose now that Λ is thin at x.Ifx is

not a limit point of Λ, it is easily seen that R

(x) <u(x)sinceu peaks at x.

Assuming that x is limit point of Λ, by Theorem 5.6.4 there is a potential v on

Ω such that v(x) < lim inf

y→x,y∈Λ

v(y).Itcanbeassumedthatv is bounded

above on Ω by replacing v by min (v,v(x) + 1) if necessary. Let α be such that

v(x) <α<lim inf

y→x,y∈Λ

v(y). For λ>0, let w

= u(x)+λ(v−α). There is a

neighborhood O of x such that v(y) >αfor y ∈ O∩Λ.Thenw

≥ u(x) ≥ u(y)

for y ∈ O∩Λ since u peaks at x.Moreover,u(x)−u(y) ≥ γ>0fory ∈ Ω ∼ O

since u peaks at x.Sincev is bounded, a positive number λ

canbechosenso

that λ

|v(y)−α|≤γ ≤ u(x)−u(y)fory ∈ Ω ∼ O.Thenλ

(v(y)−α) ≥ u(y)−

u(x)fory ∈ Ω ∼ O and w

(y)=u(x)+λ

(v(y) −α) ≥ u(y)fory ∈ Ω ∼ O.

Since w

(y) ≥ u(y)fory ∈ O ∩ Λ, w

≥ u on Λ, and therefore w

≥ R

By choice of α, u(x) >u(x)+λ

(v(x) − α)=w

(x) ≥ R

(x) ≥

(x).

Lemma 5.7.3 If B is a ball of radius

, then there is a positive, continuous,

superharmonic function u on B such that for each x ∈ B the function u

has a decomposition into a sum of two positive, continuous, superharmonic

functions of which one peaks at x.

Proof: Only the n ≥ 3 case will be discussed, the n = 2 case being essentially

the same. Deﬁne

u(y)=



|y −z|

n−2

dz, y ∈ B.

It follows from Theorem 3.4.12 that u is a positive, continuous, superharmonic

function on B.Consideranyx ∈ B and choose r>0 such that B

−

x,r

⊂ B.

Letting

230 5 Dirichlet Problem for Unbounded Regions

(y)=



x,r

|y − z|

n−2

dz, y ∈ B

(y)=



B∼B

x,r

|y −z|

n−2

dz, y ∈ B,

u = u

+ u

. Using Gauss’ Averaging Principle, Theorem 1.6.2, to evaluate

the integrals, it can be shown that u

peaks at x.

Theorem 5.7.4 The set of points of Λ ⊂ R

where Λ is thin is a polar set.

Proof: Since thinness is a local property and a countable union of polar sets

is again polar, it suﬃces to consider the case for which Λ is a subset of a ball of

radius

. Suppose Λ is thin at x ∈ Λ.Letw = w

+ w

be the superharmonic

function described in the preceding lemma. Taking reduced functions relative

to B,

(x) <w

(x) by Theorem 5.7.2. By Theorem 4.3.5,

(x) ≤

(x) <w

(x)+w

(x)=w(x)=R

(x)

and x ∈{y;

(y) < R

(y)}. By Corollary 4.6.2, the latter set is polar and

it follows that the set of points in Λ where Λ is thin is a polar set.

Corollary 5.7.5 AsetZ ⊂ R

is polar if and only if it is thin at each of

its points.

Proof: If Z is thin at each of its points, then Z is polar by the preceding

theorem. By Theorem 5.6.2, a polar set is thin at each of its points.

Regularity of a boundary point of a Greenian region Ω can be related to

thinness of ∼ Ω at the point.

Lemma 5.7.6 Let F be a closed subset of R

.ThenF is not thin at the

ﬁnite point x ∈ ∂F if and only if there is a positive superharmonic function

w deﬁned on some neighborhood O of x such that lim

y→x,y∈O∼ F

w(y)=0.

Proof: Suppose F is not thin at the ﬁnite point x ∈ ∂F. It can be assumed

that F ⊂ B = B

x,δ

.Alsoletu(y)=δ −|y −x| for y ∈ B.Thenu is a positive

superharmonic function on B that peaks at x.Consider

relative to B.

Then

(x)=u(x) by Theorem 5.7.2 and

lim inf

y→x,y∈B∼F

(y) ≥ lim inf

y→x,y∈B

(y) ≥

(x)=u(x).

Consider the superharmonic function w = u −

on B ∼ F .Ifw van-

ishes at some point of B ∼ F , it must be identically zero on B ∼ F by the

minimum principle; this would imply that u is harmonic on B ∼ F since

is harmonic there, a contradiction since the Laplacian of u is strictly

negative. Thus, w>0onB ∼ F . Moreover, lim sup

y→x,y∈B∼F

w(y) ≤

u(x) − lim inf

y→x,B∼F

(y) ≤ 0. Therefore, lim

y→x,y∈B∼F

w(y)=0.

5.7 Thinness and Regularity 231

This proves the necessity with O = B. To prove the converse, let w be a

positive, superharmonic function on O ∼ F for some neighborhood O of x

such that lim

y→x,y∈O∼ F

w(y) = 0, and let B = B

x,δ

⊂ O. It can be assumed

that O = B. Assume that F is thin at x; that is, there is a subharmonic

function v on B such that v(x)=1andv(z) ≤−1onB ∩(F ∼{x}) accord-

ing to Theorem 5.6.4. Let B

be a second ball with center x with B

−

⊂ B.

Since v ≤−1onF ∩ ∂B

,v < 0 on a neighborhood W of F ∩ ∂B

,and

for any positive number λ, v − λw ≤ 0onW ∩ (∂B

∼ F ). Since w>0on

∂B

∼ W, inf

z∈∂B

∼W

w(z) > 0. Along with the fact that v is bounded above

on ∂B

∼ W ,thisimpliesthatλ

> 0 can be chosen so that v − λ

w ≤ 0on

∂B

∼ W .Sincev − λ

w ≤ 0onW ∩ (∂B

∼ F )also,

lim sup

z→y,z∈B

∼F

(v(z) − λ

w(z) − u

(z)) ≤ 0

for any >0 whenever y ∈ ∂B

∼ F . This inequality also holds at points y ∈

−

∩(∂F ∼{x}) for in a neighborhood of such a point v is strictly negative;

it also holds at x, since in this case the left side is −∞. By Corollary 2.3.6,

v − λ

w − u

≤ 0onB

∼ F for every >0. Therefore, v ≤ λ

w on

∼ F and lim sup

y→x,y∈B

∼F

v(y) ≤ 0. Since lim sup

y→x,y∈F ∼{x}

v(y) ≤

−1,v(x) ≤ lim sup

y→x,y=x

v(y) ≤ 0. But by Theorem 2.4.7, 1 = v(x)=

lim sup

y→x,y∈B

v(y), a contradiction.

Theorem 5.7.7 A ﬁnite point x ∈ ∂Ω is a regular boundary point for the

Greenian set Ω if and only if ∼ Ω is not thin at x.

Proof: By the preceding lemma, ∼ Ω is not thin at the ﬁnite point x ∈ ∂Ω

if and only if there is a barrier at x. The existence of a barrier is necessary

and suﬃcient for regularity by Remark 5.4.11 and Theorem 5.4.2.

Corollary 5.7.8 AlinesegmentI ⊂ R

is not thin at its end points.

Proof: It will be shown ﬁrst that I cannot be thin at an interior point x.

Since thinness is a local property, any closed subinterval of I containing x as

an interior point will be thin at x if I is thin at x. It suﬃces to show that

a closed line segment I cannot be thin at an interior point. Let B be a ball

containing I in its interior. Regarding x as a boundary point B ∼ I,x is a

regular boundary point for B ∼ I by Theorem 2.6.27 and I is not thin at

x by the preceding theorem. Suppose now that I is thin at an end point x.

Then it follows from Theorem 5.6.4 that there is a superharmonic function

w such that w(x) < lim

y→x,y∈I∼{x}

w(y)=+∞. Since superharmonicity is

invariant under a rotation about x, a line segment I



can be constructed

having x as an interior point and a superharmonic function w



(as a sum of

w and two rotations of w about x) can be constructed such that w



(x) <

lim

y→x,y∈I



∼{x}



(y); that is, I



is thin at an interior point, but this is not

possible by the ﬁrst part of the proof.

232 5 Dirichlet Problem for Unbounded Regions

Theorem 5.7.9 If Ω ⊂ R

and ∼ Ω is thin at x, then there are arbitrarily

small ρ>0 such that (∼ Ω) ∩ ∂B

x,ρ

= ∅.

Proof: If x is not a limit point of ∼ Ω, the assertion is trivially true. Let x be

a limit point of ∼ Ω,andlet(r, θ) be the polar coordinates of a point in the

plane relative to the pole x and a ray  having x as an end point. Consider

the map τ : R

→  taking the point (r, θ)intothepoint(r, 0). It is easily

seen that |τx − τy| = |x − y| for all y ∈ R

. Suppose there is a ρ

> 0such

that (∼ Ω) ∩B

x,ρ

= ∅ for all ρ ≤ ρ

.Thenτ(∼ Ω) contains the line segment

 = {(ρ, 0) : 0 <ρ≤ ρ

}.Sinceτ(∼ Ω)isthinatx by Theorem 5.6.6,

 is

thin at x, but this contradicts the preceding corollary and there is no such ρ.

Remark 5.7.10 (Proof of Theorem 2.6.28)LetΩ be an open subset of

,letx ∈ ∂Ω,andlet be a continuum in ∼ Ω containing the point x.By

Theorem 5.7.7, x is a regular boundary point for Ω if and only if ∼ Ω is not

thin at x. Assume that ∼ Ω is thin at x. By the above theorem, there is a ρ>0

such that (∼ Ω)∩∂B

x,ρ

= ∅.Since∩∂B

x,ρ

= ∅,=(B

x,ρ

∩)∪(∼ B

−

x,ρ

∩),

contradicting the connectedness of .Thus,Ω is not thin at x and x is a

regular boundary point for Ω.

Theorem 5.7.11 (Brelot [9]) Let Ω be a Greenian subset of R

,x ∈

Ω,Λ ⊂ Ω,andu any nonnegative superharmonic function on Ω.Then

(i) if x ∈ cl

Λ,thenδ

(x, {x})=1, G

(x, ·)=G

(x, ·),and

Λ∩U

(x)=

u(x) for all neighborhoods U of x,and

(ii) if x ∈ cl

Λ,thenδ

(x, {x})=0, G

(x, ·) = G

(x, ·),andlim

U↓{x}

Λ∩U

=0on Ω whenever u(x) < +∞ ,

Proof: (i)Ifx ∈ cl

Λ and U is any neighborhood of x,thenx is a ﬁne

limit point of Λ and therefore a ﬁne limit point of Λ ∩U . By Corollary 4.6.2,

Λ∩U

= u quasi everywhere on Λ ∩ U . Thus, there is a polar set Z ⊂ Λ ∩ U

such that

Λ∩U

= u on (Λ ∩ U) ∼ Z.SinceZ does not have any ﬁne

limit points by Theorem 5.5.2, there is a ﬁne neighborhood O

of x such

that Z ∩ O

= ∅.Thus,

Λ∩U

= u on (Λ ∩ U) ∩ O

. Since both func-

tions are ﬁnely continuous,

Λ∩U

(x)=u(x). Taking u = G

(x, ·)and

U = Ω,G

(x, x)=

(x, ·),x)=G

(x, x) by the preceding step. From

the deﬁnition of δ

,δ

(x, {x})=δ

(x, {x})=1.(ii) Suppose x ∈ cl

Λ.By

adjoining a countable set to Λ, it can be assumed that x is a limit point of Λ.

This modiﬁcation will have no eﬀect on reductions since the set of augmented

points is a polar set. Since x is not a ﬁne limit point of Λ, the latter is thin at x.

By Theorem 5.6.4, there is a superharmonic function v such that v(x) < +∞

and lim

y→x,y∈Λ∼{x}

u(y)=+∞.If>0, choose a ball B with center at x

such that u>1/ on Λ ∩ (B ∼{x}). By Theorem 4.7.4 and Lemma 4.3.5,

Λ∩B

(x, {x}) ≤ δ

Λ∩B

(x, Ω)=

Λ∩B

(x) ≤ 

Λ∩B

(x) ≤ u(x); that is,

lim

B↓{x}

Λ∩B

(x, {x}) = 0. It follows from Lemma 4.7.9 that δ

(x, {x})=0

5.7 Thinness and Regularity 233

for if δ

(x, {x})=1,thenδ

Λ∩U

(x, {x}) = 1 for all neighborhoods U of x.

The assertion that lim

U↓{x}

Λ∩U

=0onΩ follows from the same lemma.

Lemma 5.7.12 If Ω is a Greenian subset of R

, then there is a bounded,

continuous, nonnegative superharmonic function w satisfying

Λ ∩ Ω = {x;

(x)=w(x)}

for every Λ ⊂ Ω.

Proof: Let {B

} be a sequence of balls with closures in Ω that forms a basis

for the topology of Ω,andlet

w =

∞



j=1

−j

on Ω.Since2

−j

≤ 2

−j

and

is continuous on Ω, w is a bounded,

continuous, superharmonic function on Ω by the Weierstrass M-test and

Theorem 2.4.8. By Theorem 4.6.13, for any Λ ⊂ Ω

∞



j=1

−j

(

, ·).

If x ∈ cl

Λ ∩ Ω,then

(x)=w(x) by Theorem 5.7.11. Consider any

x ∈ Ω ∼ cl

Λ and those j for which x ∈ B

.Since

(

,x)canbe

made arbitrarily small by making j suﬃciently large according to (iii)of

Lemma 4.7.9 and

(x)=1forsuchj, w(x) >

(x).

Theorem 5.7.13 If u is the potential of a measure μ on the Greenian set

Ω,Λ ⊂ Ω,and

= G

,thenμ

is supported by cl

Λ;inparticular,ifu

is a nonnegative superharmonic function on Ω, the Riesz measure associated

with

is supported by cl

Λ.

Proof: It will be shown ﬁrst that δ

(x, ·) is supported by cl

Λ;thatis,

(x, Ω ∼ cl

Λ)=0.Letw be a superharmonic function as described

in the preceding lemma. Since

(

, ·) by Lemma 4.7.5,



w(y) δ

(·,dy), and

(

, ·)=



(y) δ

(·,dy),



(w(y) −

(y)) δ

(x, dy)=0,x∈ Ω.

Since w ≥

, {y; w(y) >

(y)} = Ω ∼ cl

Λ has δ

(x, ·) measure zero;

that is, δ

(x, ·) is supported by cl

Λ.Nowletu = G

μ be the potential of

ameasureμ on Ω,andlet



(y,·) μ(dy).

234 5 Dirichlet Problem for Unbounded Regions

Clearly, μ

is also supported by cl

Λ.Since

(x)=



(x, z)



(y,dz) μ(dy)







(x, z)δ

(y,dz)



μ(dy)



(y,x) μ(dy)



(x, y) μ(dy)

= G

μ(x)

by Theorem 5.4.16 and

= G

μ by Theorem 5.4.17,

= G

.Thus,

= μ

and μ

is supported by cl

Λ. Lastly, suppose u is a nonnegative

superharmonic function on Ω,letν

be the Riesz measure associated with

and let v

= G

. Using the notation just introduced, ν

= ν

and ν

is therefore supported by cl

Λ.

Theorem 5.7.14 (Brelot [9]) Let u be a nonnegative superharmonic func-

tion on the Greenian set Ω and let μ be the Riesz measure associated with u.

(i) If x ∈ Ω,then

f-lim

y→x,y∈Ω

u(y)

(x, y)

= μ({x})= inf

y∈Ω∼{x}

u(y)

(x, y)

. (5.10)

(ii) If x ∈ Ω is a limit point of Λ ⊂ Ω and Λ is thin at x, then there is a

potential u on Ω for which

lim

y→x,y∈Λ

u(y)

(x, y)

=+∞. (5.11)

Conversely, if there is a nonnegative superharmonic function u on Ω ∼{x}

satisfying Equation (5.11), then Λ is thin at x.

Proof: (i) Let c denote the third expression in Equation (5.10). Since

u(y)=



(z,y) dμ(z) ≥ μ({x})G

(x, y)

for y ∈ Ω ∼{x},c ≥ μ({x}). On the other hand, u − cG

(x, ·) ≥ 0on

Ω ∼{x} and is superharmonic thereon. By Theorem 4.2.15, the diﬀerence

has a superharmonic extension v on Ω.Thus,u = cG

(x, ·)+v so that

u =



(z,·) d(cδ

{x}

+ ν)(z)+h

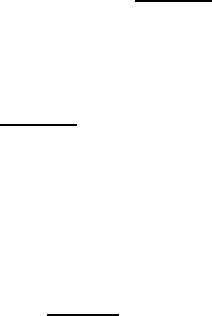
5.7 Thinness and Regularity 235

where ν is the Riesz measure and h is the harmonic function associated with

v. Since the Riesz decomposition is unique according to Theorem 3.5.11,

μ({x}) ≥ c.Thus,μ({x})=c and the right equation in (i)isproved.Itcan

be assumed that both sides of the second equation in (i) vanish, for if not,

replace u by the nonnegative superharmonic function u − μ({x})G

(x, ·),

extended across {x} as above. Assume that

f-limsup

y→x,y∈Ω

u(y)

(x, y)

> 0.

Then there is a b>0 and a ﬁne neighborhood U

of x such that

u(y)

(x, y)

≥ b for y ∈ U

Since x ∈ U

⊂ cl

, by Theorem 5.7.11

u ≥

≥ bG

(x, ·)=bG

(x, ·)

so that

(x, ·)

≥ b>0,

a contradiction. (ii) The second assertion of (ii) will be proved ﬁrst. If

u is a nonnegative superharmonic function on Ω ∼{x} for which Equa-

tion (5.11) holds, then u has a nonnegative superharmonic extension to Ω by

Theorem 4.2.15. According to Equation (5.10), there is a ﬁne neighborhood

of x on which u/G

(x, ·) is bounded above on O

∼{x} by some con-

stant M<+∞. On the other hand, by Equation (5.11) there is an ordinary

neighborhood U of x such that u/G

(x, ·) >M on (U ∩Λ) ∼{x}. Therefore,

∩ U) ∼{x}) ∩ Λ = ∅.Thus,x is not a ﬁne limit point of Λ and Λ is

therefore thin at x. To prove the ﬁrst assertion of (ii), let x be a limit point

of Λ ⊂ Ω which is thin at x. It suﬃces to show that there is a potential u on

the component of Ω containing x satisfying Equation (5.11). It therefore can

be assumed that Ω is connected. Let y be a ﬁxed point of ∈ Ω ∼{x}.Since

(x, y) < +∞,by(ii) of Theorem 5.7.11 there is a decreasing sequence

} of neighborhoods of x with y ∈∼ U

−

such that

Λ∩U

(x, y))(y) < 2

−n

,n≥ 1.

Since

Λ∩U

(x, ·))(y)=R

Λ∩U

(x, ·))(y), there is a positive, super-

harmonic function u

majorizing G

(x, ·)onΩ ∩U

such that u

(y) < 2

−n

By replacing u

by min (u

(x, ·)), it can be assumed that each u

is a

potential. Letting u =



∞

n=1

, u is superharmonic on Ω since u(y) < +∞.

236 5 Dirichlet Problem for Unbounded Regions

Since u ≥ nG

(x, ·)onΛ ∩ U

for each n ≥ 1, Equation (5.11) holds. By

considering the Riesz decomposition of u, it can be assumed that u is a po-

tential on Ω.

Theorem 5.7.15 Let Λ ⊂ R

and let φ be any inversion with respect to a

sphere.

(i) Either φ(Λ) is thin at φ(∞) for every inversion φ,orφ(Λ) is not thin

at φ(∞) for any inversion φ.

(ii) If Λ is unbounded, then φ(Λ) is thin at φ(∞) for every inversion φ if

and only if there is a nonnegative superharmonic function u on a deleted

neighborhood of ∞ such that

lim

y→∞,y∈Λ

u(y)

log (y)

=+∞ if n =2

lim

y→∞,y∈Λ

u(y)=+∞ if n ≥ 3;

in the n ≥ 3 case, there is a nonnegative superharmonic function u on R

satisfying the last equation.

(iii) If x and φ(x) are ﬁnite, φ(Λ) is thin at φ(x) if and only if Λ is thin at x.

Proof: Since (i)and(ii) apply only to unbounded sets, Λ will be assumed

to be unbounded. (i)(n = 2) Consider an arbitrary inversion y → y

∗

relative

toasphere∂B where B = B

x,δ

. Suppose Λ

∗

is thin at x. By replacing Λ by

Λ ∩ (∼ B

−

), if necessary, it can be assumed that Λ

∗

⊂ B.Sincex is a limit

point of Λ

∗

, according to Theorem 5.7.14 there is a potential v on B such

that

lim

y→x,y∈Λ

∗

v(y)

(x, y)

=+∞.

Since G

(x, y)=−log |x − y| + h

where h

is harmonic on B,

lim

y→x,y∈Λ

∗

−

v(y)

log |x − y|

=+∞.

Letting v

∗

denote the Kelvin transform of v,

lim

∗

→∞,y

∗

∈Λ

∗

)

log |y

∗

− x|

= lim

y→x,y∈Λ

∗

v(y)

log δ

− log |y − x|

= lim

y→x,y∈Λ

∗

−

v(y)

log |y − x|

=+∞.

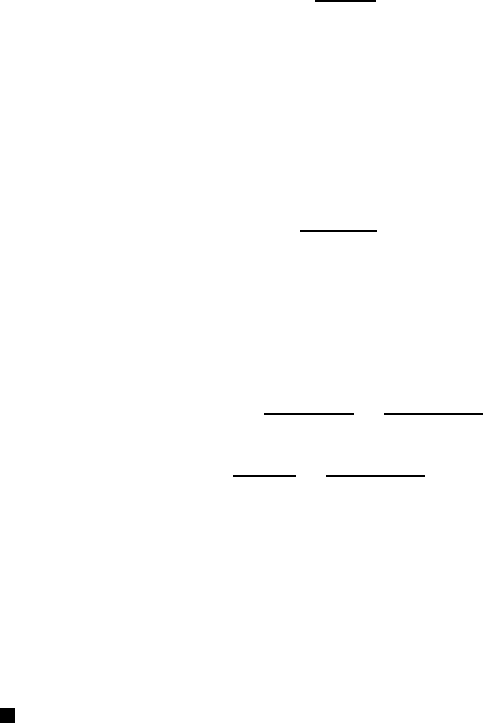
5.7 Thinness and Regularity 237

Since lim

∗

→∞,y

∗

∈Λ

(log |y

∗

− x|/ log |y

∗

|)=1,

lim

∗

→∞,y

∗

∈Λ

∗

)

log |y

∗

=+∞.

Letting u = v

∗

, lim

y→∞,y∈Λ

u(y)/ log |y| =+∞.Thisproves(ii)inthen =2

case. Since the preceding steps are reversible, the existence of such a function

u on a neighborhood of x suﬃces to prove that Λ

∗

is thin at x for every

inversion with respect to a sphere. (ii)(n ≥ 3) Again consider an arbitrary

inversion with respect to a sphere ∂B where B = B

x,δ

, and suppose Λ

∗

thin at x. By Theorem 5.7.14, there is a potential v on R

such that

lim

y→x,y∈Λ

∗

v(y)

(x, y)

=+∞.

Since it is easily seen that

lim

y→x,y∈Λ

∗

(x, y)/G(x, y)=1

and

G(x, y)=

|x − y|

n−2

|x − y

∗

n−2

2n−4

v(y)

G(x, y)

2n−4

v(y)

|x − y

∗

n−2

Since the expression on the right is just v

∗

), the Kelvin transform of v,

lim

∗

→∞,y

∗

∈Λ

∗

)=+∞.

Since v

∗

is a nonnegative superharmonic function on R

∼{x},ithasa

superharmonic extension to R

by Theorem 4.2.15 which will be denoted by

the same symbol. Letting u(y)=v

∗

), lim

y→∞,y∈Λ

u(y)=+∞. As before,

the preceding steps are reversible. Assertion (iii) follows from Remark 5.3.11.

Deﬁnition 5.7.16 The ﬁne topology on R

∞

is the smallest topology con-

taining the set {∞}, ﬁnely open subsets of R

,andsetsΛ ⊂ R

∞

for which

φ(Λ ∼{∞}) is a deleted ﬁne neighborhood of φ(∞) for every inversion φ

with respect to a sphere.

Since a set Λ is thin at x ∈ R

if and only if ∼ Λ is a ﬁne neighborhood of x,

the requirement that φ(Λ ∼{∞}) is a deleted ﬁne neighborhood of φ(∞)

holds for all inversions if it holds for one. The fact that {∞} is ﬁnely open in

∞

means that ∞ is ﬁnely isolated and cannot be a ﬁne limit point of any

subset of R

∞

Deﬁnition 5.7.17 AsetΛ ⊂ R

∞

is thin at ∞ if φ(Λ ∼{∞})isthinat

φ(∞) for every inversion φ with respect to a sphere.