Helms L.L. Potential Theory
Подождите немного. Документ загружается.


88 2 The Dirichlet Problem
Since the two extreme limits are the same,
lim sup
z→x,z∈Ω
H
r
(z) = lim sup
z→x,z∈Ω
u(z)=0.
Remark 2.6.30 It is worth noting an interesting fact in the preceding proof.
Namely, the fact that lim
z→y,z∈Ω
(αv(z) − u(z)) ≥ 0 for all y ∈ ∂Ω except
possibly for a single point was enough to prove that αv − u ≥ 0onΩ,as
though a single point is negligible. The proof was accomplished by exploiting
the fact that u
x
=+∞ at x. The same proof would have worked for more
than one point if a superharmonic function u could be found that takes on
the value +∞ on the set of points where lim
z→y,z∈Ω
(αv(z) − u(z)) ≥ 0 fails
to hold. This suggests that sets of points where a superharmonic function
takes on the value +∞ are negligible. Such sets are called polar sets and
will be taken up later.
Corollary 2.6.31 If Ω is an open subset of R
n
,n ≥ 2, then there is an
increasing sequence {Ω
j
} of regular bounded open sets with closures in Ω
such that Ω = ∪Ω
j
.
Proof: Let {Γ
j
} be an increasing sequence of compact sets such that Ω =
∪Γ
j
.ConsiderΓ
1
and a finite covering of Γ
1
by balls B
(1)
1
,...,B
(1)
k
1
having
closures in Ω. By slightly increasing the radii of some of the balls, it can
be assumed that each boundary point of Ω
1
= ∪
k
1
j=1
B
(1)
j
is the vertex of a
truncated closed solid cone lying outside Ω
1
; that is, it can be assumed that
Ω
1
is a regular region containing Γ
1
. Apply the same procedure to Ω
−
1
∪Γ
2
,
etc.
2.7 The Radial Limit Theorem
If h = PI(μ : B) on the ball B and μ is the indefinite integral of a continuous
boundary function f relative to surface area σ on ∂B, then lim
z→x,z∈B
h(z)=
f(x) for all x ∈ ∂B. Since the Radon-Nikodym derivative of μ with respect to
σ is equal to fa.e.(σ), the boundary behavior of h is related to the derivative
dμ/dσ. Since there are several ways of defining a derivate, different boundary
limit theorems may be obtained depending upon the definition of the derivate
used. Generally speaking, a definition that places stringent conditions on μ
for the existence of the derivate will yield the strongest results. In this section,
a weak derivate will be used first to describe the behavior of h along radial
approaches to the boundary of B.
As a matter of notational convenience, let B = B
0,1
. It is not essential
the 0 is the center of the ball or that the radius is equal to 1. If z ∈ ∂B and
0 ≤ α ≤ π/2,C
z,α
will denote a closed cone with vertex 0, axis coincident
with the vector z, and half-angle α;thatis,

2.7 The Radial Limit Theorem 89
C
z,α
= {y;cosα ≤
(y,z)
|y||z|
≤ 1} .
Each such cone determines a closed polar cap K
z,α
= C
z,α
∩∂B having center
z and spherical angle α.
Let μ be a signed measure of bounded variation on the Borel subsets of
∂B.
Definition 2.7.1 If lim
σ(K)→0
μ(K)/σ(K) exists, where K is any closed po-
lar cap centered at x, the limit is called the symmetric derivate of μ with
respect to σ at x and is denoted by D
s
μ(x).
The symmetric derivate is a weak derivate and results in the following
weak boundary limit theorem. Although the theorem is stated for harmonic
functions, versions of the theorem are true for superharmonic functions.
Littlewood [41] proved an a.e. radial limit theorem for potentials G
B
μ in
the n = 2 case, F. Riesz [53] extended Littlewood’s theorem to positive su-
perharmonic functions in the n = 2 case, and Privalov [51, 52] extended the
latter to the n ≥ 3case.
Theorem 2.7.2 Let μ be a signed measure of bounded variation on the Borel
subsets of ∂B and let h = PI(μ : B).IfD
s
μ exists at x ∈ ∂B,then
lim
λ→1
− h(λx)=D
s
μ(x).
Proof: (B = B
0,1
)Itwillbeshownfirstthata = D
s
μ(x),x fixed, can be
assumed to be zero. Consider the signed measure μ − aσ which is also of
bounded variation. For this measure, D
s
(μ−aσ)(x)=D
s
μ(x)−aD
s
σ(x)=0
and PI(μ−aσ : B)=PI(μ : B)−aPI(σ : B)=h−a.Ifitcanbeshownthat
lim
λ→1
−
(h(λx) −a) = 0, it would follow that lim
λ→1
−
h(λx)=a. Therefore,
by replacing μ by μ − aσ, it can be assumed that a =0.Forx ∈ ∂B,
h(λx)=
1
σ
n
∂B
1 −|λx|
2
|z − λx|
n
dμ(z)
=
1
σ
n
∂B
1 − λ
2
|z − λx|
n
dμ(z).
If γ denotes the angle between λx and the point z ∈ ∂B,then
h(λx)=
1
σ
n
∂B
1 − λ
2
(1 + λ
2
− 2λ cos γ)
n/2
dμ(z)
=
1
σ
n
∂B
1 − λ
2
((1 − λ)
2
+4λ sin
2
(γ/2))
n/2
dμ(z).
With λ fixed, the integrand depends only upon γ and a “zonal region” method
can be used to evaluate the integral. Letting α(γ)=μ(K
x,γ
), 0 ≤ γ ≤ π, α is
of bounded variation on [0,π]and
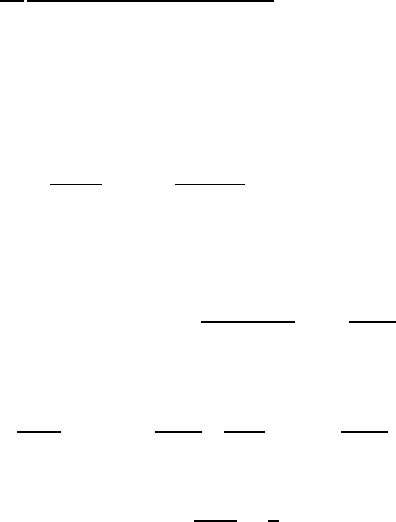
90 2 The Dirichlet Problem
h(λx)=
π
0
P (λ, γ) dα(γ)
where
P (λ, γ)=
1
σ
n
1 − λ
2
((1 − λ)
2
+4λ sin
2
(γ/2))
n/2
, 0 <λ<1, 0 ≤ γ ≤ π.
Since lim
γ→0
μ(K
x,γ
)/σ(K
x,γ
)=0,α(0) = μ(K
x,0
) = 0. For each λ ∈
(0, 1),P(λ, ·) is positive, monotone decreasing on [0,π], and has a continuous
derivative P
γ
(λ, ·)on[0,π]. Consider also the function β(γ)=σ(K
x,γ
), 0 ≤
γ ≤ π,forwhich
lim
γ→0
|α(γ)|
β(γ)
= lim
γ→0
μ(K
x,γ
σ(K
x,γ
)
= |D
s
μ(x)| =0.
Expressing the surface area of K
x,γ
as an integral and transforming to spher-
ical coordinates (r, θ),
β(γ)=σ(K
x,γ
)=σ
n−1
sin γ
0
r
n−2
(1 − r
2
)
1/2
dr ≥
σ
n−1
n − 1
sin
n−1
γ
and so
lim sup
γ→0
γ
n−1
β(γ)
≤ lim sup
γ→0
n − 1
σ
n−1
γ
sin γ
n−1
=
n − 1
σ
n−1
< +∞.
Choose >0 such that
lim sup
γ→0
γ
n−1
β(γ)
<
1
and then choose φ ∈ (0,π) such that |α(γ)|≤β(γ)andγ
n−1
≤ β(γ)for
all γ ∈ [0,φ]. Integrating by parts and noting that P
γ
(λ, γ) ≤ 0,
φ
0
P (λ, γ) dα(γ)=α(φ)P(λ, φ) −
φ
0
α(γ)P
γ
(λ, γ) dγ
≤ α(φ)P(λ, φ) −
φ
0
|α(γ)|P
γ
(λ, γ) dγ
≤ α(φ)P(λ, φ) −
φ
0
β(γ)P
γ
(λ, γ) dγ
= P (λ, φ)(α(φ) −β(φ)) +
φ
0
P (λ, γ) dβ(γ)
≤ P (λ, φ)(|α(φ)|−β(φ)) +
φ
0
P (λ, γ) dβ(γ)
≤
φ
0
P (λ, γ) dβ(γ).
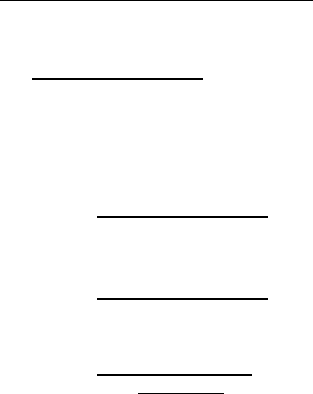
2.7 The Radial Limit Theorem 91
Since
1=PI(σ : B)(λx)=
∂B
P (λ, γ) dσ(z)=
π
0
P (λ, γ) dβ(γ),
integrating by parts
1 ≥
φ
0
P (λ, γ) dβ(γ)
= β(φ)P (λ, φ) −
φ
0
β(γ)P
γ
(λ, γ) dγ
≥ β(φ)P (λ, φ) −
φ
0
γ
n−1
P
γ
(λ, γ) dγ
= P (λ, φ)(β(φ) −φ
n−1
)+(n − 1)
φ
0
P (λ, γ)γ
n−2
dγ
≥
φ
0
P (λ, γ)γ
n−2
dγ.
Hence,
h(λx)=
φ
0
P (λ, γ) dα(γ)+
π
φ
P (λ, γ) dα(γ)
≤
φ
0
P (λ, γ) β(γ)+
π
φ
P (λ, γ) dα(γ)
φ
0
P (λ, γ) dβ(γ)
≤ +
π
φ
P (λ, γ) dα(γ)
φ
0
P (λ, γ)γ
n−2
dγ
.
Letting V
α
denote the total variation of α,
|h(λx)|≤ +
π
φ
P (λ, γ) dV
α
(γ)
φ
0
P (λ, γ)γ
n−2
dγ
≤ +
P (λ, φ)
π
φ
dV
α
(γ)
φ
0
P (λ, γ)γ
n−2
dγ
≤ +
|μ|
φ
0
P (λ,γ)γ
n−2
P (λ,φ)
dγ
.

92 2 The Dirichlet Problem
By Fatou’s Lemma, applied sequentially,
lim inf
λ→1
−
φ
0
P (λ, γ)γ
n−2
P (λ, φ)
dγ ≥
φ
0
lim inf
λ→1
−
P (λ, γ)γ
n−2
P (λ, φ)
dγ
=
φ
0
sin
n
(φ/2)
γ
n−2
sin
n
(γ/2)
dγ
=+∞,
and therefore lim sup
λ→1
−
|h(λx)|≤.Since can be taken arbitrarily small,
lim
λ→1
−
h(λx)=0.
Example 2.7.3 Consider B = B
0,1
and the function
f(x, y)=
1(x, y) ∈ ∂B,y ≥ 0
0(x, y) ∈ ∂B,y < 0.
Let μ(M)=
M
fdσfor each Borel set M ⊂ ∂B.ThenD
s
μ exists for (x, y) ∈
∂B and is equal to 1, 0, or 1/2accordingtoy>0,y <0, or y = 0, respectively.
Consider the harmonic function h = PI(μ : B). Then lim
λ→1
−
h(λx, λy)=
1, 0, or 1/2for(x, y) ∈ ∂B according to y>0,y < 0, or y = 0, respectively.
2.8 Nontangential Boundary Limit Theorem
Obtaining stronger results concerning the boundary behavior of harmonic
functions necessitates replacement of the symmetric derivate by a more re-
strictive derviate.
Definition 2.8.1 If lim
σ(K)→0
μ(K)/σ(K) exists, where K is any closed po-
lar cap containing x, the limit is called the derivate of μ with respect to σ
at x and is denoted by Dμ(x).
Proof of the existence of a derivate requires a discussion of the Vitali
covering theorem. Let X be a metric space with metric d.IfF ⊂ X,the
diameter of F is defined to be d(F )=sup{d(x, y); x, y ∈ F }.IfF ⊂ X and
x ∈ X, the distance from F to x is denoted by d(F, x)=inf{d(y, x); y ∈ F }.
An -neighborhood of F ⊂ X is denoted by N(F, )={x; d(F, x) <}.
Definition 2.8.2 Let μ be a measure defined on the Borel subsets of a com-
pact metric space X.AsetA ⊂ X is said to be covered in the sense of
Vitali by a family F of closed sets if each F ∈Fhas positive μ measure and
there is a constant α such that each point of A is contained in elements F ∈F
of arbitrarily small positive diameter for which μ(N(F, 3d(F )))/μ(F ) ≤ α.
Note that if A is covered in the sense of Vitali by F, then any subset of A
is also. A proof of the following theorem can be found in [18].
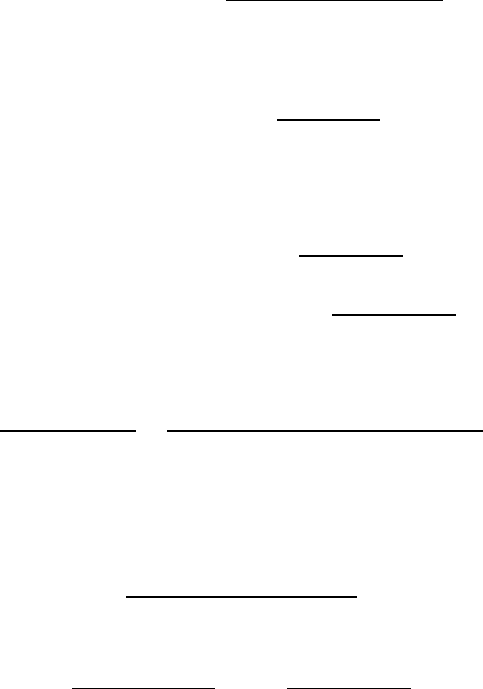
2.8 Nontangential Boundary Limit Theorem 93
Theorem 2.8.3 (Vitali Covering Theorem) Let μ be the completion of
a finite measure on the σ-algebra of Borel subsets of a compact metric space
X.IfA ⊂ X is covered in the sense of Vitali by a family F of closed sets,
then there is a sequence {F
j
} of disjoint sets in F such that μ(A ∼∪F
j
)=0.
The Vitali covering theorem will be applied in the following context. For X
take ∂B where B = B
0,ρ
⊂ R
n
. The family F will consist of all nondegenerate
closed polar caps K ⊂ ∂B. To show that F covers ∂B in the sense of Vitali,
it is necessary to estimate the surface area of polar caps.
Let K = K
x,α
, 0 <α≤ π/2, be a closed polar cap on ∂B.Makingfree
use of the fact that surface area is invariant under rotations of the sphere by
assuming that x =(0, 0....,0,ρ),
σ(K)=
···
y
2
1
+···+y
2
n−1
≤ρ
2
sin
2
α
ρ
(ρ
2
− y
2
1
−···−y
2
n−1
)
1/2
dy
1
...dy
n−1
.
Introducing the spherical coordinates (r, θ)for(y
1
,...,y
n−1
),
σ(K)=ρσ
n−1
ρ sin α
0
r
n−2
(ρ
2
− r
2
)
1/2
dr.
It is easy to see that d(K)=2ρ sin α. There is also an α
0
with 0 <α
0
<π/2
such that for α ≤ α
0
σ(N(K, 3d(K)) ≤ ρσ
n−1
7ρ sin α
0
r
n−2
(ρ
2
− r
2
)
1/2
dr
= ρσ
n−1
7
n−1
ρ sin α
0
r
n−2
(ρ
2
− 49r
2
)
1/2
dr.
Therefore,
σ(N(K, 3d(K))
σ(K)
≤
7
n−1
ρ sin α
0
(r
n−2
/(ρ
2
− 49r
2
)
1/2
) dr
ρ sin α
0
(r
n−2
/(ρ
2
− r
2
)
1/2
) dr
.
Considering the integrals as functions of their upper limits and applying the
second mean value theorem of the differential calculus, there is a point ξ with
0 <ξ<ρsin α such that the quotient on the right is equal to
7
n−1
(ξ
n−2
/(ρ
2
− 49ξ
2
)
1/2
)
ξ
n−2
/(ρ
2
− ξ
2
)
1/2
.
Therefore,
σ(N(K, 3d(K)))
σ(K)
≤ 7
n−1
(ρ
2
− ξ
2
)
1/2
(ρ
2
− 49ξ
2
)
1/2
.
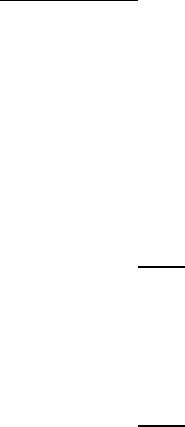
94 2 The Dirichlet Problem
As α → 0,ξ → 0 and the right member has the limit 7
n−1
. This shows that
there is a constant α
0
with 0 <α
0
<π/2 such that
σ(N(k, 3d(K)))
σ(K)
≤ constant
whenever K = K
x,α
is a closed polar cap with spherical angle α<α
0
.There-
fore, the family F of closed polar caps covers ∂B in the sense of Vitali. Unless
stated otherwise, K will denote a closed polar cap in ∂B where B = B
0,ρ
.
Lemma 2.8.4 Let μ be a measure on the Borel subsets of ∂B,andlet
0 <r<+∞.
(i) If for each x in a set A ⊂ ∂B
lim inf
σ(K)→0,x∈K
μ(K)
σ(K)
<r,
then each neighborhood of A contains an open set O such that σ(A ∼ O)=
0 and μ(O) <rσ(O).
(ii) If for each x in a set A ⊂ ∂B
lim sup
σ(K)→0,x∈K
μ(K)
σ(K)
>r,
then each neighborhood of A contains a Borel set A
0
such that σ(A ∼
A
0
)=0and μ(A
0
) >rσ(A
0
).
Proof: To prove (i), let U be any open set with A ⊂ U ⊂ ∂B,andletF be
the family of all closed polar caps K ⊂ U satisfying μ(K) <rσ(K). Then
F covers A in the sense of Vitali. By the Vitali covering theorem, there is a
sequence {K
j
} of disjoint closed polar caps in F such that σ(A ∼∪K
j
)=0.
Let K
◦
j
be the interior, relative to ∂B,ofK
j
.Sinceσ(K
j
∼ K
◦
j
)=0,σ(A ∼
K
◦
j
)=0and
μ(K
◦
j
) ≤ μ(K
j
) <rσ(K
j
)=rσ(K
◦
j
).
Letting O =
K
◦
j
, O is open, O ⊂ U,andσ(A ∼ O)=0.Moreover,
μ(O)=
∞
j=1
μ(K
◦
j
) <r
∞
j=1
σ(K
◦
j
)=rσ(O).
To prove (ii), let U be an open set such that A ⊂ U ⊂ ∂B.Byhypothesis,
the family F of closed polar caps K ⊂ U satisfying μ(K) >rσ(K)covers
A in the sense of Vitali. By the Vitali covering theorem, there is a sequence
{K
j
} of disjoint closed polar caps in F such that σ(A ∼
K
j
)=0.Let
A
0
=
K
j
.Since

2.8 Nontangential Boundary Limit Theorem 95
μ(A
0
)=
∞
j=1
μ(K
j
) >r
∞
j=1
σ(K
j
)=rσ(A
0
),
A
0
satisfies the requirements of (ii).
It is assumed throughout the remainder of this section that σ is a complete
measure.
Lemma 2.8.5 Let μ be a measure on the Borel subsets of ∂B such that
Dμ(x) = lim
σ(K)→0,x∈K
μ(K)/σ(K) exists for almost all (σ) points x ∈ ∂B,
and define Dμ arbitrarily at points where the limit does not exist. Then Dμ
is Lebesgue measurable on ∂B.
Proof: For each positive integer m,let{k
m1
,...,k
mj
m
} be a finite collection
of closed polar caps which covers ∂B and σ(K
mj
) < 1/m, j =1,...,j
m
.
Define a sequence of functions {f
m
} on ∂B as follows. Let K
m0
= ∅.If
x ∈ K
mj
∼∪
j−1
i=1
K
mi
,j =1,...,j
m
,letf
m
(x)=μ(K
mj
)/σ(K
mj
). Then
each f
m
is measurable and the sequence {f
m
} converges a.e.(σ)toDμ.
Theorem 2.8.6 (Lebesgue) Let μ be a signed measure of bounded varia-
tion on the Borel subsets of ∂B.ThenDμ(x) exists for almost all (σ) points
x ∈ ∂B and is Lebesgue measurable.
Proof: By decomposing μ into its positive and negative variations, it can be
assumed that μ is a measure. Let A be the set of points x ∈ ∂B for which
lim sup
σ(K)→0,x∈K
μ(K)
σ(K)
> lim inf
σ(K)→0,x∈K
μ(K)
σ(K)
.
If i is a nonnegative integer and j is a positive integer, let A
ij
be the set of
x ∈ ∂B where
lim sup
σ(K)→0,x∈K
μ(K)
σ(K)
>
i +1
j
>
i
j
> lim inf
σ(K)→0,x∈K
μ(K)
σ(K)
.
Suppose σ
∗
(A) > 0whereσ
∗
is the outer measure on ∂B induced by σ.Since
A =
A
ij
,σ
∗
(A
ij
) > 0forsomei and j.Given>0, there is an open set U
such that A
ij
⊂ U and σ(U ) <σ
∗
(A
ij
)+. Applying (i) of Lemma 2.8.4 with
r replaced by i/j, there is an open set O such that O ⊂ U, σ(A
ij
∼ O)=0,
and
μ(O) <
i
j
σ(O) <
i
j
(σ
∗
(A
ij
)+).
Applying (ii) of Lemma 2.8.4 to the set A
ij
∩O,thereisaBorelsetM ⊂ O
such that σ((A
ij
∩ O) ∼ M)=0andμ(M) > ((i +1)/j)σ(M). Since
A
ij
⊂ M ∪ (A
ij
∼ O) ∪ ((A
ij
∩ O) ∼ M )

96 2 The Dirichlet Problem
and
σ(A
ij
∼ O)=0=σ((A
ij
∩O) ∼ M ),
σ(M) ≥ σ
∗
(A
ij
). Thus,
i
j
(σ
∗
(A
ij
)+) >μ(O) ≥ μ(M ) >
i +1
j
σ(M) ≥
i +1
j
σ
∗
(A
ij
).
Letting → 0, (i/j)σ
∗
(A
ij
) ≥ ((i +1)/j)σ
∗
(A
ij
), a contradiction. Therefore,
σ(A)=0andDμ(x) = lim
σ(K)→0,x∈K
μ(K)/σ(K) exists as a finite or infinite
limit for all x ∈ A.LetZ = {x ∈∼ A; Dμ(x)=+∞}.By(ii) of Lemma 2.8.4,
for each positive integer j there is a Borel set Z
j
⊂ ∂B such that σ(Z ∼
Z
j
)=0andμ(Z
j
) >jσ(Z
j
). Since σ
∗
(Z) ≤ σ
∗
(Z ∩ Z
j
)+σ
∗
(Z ∼ Z
j
)=
σ
∗
(Z ∩ Z
j
) ≤ σ
∗
(Z
j
),
+∞ >μ(∂B) ≥ μ(Z
j
) >jσ(Z
j
) ≥ jσ
∗
(Z)
for all positive integers j. Therefore, σ
∗
(Z)=0andDμ<+∞a.e.(σ)on
∼ A. The measurability of Dμ follows from the preceding lemma.
The proof of the principal boundary limit theorem requires the exclusion
of null sets of boundary points in addition to those where Dμ is not defined.
Theorem 2.8.7 (Lebesgue) Let μ be a signed measure of bounded varia-
tion on the Borel subsets of ∂B.
(i) If f is an integrable function on ∂B and μ(M)=
M
fdμfor each Borel
set M ⊂ ∂B,thenDμ = fa.e.(σ).
(ii) If μ is singular relative to σ,thenDμ =0a.e.(σ).
Proof: Suppose μ(M)=
M
fdσwith f integrable. It can be assumed that
f ≥ 0 by decomposing f into its positive and negative parts, if necessary.
Let A = {x ∈ ∂B; f(x) > D μ(x)} and assume that σ(A) > 0. There are then
positive numbers α and β such that A
αβ
= {x ∈ ∂B; f(x) >α>β>Dμ(x)}
and σ(A
αβ
) > 0. Since σ is a regular Borel measure, there is a compact set
Γ ⊂ A
αβ
with σ(Γ ) > 0. Thus,
μ(Γ )=
Γ
fdσ≥ ασ(Γ ). (2.8)
Since Dμ(x) = lim
σ(K)→0,x∈K
μ(K)/σ(K) <βfor all x ∈ Γ , each neighbor-
hood of Γ contains an open set O such that μ(O) <βσ(O)andσ(Γ ∼ O)=0
by part (i) of Lemma 2.8.4. Since σ(Γ ∼ O)=0andμ is the indefinite
integral of an integrable function, a small open set can be adjoined to O
so that Γ ⊂ O and μ(O) <βσ(O). Since Γ is the intersection of a se-
quence of such open sets O, μ(Γ ) ≤ βσ(Γ ) <ασ(Γ ) ≤ μ(Γ ), the latter
inequality holding by Inequality (2.8), a contradiction. Therefore, σ(A)=0.
In a similar manner, using part (ii) of Lemma 2.8.4, it can be shown that
σ({x ∈ ∂B; f(x) < Dμ(x)}) = 0. Therefore, Dμ = fa.e.(σ). Suppose now
2.8 Nontangential Boundary Limit Theorem 97
that μ is singular relative to σ.Sinceμ = μ
+
− μ
−
and the positive and
negative variations of μ are singular relative to σ, it can be assumed that μ
is a measure and Dμ ≥ 0. There is then a measurable set M ⊂ ∂B such that
σ(M)=0andμ(∂B ∼ M )=0.LetP = {x ∈ ∂B; Dμ(x) > 0} and assume
that σ(P) > 0. Since Dμ is measurable and σ(M)=0,thereisan>0and
acompactsetΓ ⊂ P ∼ M such that σ(Γ) > 0andDμ(x) >for x ∈ Γ .
By (ii) of Lemma 2.8.4, each neighborhood Γ has μ measure greater than
σ(Γ). Since Γ is the intersection of such neighborhoods, μ(Γ ) ≥ σ(Γ ) > 0;
but this contradicts the fact that 0 ≤ μ(Γ ) ≤ μ(P ∼ M) ≤ μ(∂B ∼ M)=0.
Therefore, σ(P )=0andDμ =0a.e.(σ).
Points x ∈ ∂B satisfying the equation of the following theorem are called
Lebesgue points.
Theorem 2.8.8 (Lebesgue) Let f be a measurable function on ∂B which is
integrable relative to σ,andletμ(M)=
M
fdσfor each Borel set M ⊂ ∂B.
Then
lim
σ(K)→0,x∈K
1
σ(K)
K
|f(y) − f (x)|dσ(y)=0
a.e.(σ) on ∂B.
Proof: Let {r
i
} be an enumeration of the rationals. By the preceding the-
orem, for each i ≥ 1thereisasetZ
i
⊂ ∂B with σ(Z
i
) = 0 such that for
x ∈ Z
i
,
lim
σ(K)→0.x∈K
1
σ(K)
K
|f(y) − r
i
|dσ(y)=|f(x) − r
i
|.
Then Z =
Z
i
has surface area zero. Let x ∈ ∂B ∼ Z,let>0, and choose
r
j
such that |f(x) − r
j
| </2. Then
lim
σ(K)→0,x∈K
1
σ(K)
K
|f(y) − f (x)|dσ(y)
≤ lim sup
σ(K)→0,x∈K
1
σ(K)
K
(|f(y) − r
j
|+ |r
j
− f (x)|) dσ(y)
≤ lim sup
σ(K)→0,x∈K
1
σ(K)
K
|f(y) − r
j
|dσ(y)+
2
= |f(x) − r
j
| +
2
<.
It is not difficult to construct examples of harmonic functions h on ∂B ⊂
R
2
that behave badly in a neighborhood of a point z ∈ ∂B. In fact, suppose
h = PI(δ
z
: B)onB = B
0,ρ
,whereδ
z
is a unit measure supported by the
singleton {z}.Forx ∈ B,
h(x)=
1
2πρ
ρ
2
−|x|
2
|z −x|
2
.
