Hassani S. Mathematical Methods: For Students of Physics and Related Fields
Подождите немного. Документ загружается.

19.1 Complex Functions 503
The functions u(x, y)andv(x, y) of an analytic function have an interesting
property which the following example investigates.
Example 19.1.6.
The family of curves u(x, y) = constant is perpendicular to curves of constant
u and v are
perpendicular.
the family of curves v(x, y) = constant at each point of the complex plane where
f(z)=u + iv is analytic.
This can easily be seen by looking at the normal to the curves. The normal to
the curve u(x, y) = constant is simply ∇u = ∂u/∂x, ∂u/∂y (see Theorem 12.3.2).
Similarly, the normal to the curve v(x, y)=constant is∇v = ∂v/∂x, ∂v/∂y.
Taking the dot product of these two normals, we obtain
(∇u) · (∇v)=
∂u
∂x
∂v
∂x
+
∂u
∂y
∂v
∂y
=
∂u
∂x
−
∂u
∂y
+
∂u
∂y
∂u
∂x
=0
by the C–R conditions.
Historical Notes
One can safely say that rigorous complex analysis was founded by a single man:
Cauchy. Augustin-Louis Cauchy was one of the most influential French mathe-
maticians of the nineteenth century. He began his career as a military engineer, but
when his health broke down in 1813 he followed his natural inclination and devoted
himself wholly to mathematics.
In mathematical productivity Cauchy was surpassed only by Euler, and his col-
lected works fill 27 fat volumes. He made substantial contributions to number theory
and determinants; is considered to be the originator of the theory of finite groups;
and did extensive work in astronomy, mechanics, optics, and the theory of elasticity.
Augustin-Louis
Cauchy 1789–1857
His greatest achievements, however, lay in the field of analysis. Together with his
contemporaries Gauss and Abel, he was a pioneer in the rigorous treatment of limits,
continuous functions, derivatives, integrals, and infinite series. Several of the basic
tests for the convergence of series are associated with his name. He also provided the
first existence proof for solutions of differential equations, gave the first proof of the
convergence of a Taylor series, and was the first to feel the need for a careful study
of the convergence behavior of Fourier series. However, his most important work
was in the theory of functions of a complex variable, which in essence he created and
which has continued to be one of the dominant branches of both pure and applied
mathematics. In this field, Cauchy’s integral theorem and Cauchy’s integral formula
are fundamental tools without which modern analysis could hardly exist.
Unfortunately, his personality did not harmonize with the fruitful power of his
mind. He was an arrogant royalist in politics and a self-righteous, preaching, pious
believer in religion—all this in an age of republican skepticism—and most of his
fellow scientists disliked him and considered him a smug hypocrite. It might be fairer
to put first things first and describe him as a great mathematician who happened
also to be a sincere but narrow-minded bigot.
19.1.2 Integration of Complex Functions
We have thus far discussed the derivative of a complex function. The concept
of integration is even more important because, as we shall see later, derivatives
can be written in terms of integrals.
504 Complex Derivative and Integral
The definite integral of a complex function is naively defined in analogy
to that of a real function. However, a crucial difference exists: While in the
complex integrals
are
path-dependent.
real case, the limits of integration are real numbers and there is only one way
to connect these two limits (along the real line), the limits of integration of
a complex function are points in the complex plane and there are infinitely
many ways to connect these two points. Thus, we speak of a definite integral
of a complex function along a path. It follows that complex integrals are, in
general, path-dependent.
#
α
2
α
1
f(z) dz = lim
N→∞
Δz
i
→0
N
i=1
f(z
i
)Δz
i
, (19.7)
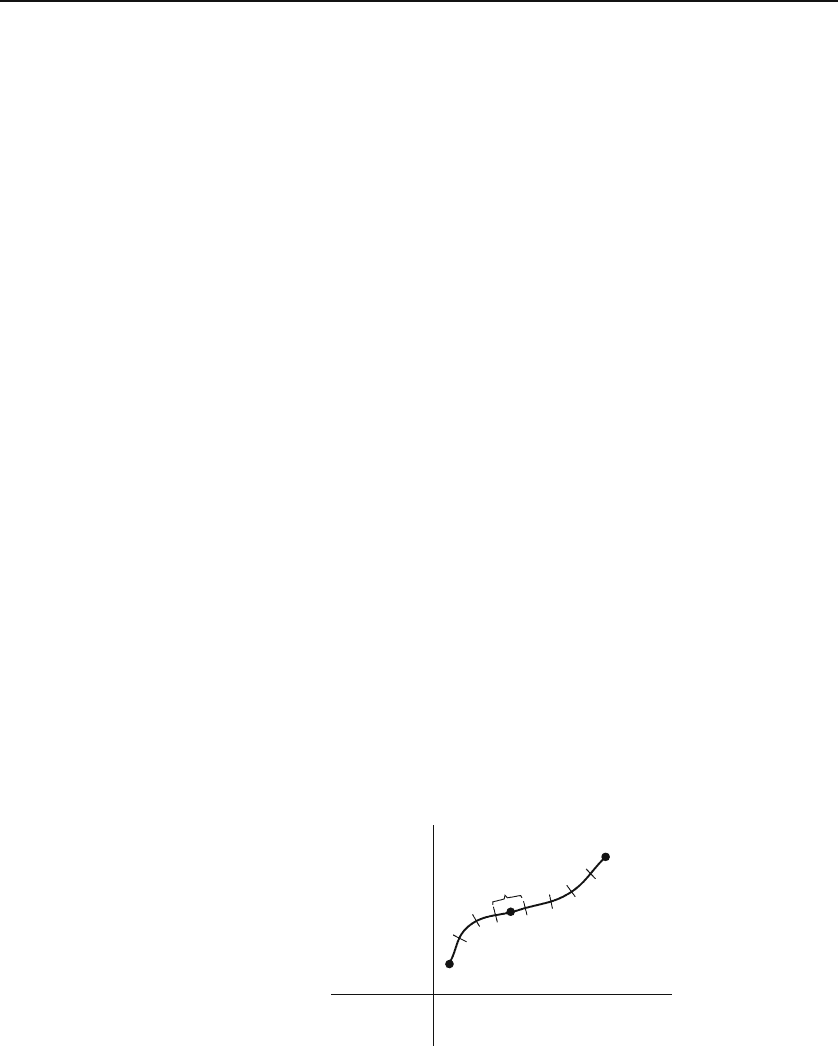
where Δz
i
is a small segment—situated at z
i
—of the curve that connects the
complex number α
1
to the complex number α
2
in the z-plane (see Figure 19.3).
An immediate consequence of this equation is
#
α
2
α
1
f(z) dz
= lim
N→∞
Δz
i
→0
N
i=1
f(z
i
)Δz
i
≤ lim
N→∞
Δz
i
→0
N
i=1
|f(z
i
)Δz
i
|
= lim
N→∞
Δz
i
→0
N
i=1
|f(z
i
)||Δz
i
| =
#
α
2
α
1
|f(z)||dz|, (19.8)
where we have used the triangle inequality as expressed in Equation (18.7).
Since there are infinitely many ways of connecting α
1
to α
2
, there is no
guarantee that Equation (19.7) has a unique value: It is possible to obtain
different values for the integral of some functions for different paths. It may
seem that we should avoid such functions and that they will have no use in
physical applications. Quite to the contrary, most functions encountered, will
not, in general, give the same result if we choose two completely arbitrary
paths in the complex plane. In fact, it turns out that the only complex
function that gives the same integral for any two arbitrary points connected
by any two arbitrary paths is the constant function. Because of the importance
of paths in complex integration, we need the following definition:
α
1
α
2
Δ
z
i
z
i
Figure 19.3: One of the infinitely many paths connecting two complex points α
1
and
α
2
.

19.1 Complex Functions 505
Box 19.1.2. A contour is a collection of connected smooth arcs. When
the beginning point of the first arc coincides with the end point of the last
one, the contour is said to be a simple closed contour (or just closed
contour).
We encountered path-dependent integrals when we tried to evaluate the
line integral of a vector field in Chapter 14. The same argument for path-
independence can be used to prove (see Problem 19.21)
Theorem 19.1.7. (Cauchy–Goursat Theorem).Letf(z) be analytic on
a simple closed contour C and at all points inside C.Then
2
C
f(z) dz =0
Equivalently,
α
2
α
1
f(z) dz is independent of the smooth path connecting α
1
and
α
2
as long as the path lies entirely in the region of analyticity of f (z).
Example 19.1.8.
We consider a few examples of definite integrals.
(a) Let us evaluate the integral I
1
=
γ
1
zdz where γ
1
is the straight line drawn
from the origin to the point (1, 2) (see Figure 19.4). Along such a line y =2x and
thus γ
1
(t)=t +2it where 0 ≤ t ≤ 1, and
3
I
1
=
#
γ
1
zdz=
#
1
0
(t +2it)(dt +2idt)=
#
1
0
(−3tdt +4itdt)=−
3
2
+2i.
For a different path γ
2
, along which y =2x
2
,wegetγ
2
(t)=t+2it
2
where 0 ≤ t ≤ 1,
and
I
1
=
#
γ
2
zdz=
#
1
0
(t +2it
2
)(dt +4itdt)=−
3
2
+2i.
Therefore, I
1
= I
1
. This is what is expected from the Cauchy–Goursat theorem
because the function f(z)=z is analytic on the two paths and in the region bounded
by them.
γ
1
γ
2
γ
3
x
y
(1, 2)
Figure 19.4: The three different paths of integration corresponding to the integrals I
1
,
I
1
, I
2
,andI
2
.
3
We are using the parameterization x = t, y =2x =2t for the curve.
506 Complex Derivative and Integral
(b) Now let us consider I
2
=
γ
1
z
2
dz with γ
1
as in part (a). Substituting for z in
terms of t,weobtain
I
2
=
#
γ
1
(t +2it)
2
(dt +2idt)=(1+2i)
3
#
1
0
t
2
dt = −
11
3
−
2
3
i.
Next we compare I
2
with I
2
=
γ
3
z
2
dz where γ
3
is as shown in Figure 19.4. This
path can be described by
γ
3
(t)=
0
t for 0 ≤ t ≤ 1,
1+i(t − 1) for 1 ≤ t ≤ 3.
Therefore,
I
2
=
#
1
0
t
2
dt +
#
3
1
[1 + i(t −1)]
2
(idt)=
1
3
− 4 −
2
3
i = −
11
3
−
2
3
i,
which is identical to I
2
, once again because the function is analytic on γ
1
and γ
3
as
well as in the region bounded by them.
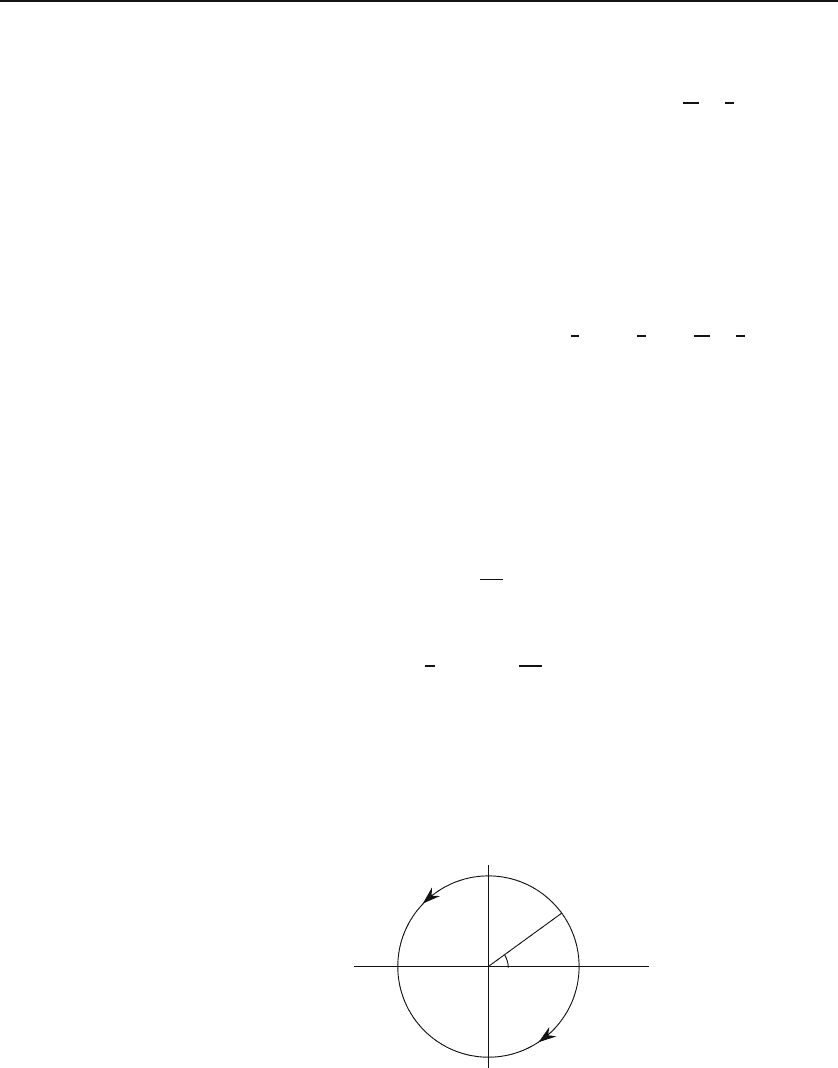
(c) An example of the case where equality for different paths is not attained is
I
3
=
γ
4
dz/z where γ
4
is the upper semicircle of unit radius, as shown in Figure 19.5.
A parametric equation for γ
4
can be given in terms of θ:
γ
4
(θ)=cosθ + i sin θ = e
iθ
⇒ dz = ie
iθ
dθ, 0 ≤ θ ≤ π.
Thus, we obtain
I
3
=
#
π
0
1
e
iθ
ie
iθ
dθ = iπ.
On the other hand,
I
3
=
#
γ
4
1
z
dz =
#
π
2π
.
1
e
iθ
ie
iθ
dθ = −iπ.
Here the two integrals are not equal. From γ
4
and γ
4
we can construct a counter-
clockwise simple closed contour C, along which the integral of f (z)=1/z becomes
E
C
dz/z = I
3
− I
3
=2iπ. That the integral is not zero is a consequence of the
fact that 1/z is not analytic at all points of the region bounded by the closed
contour C.
γ
4
′ γ
4
r = 1
θ
Figure 19.5: The two semicircular paths for calculating I
3
and I
3
.
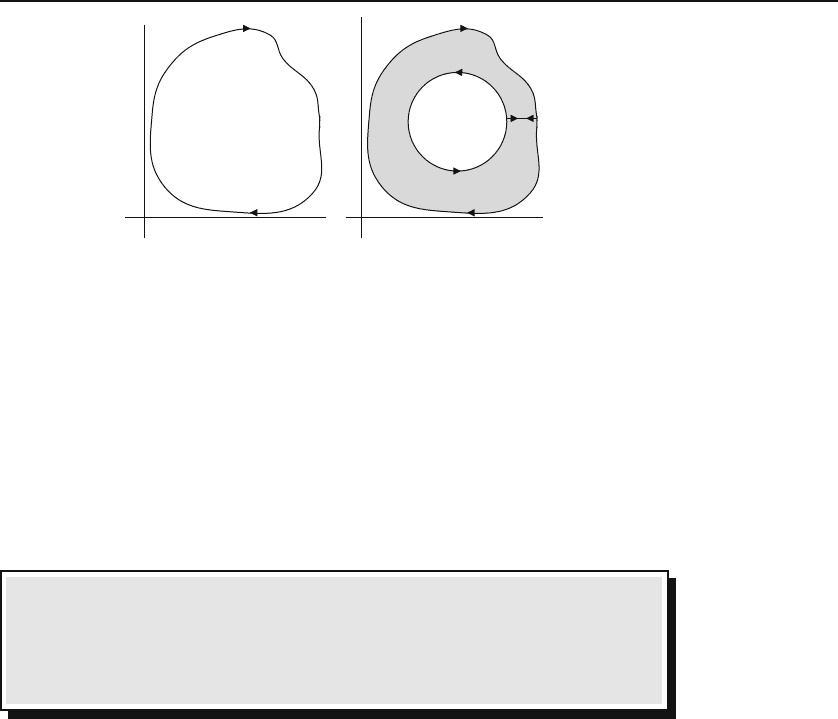
19.1 Complex Functions 507
C C
Γ
L
(a)
(b)
Figure 19.6: A contour of integration can be deformed into another contour. The
second contour is usually taken to be a circle because of the ease of its corresponding
integration. (a) shows the original contour, and (b) shows the two contours as well as
the (shaded) region between them in which the function is analytic.
The Cauchy–Goursat theorem applies to more complicated regions. When
a region contains points at which f(z) is not analytic, those points can be
avoided by redefining the region and the contour (Figure 19.6). Such a pro-
cedure requires a convention regarding the direction of “motion” along the
contour. This convention is important enough to be stated separately.
convention for
positive sense of
integration around
aclosedcontour
Box 19.1.3. (Convention). When integrating along a closed contour,
we agree to traverse the contour in such a way that the region enclosed
by the contour lies to our left. An integration that follows this convention
is called integration in the positive sense. Integration performed in the
opposite direction acquires a minus sign.
Suppose that we want to evaluate the integral
E
C
f(z) dz where C is some
contour in the complex plane [see Figure 19.6(a)]. Let Γ be another—usually
simpler, say a circle—contour which is either entirely inside or entirely out-
side C. Figure 19.6 illustrates the case where Γ is entirely inside C.We
assume that Γ is such that f (z) does not have any singularity in the region
between C and Γ. By connecting the two contours with a line as shown in
Figure 19.6(b), we construct a composite closed contour consisting of C,Γ,
and twice the line segment L, once in the positive directions and once in
the negative. Within this composite contour, the function f (z)isanalytic.
Therefore, by the Cauchy–Goursat theorem, we have
−
2
C
f(z) dz +
2
Γ
f(z) dz +
#
L
f(z) dz −
#
L
f(z) dz =0.
The negative sign for C is due to the convention above. It follows from this
equation that the integral along C is the same as that along the circle Γ. This
result can be interpreted by saying that
508 Complex Derivative and Integral
Box 19.1.4. We can always deform the contour of an integral in the com-
plex plane into a simpler contour, as long as in the process of deformation
we encounter no singularity of the function.
19.1.3 Cauchy Integral Formula
One extremely important consequence of the Cauchy–Goursat theorem, the
centerpiece of complex analysis, is the Cauchy integral formula which we state
without proof.
4
Theorem 19.1.9. Let f(z) be analytic on and within a simple closed contour
C integrated in the positive sense. Let z
0
be any interior point of C.Then
f(z
0
)=
1
2πi
2
C
f(z)
z − z
0
dz.
This is called the Cauchy integral formula (CIF).
Example 19.1.10.
We can use the CIF to evaluate the following integrals:
I
1
=
2
C
1
z
2
dz
(z
2
+3)
2
(z − i)
,I
2
=
2
C
2
(z
2
− 1) dz
(z −
1
2
)(z
2
− 4)
3
,
I
3
=
2
C
3
e
z/2
dz
(z − iπ)(z
2
− 20)
4
,
where C
1
, C
2
,andC
3
are circles centered at the origin with radii r
1
=
3
2
, r
2
=1,
and r
3
= 4, respectively.
For I
1
we note that f(z)=z
2
/(z
2
+3)
2
is analytic within and on C
1
,andz
0
= i
lies in the interior of C
1
.Thus,
I
1
=
2
C
1
f(z)dz
z − i
=2πif(i)=2πi
i
2
(i
2
+3)
2
= −i
π
2
.
Similarly, f(z)=(z
2
− 1)/(z
2
− 4)
3
for I
2
is analytic on and within C
2
,andz
0
=
1
2
is an interior point of C
2
.Thus,theCIFgives
I
2
=
2
C
2
f(z)dz
z −
1
2
=2πif(
1
2
)=2πi
1
4
− 1
(
1
4
− 4)
3
=
32π
1125
i.
For the last integral, f (z)=e
z/2
/(z
2
− 20)
4
, and the interior point is z
0
= iπ:
I
3
=
2
C
3
f(z)dz
z −iπ
=2πif(iπ)=2πi
e
iπ/2
(−π
2
− 20)
4
= −
2π
(π
2
+ 20)
4
.
The CIF gives the value of an analytic function at every point inside a
simple closed contour when it is given the value of the function only at points
on the contour. It seems as though analytic functions have no freedom within
why analytic
functions “remote
sense” their values
at distant points
a contour: They are not free to change inside a region once their value is
fixed on the contour enclosing that region. There is an analogous situation in
4
For a proof, see Hassani, S. Mathematical Physics: A Modern Introduction to Its Foun-
dations, Springer-Verlag, 1999, Chapter 9.
19.1 Complex Functions 509
certain areas of physics, for example, electrostatics: The specification of the
potential at the boundaries, such as conductors, automatically determines it
at any other point in the region of space bounded by the conductors. This is
the content of the uniqueness theorem (to be discussed later in this book) used
in electrostatic boundary-value problems. However, the electrostatic potential
Φ is bound by another condition, Laplace’s equation; and the combination of
Laplace’s equation and the boundary conditions furnishes the uniqueness of Φ.
It seems, on the other hand, as though the mere specification of an analytic
function on a contour, without any other condition, is sufficient to determine
the function’s value at all points enclosed within that contour. This is not
the case. An analytic function, by its very definition, satisfies another re-
strictive condition: Its real and imaginary parts separately satisfy Laplace’s
equation in two dimensions! [see Equation (19.4)]. Thus, it should come as
no surprise that the value of an analytic function at a boundary (contour)
determines the function at all points inside the boundary.
19.1.4 Derivatives as Integrals
The CIF is a very powerful tool for working with analytic functions. One
of the applications of this formula is in evaluating the derivatives of such
functions. It is convenient to change the dummy integration variable to ξ and
write the CIF as
f(z)=
1
2πi
2
C
f(ξ) dξ
ξ − z
, (19.9)
where C is a simple closed contour in the ξ-plane and z is a point within C.
By carrying the derivative inside the integral, we get
df
dz
=
1
2πi
d
dz
2
C
f(ξ) dξ
ξ −z
=
1
2πi
2
C
d
dz
f(ξ) dξ
ξ − z
=
1
2πi
2
C
f(ξ) dξ
(ξ − z)
2
.
By repeated differentiation, we can generalize this formula to the nth deriva-
tive, and obtain
Theorem 19.1.11. The derivatives of all orders of an analytic function f(z)
exist in the domain of analyticity of the function and are themselves analytic
in that domain. The nth derivative of f(z) is given by
f
(n)
(z)=
d
n
f
dz
n
=
n!
2πi
2
C
f(ξ) dξ
(ξ − z)
n+1
. (19.10)
Example 19.1.12.
Let us apply Equation (19.10) directly to some simple func-
tions. In all cases, we will assume that the contour is a circle of radius r centered
at z.
(a) Let f(z)=K, a constant. Then, for n =1wehave
df
dz
=
1
2πi
2
C
Kdξ
(ξ − z)
2
.
510 Complex Derivative and Integral
Since ξ is always on the circle C centered at z, ξ − z = re
iθ
and dξ = rie
iθ
dθ.So
we have
df
dz
=
1
2πi
#
2π
0
Kire
iθ
dθ
(re
iθ
)
2
=
K
2πr
#
2π
0
e
−iθ
dθ =0.
That is, the derivative of a constant is zero.
(b) Given f(z)=z, its first derivative will be
df
dz
=
1
2πi
2
C
ξdξ
(ξ −z)
2
=
1
2πi
#
2π
0
(z + re
iθ
)ire
iθ
dθ
(re
iθ
)
2
=
1
2π
z
r
#
2π
0
e
−iθ
dθ +
#
2π
0
dθ
=
1
2π
(0 + 2π)=1.
(c) Given f(z)=z
2
, for the first derivative Equation (19.10) yields
df
dz
=
1
2πi
2
C
ξ
2
dξ
(ξ − z)
2
=
1
2πi
#
2π
0
(z + re
iθ
)
2
ire
iθ
dθ
(re
iθ
)
2
=
1
2π
#
2π
0
*
z
2
+(re
iθ
)
2
+2zre
iθ
+
(re
iθ
)
−1
dθ
=
1
2π
z
2
r
#
2π
0
e
−iθ
dθ + r
#
2π
0
e
iθ
dθ +2z
#
2π
0
dθ
=2z.
Itcanbeshownthat,ingeneral,(d/dz)z
m
= mz
m−1
. The proof is left as Problem
19.24.
The CIF is a central formula in complex analysis. However, due to space
limitations, we cannot explore its full capability here. Nevertheless, one of its
applications is worth discussing at this point. Suppose that f is a bounded
entire function and consider
df
dz
=
1
2πi
2
C
f(ξ) dξ
(ξ − z)
2
.
Since f is analytic everywhere in the complex plane, the closed contour C can
be chosen to be a very large circle of radius R with center at z.Takingthe
absolute value of both sides yields
df
dz
=
1
2π
#
2π
0
f(z + Re
iθ
)
(Re
iθ
)
2
iRe
iθ
dθ
≤
1
2π
#
2π
0
|f(z + Re
iθ
)|
R
dθ ≤
1
2π
#
2π
0
M
R
dθ =
M
R
,
where we used Equation (19.8) and |e
iθ
| =1. M is the maximum of the
function in the complex plane.
5
Now as R →∞, the derivative goes to zero.
The only function whose derivative is zero is the constant function. Thus
Box 19.1.5. A bounded entire function is necessarily a constant.
5
M exists because f is assumed to be bounded.

19.2 Problems 511
There are many interesting and nontrivial real functions that are bounded and
have derivatives (of all orders) on the entire real line. For instance, e
−x
2
is such any nontrivial
function is either
unbounded or not
entire.
a function. No such freedom exists for complex analytic functions according
to Box 19.1.5! Any nontrivial analytic function is either not bounded (goes
to infinity somewhere on the complex plane) or not entire [it is not analytic
at some point(s) of the complex plane].
A consequence of Proposition 19.1.5 is the fundamental theorem of
algebra which states that any polynomial of degree n ≥ 1hasn roots (some
fundamental
theorem of algebra
proved
of which may be repeated). In other words, the polynomial
p(x)=a
0
+ a
1
x + ···+ a
n
x
n
for n ≥ 1
can be factored completely as p(x)=c(x −z
1
)(x − z
2
) ...(x − z
n
)wherec is
a constant and the z
i
are, in general, complex numbers.
To see how Proposition 19.1.5 implies the fundamental theorem of algebra,
we let f(z)=1/p(z) and assume the contrary, i.e., that p(z) is never zero for
any (finite) z.Thenf(z) is bounded and analytic for all z, and Proposition
19.1.5 says that f(z) is a constant. This is obviously wrong. Thus, there must
be at least one z,sayz = z
1
,forwhichp(z) is zero. So, we can factor out
(z −z
1
)fromp(z)andwritep(z)=(z −z
1
)q(z)whereq(z)isofdegreen −1.
Applying the above argument to q(z), we have p(z)=(z − z
1
)(z − z
2
)r(z)
where r(z)isofdegreen −2. Continuing in this way, we can factor p(z)into
linear factors. The last polynomial will be a constant (a polynomial of degree
zero) which we have denoted as c.
19.2 Problems
19.1. Show that f(z)=z
2
maps a line that makes an angle α with the
real axis of the z-plane onto a line in the w-plane which makes an angle
2α with the real axis of the w-plane. Hint: Use the trigonometric identity
tan 2α =2tanα/(1 −tan
2
α).
19.2. Show that the function w =1/z maps the straight line y =
1
2
in the
z-plane onto a circle in the w-plane.
19.3. (a) Using the chain rule, find ∂f/∂z
∗
and ∂f/∂z in terms of partial
derivatives with respect to x and y.
(b) Evaluate ∂f/∂z
∗
and ∂f/∂z assuming that the C–R conditions hold.
19.4. (a) Show that, when z is represented by polar coordinates, the C–R
conditions on a function f(z)are
∂U
∂r
=
1
r
∂V
∂θ
,
∂U
∂θ
= −r
∂V
∂r
,
where U and V are the real and imaginary parts of f(z) written in polar
coordinates.
512 Complex Derivative and Integral
(b) Show that the derivative of f can be written as
df
dz
= e
−iθ
∂U
∂r
+ i
∂V
∂r
.
Hint: Start with the C–R conditions in Cartesian coordinates and apply the
chain rule to them using x = r cos θ and y = r sin θ.
19.5. Prove the following identities for differentiation by finding the real
and imaginary parts of the function—u(x, y)andv(x, y)—and differentiat-
ing them:
(a)
d
dz
(f + g)=
df
dz
+
dg
dz
. (b)
d
dz
(fg)=
df
dz
g + f
dg
dz
.
(c)
d
dz
f
g
=
f
(z)g(z) − g
(z)f (z)
[g(z)]
2
, where g(z) =0.
19.6. Show that d/dz(ln z)=1/z.Hint:Findu(x, y)andv(x, y)forlnz
using the exponential representation of z, then differentiate them.
19.7. Show that sin z and cos z have only real roots. Hint: Use definition of
sine and cosine in terms of exponentials.
19.8. Use mathematical induction and the product rule for differentiation to
show that
d
dz
(z
n
)=nz
n−1
.
19.9. Use Equations (19.5) and (19.6), to establish the following identities:
(a) Re(sin z)=sinx cosh y, Im(sin z)=cosx sinh y.
(b) Re(cos z)=cosx cosh y, Im(cos z)=−sin x sinh y.
(c) Re(sinh z)=sinhx cos y, Im(sinh z)=coshx sin y.
(d) Re(cosh z)=coshx cos y, Im(cosh z)=sinhx sin y.
(e) |sin z|
2
=sin
2
x +sinh
2
y, |cos z|
2
=cos
2
x +sinh
2
y.
(f) |sinh z|
2
=sinh
2
x +sin
2
y, |cosh z|
2
=sinh
2
x +cos
2
y.
19.10. Find all the zeros of sinh z and cosh z.
19.11. Verify the following trigonometric identities:
(a) cos
2
z +sin
2
z =1.
(b) cos(z
1
+ z
2
)=cosz
1
cos z
2
− sin z
1
sin z
2
.
(c) sin(z
1
+ z
2
)=sinz
1
cos z
2
+cosz
1
sin z
2
.
(d) sin
π
2
− z
=cosz, cos
π
2
− z
=sinz.
(e) cos 2z =cos
2
z − sin
2
z, sin 2z =2sinz cos z.
(f) tan(z
1
+ z
2
)=
tan z
1
+tanz
2
1 − tan z
1
tan z
2
.
