Fujita S., Ito K., Godoy S. Quantum Theory of Conducting Matter

Подождите немного. Документ загружается.

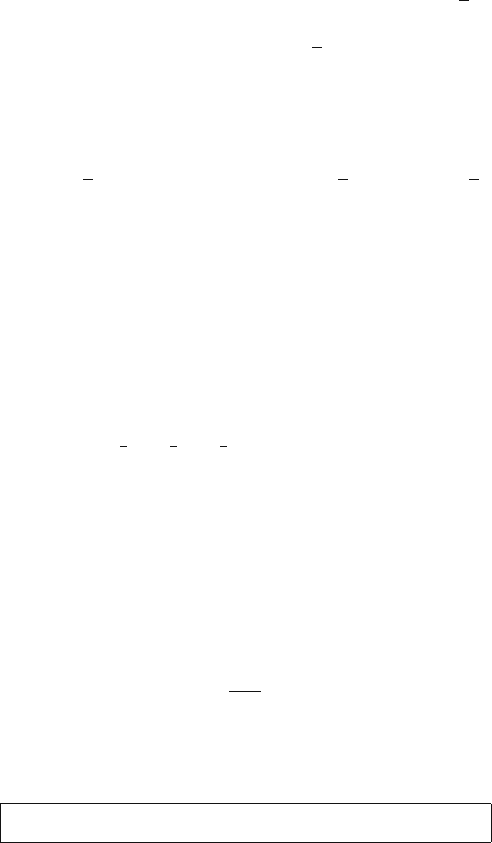
2.2 Electron–Phonon Interaction 21

We can express (q, p) in terms of (a

†

, a):

q =−i(/2ω)

1/2

†

−a), p = (ω/2)

1/2

†

+a). (2.28)

Thus, we may work entirely in terms of (a

†

, a). After straightforward calcula-

tions, we obtain (Problem 2.2.3)

ωa

†

a = (2)

−1

(p +iωq)(p −iωq)

= (2)

−1

+ω

+iω(qp − pq)] = H −

ω,

ω aa

†

= H +

ω, (2.29)

†

−a

†

a ≡ [a, a

†

] = 1, (2.30)

H =

ω(a

†

a + aa

†

) = ω



†

a +



≡ ω



n +



. (2.31)

The operators (a

†

, a) satisfy the Bose commutation rules, Equation (2.30). We

can therefore use second quantization, which is summarized in Appendix A, Sec-

tions A.1 and A.2, and obtain

• Eigenvalues of n ≡ a

†

a: n



= 0, 1, 2, ··· [see Equation (A.1)]

• Vacuum ket



: a



= 0 [see Equation (A.14)]

• Eigenkets of n:



, a

†



, (a

†

)



··· having the eigenvalues 0, 1, 2, ···

[see Equation (A.16)]

• Eigenvalues of H:

ω,

ω, ···

In summary, the quantum Hamiltonian and the quantum states of a harmonic os-

cillator can be simply described in terms of the bosonic second quantized operators

(a, a

†

We now go back to the case of the lattice normal modes. Each normal mode

corresponds to a harmonic oscillator characterized by (q,ω

). The displacements

can be expressed as

= i





2ω



1/2

−a

†

), (2.32)

where (a

, a

†

) are operators satisfying the Bose commutation rules:

, a

†

] ≡ a

†

−a

†

= δ

, [a

, a

] = [a

†

, a

†

] = 0. (2.33)

22 2 Electron–Phonon Interaction

We can express the electron density (ﬁeld) by

n(r) = ψ

†

(r)ψ(r), (2.34)

where ψ(r) and ψ

†

(r)areannihilation and creation electron ﬁeld operators, respec-

tively, satisfying the following Fermi anticommutation rules:



ψ(r),ψ

†



)



≡ ψ(r)ψ

†



) +ψ

†



)ψ(r) = δ

(3)

(r −r





ψ(r),ψ(r



)





†

(r),ψ

†



)



= 0. (2.35)

The ﬁeld operators ψ (ψ

†

) can be expanded in terms of the momentum-state

electron operators c

†

ψ(r) =

(V )

1/2



exp(ik ·r)c

,ψ

†

(r) =

(V )

1/2





exp(−ik



·r)c

†



, (2.36)

where operators c, c

†

satisfy the Fermi anticommutation rules:

, c

†



}≡c

†



†



= δ

(3)

k,k



, {c

, c



}={c

†

, c

†



}=0. (2.37)

Let us now construct an interaction Hamiltonian H

, which has the dimensions

of an energy and which is Hermitian. We propose







exp(iq ·r)ψ

†

(r)ψ(r) +h.c.



, (2.38)

where h.c. denotes the Hermitian conjugate. Using Equations (2.20), (2.32) and

(2.36), we can re-express Equation (2.38) as (Problem 2.2.3):



†

k+q

+h.c.), V

≡ A

(/2ω

)

1/2

iq. (2.39)

This is the Fr

ohlich Hamiltonian. Electrons describable in terms of c

are now

coupled with phonons describable in terms of a

.Theterm

†

k+q

∗

†

k+q

†

) (2.40)

canbe pictured as aninteraction processinwhich a phonon isabsorbed (emitted)byan

electron as represented by the Feynman diagram [8], [9] in Fig. 2.3 (a) [(b)]. Note: At

each vertex the momentum is conserved. The Fr

ohlich Hamiltonian H

is applicable

forthe longitudinalphononsonly.As notedearlier,the transverselattice normalmodes

generate no charge density variations, making the interaction negligible.

2.3 Phonon–Exchange Attraction 23

(a) (b)

Fig. 2.3 Feynman diagrams representing (a) absorption and (b) emission of a phonon by an

electron

Problem 2.2.1. Prove Equation (2.21).

Problem 2.2.2. Verify Equation (2.27).

Problem 2.2.3. Verify Equation (2.31).

Problem 2.2.4. Verify Equation (2.37).

2.3 Phonon–Exchange Attraction

By exchanging a phonon, a pair of electrons can gain attraction under a certain

condition. We treat this effect in this section by using the many-body perturbation

method [10], [11].

Let us consider an electron–phonon system characterized by

H =





†



ω



†



+λ





†

k+q s

+h.c.



≡ H

+ H

+λH

≡ H

+λV, (V ≡ H

) (2.41)

where the three sums represent: the total electron kinetic energy (H

), the total

phonon energy (H

), and the Fr

ohlich interaction Hamiltonian H

, [see Equation

(2.39)].

For comparison we consider an electron gas system characterized by the

Hamiltonian





†



···





3, 4 |v

|1, 2



†

≡ H

, (2.42)

24 2 Electron–Phonon Interaction



3, 4|v

|1, 2



≡



, k



4πe

−k

. (2.43)

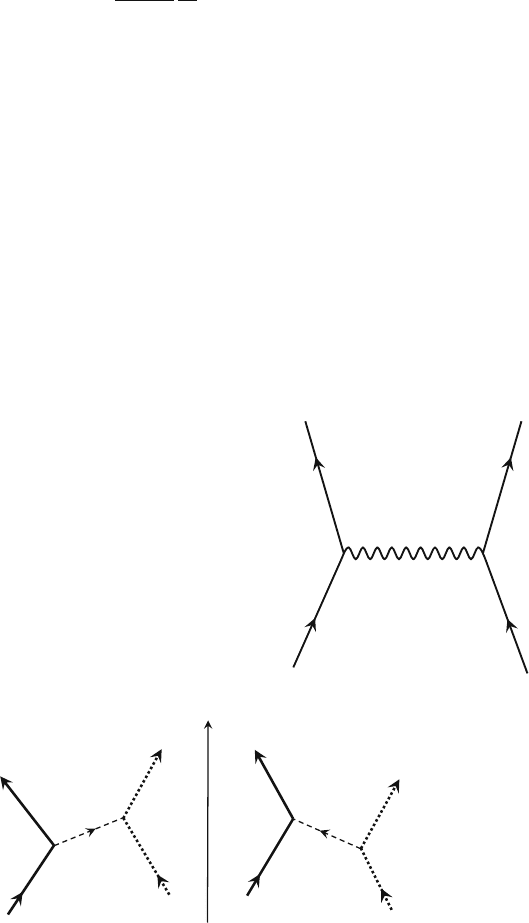
The elementary interaction process can be represented by the diagram in Fig. 2.4.

The wavy horizontal line represents the instantaneous Coulomb interaction v

.The

net momentum of a pair of electrons is conserved:

= k

, (2.44)

represented by the Kronecker’s delta in Equation (2.43). Physically, the Coulomb

force between a pair of electrons is an internal force, and hence it cannot change the

net momentum.

We wish to ﬁnd an effective Hamiltonian v

between a pair of electrons generated

by a phonon exchange. If we look for this v

in the second order in the coupling

constant λ, then the likely candidates are represented by two Feynman diagrams

in Fig. 2.5. Here, the time is measured upward. (Historically, Feynman represented

the elementary interaction processes by diagrams. Diagram representation is widely

Fig. 2.4 The Coulomb

interaction represented by the

horizontal wavy line

generates the change in the

mometa of two electrons

time

′

−

qk −

′

−

qk +

′

−

qk −

′

−

qk +

)a(

)b(

Fig. 2.5 A one-phonon–exchange process generates the change in the momenta of two electrons

similar to that caused by the Coulomb interaction

2.3 Phonon–Exchange Attraction 25

used in quantum ﬁeld theory [8], [9].) In the diagrams in Fig. 2.5, we follow the

motion of two electrons. We may therefore consider a system of two electrons and

obtain the effective Hamiltonian v

through a study of the evolution of two-body

density operator ρ

. Hereafter, we shall drop the subscript 2 on ρ indicating two-

body system.

The system-density operator ρ(t) changes in time, following the quantum Liou-

ville equation:

i

⭸ρ(t)

⭸t

= [H,ρ] ≡ Hρ. (2.45)

We assume the Hamiltonian H in Equation (2.41) and study the time evolution

of ρ(t), using quantum many-body perturbation theory. Here, we sketch only the

important steps; more detailed calculations were given in Fujita–Godoy’s book,

Quantum Statistical Theory of Superconductivity [10], [11].

Let us introduce a quantum Liouville operator

H ≡ H

+λV, (2.46)

which generates a commutator upon acting on ρ, see Equation (2.45). We assume

that the initial-density operator ρ

for the electron–phonon system can be factorized

= ρ

electron

phonon

, (2.47)

which is reasonable at 0 K, where there are no real phonons and only virtual phonons

are involved in the dynamical processes. We can then choose

phonon



, (2.48)

where



is the vacuum-state ket for phonons:



= 0 for any q. (2.49)

The phonon vacuum average will be denoted by an upper bar or by angular

brackets:

ρ(t) ≡



ρ(t)



≡



ρ(t)



. (2.50)

Using a time-dependent perturbation theory and taking a phonon-average, we

obtain from Equation (2.45)

⭸

ρ(t)

⭸t

=−





dτ



V exp(−iτ 

−1

)Vρ(t −τ )



. (2.51)

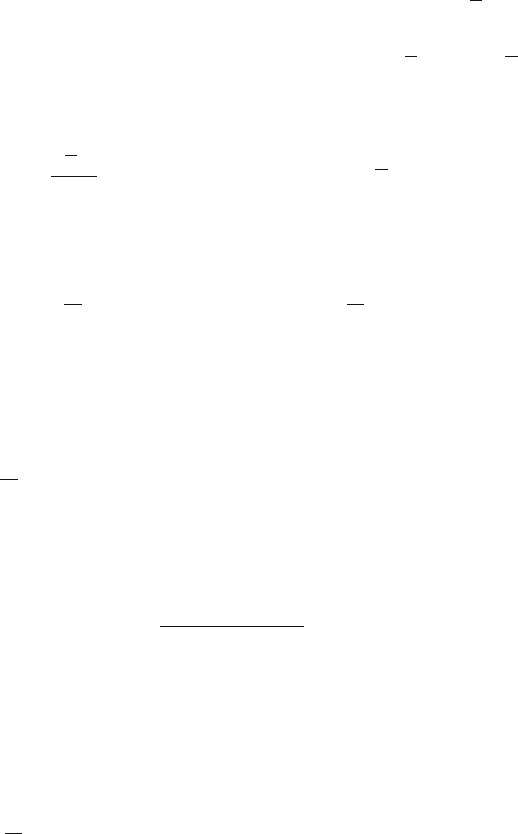
26 2 Electron–Phonon Interaction

In the weak-coupling approximation, we may calculate the phonon–exchange

effect to the lowest (second) order in λ and obtain



V exp(−iτ 

−1

)Vρ(t −τ )



= λ



V exp(−iτ 

−1



ρ(t − τ ). (2.52)

Using the Markofﬁan approximation we may replace

ρ(t − τ )byρ(t) and take

the upper limit t of the τ -integration to ∞. Using these two approximations, we

obtain from Equation (2.51)

⭸

ρ(t)

⭸t

= iλ



−1

lim

a→0



V(H

−ia)

−1



ρ(t), a > 0. (2.53)

Let us now take momentum-state matrix elements of Equation (2.53). The lhs is

⭸

⭸t



, k

|ρ(t) |k

, k



≡

⭸

⭸t

ρ(1, 2; 3, 4, t), (2.54)

where we dropped the upper bar indicating the phonon vacuum average. The rhs

requires more sophisticated computations due to the Liouville operators (V, H

After lengthy but straightforward calculations, we obtain from Equation (2.53)

⭸

⭸t

ρ(1, 2; 3, 4, t) =



−i

−1

[



1, 2 |v

|5, 6



(5, 6; 3, 4, t)

−



5, 6 |v

|3, 4



(1, 2; 5, 6, t)

]

, (2.55)



3, 4 |v

|1, 2



≡|V

ω

(

−

)

−

−k

. (2.56)

Kronecker’s delta δ

in Equation (2.56) means that the net momentum

is conserved, since the phonon exchange is an internal interaction.

For comparison, consider the electron-gas system. The two-electron density ma-

trix ρ

for this system changes, following

⭸

⭸t

(1, 2; 3, 4, t) =



−i

−1

[



1, 2 |v

|5, 6



(5, 6; 3, 4, t)

−



5, 6 |v

|3, 4



(1, 2; 5, 6, t)

]

, (2.57)

which is of the same form as Equation (2.55). The only differences are in the inter-

action matrix elements. Comparison between Equations (2.43) and (2.56) yields

References 27

4πe

(Coulomb interaction), (2.58)

ω

(

−

)

−

(phonon–exchange interaction). (2.59)

In our derivation, the weak-coupling and the Markofﬁan approximations were

used. The Markofﬁan approximation is justiﬁed in the steady state condition in

which the effect of the duration of interaction can be neglected. The electron mass is

four orders of magnitude smaller than the lattice-ion mass, and hence the coupling

between the electron and ionic motion must be small by the mass mismatch. Thus,

expression (2.59) is highly accurate for the effective phonon–exchange interaction

at 0 K. This expression has remarkable features. First, the interaction depends on

the phonon energy ω

. Second, the interaction depends on the electron energy

difference 

−

before and after the transition. Third, if

|

−

| < ω

, (2.60)

then the effective interaction is attractive. Fourth, the atraction is greatest when



− 

= 0, that is, when the phonon momentum q is parallel to the constant-

energy (Fermi) surface. A bound electron-pair, called a Cooper pair, may be formed

by the phonon–exchange attraction, which was shown in 1956 by Cooper [12].

References

1. T. W. B. Kibble, Classical Mechanics, (McGraw-Hill, Maidenhead, England, 1966), pp.

166–171.

2. C. B. Walker, Phys. Rev. 103, 547 (1956).

3. L. Van Hove, Phys. Rev. 89, 1189 (1953).

4. P. Debye, Ann. Physik 39, 789 (1912).

5. J. Bardeen, L. N. Cooper and J. R. Schrieffer, Phys. Rev. 108, 1175 (1957).

6. H. Fr

ohlich, Phys. Rev. 79, 845 (1950).

7. H. Fr

ohlich, Proc. R. Soc. London A 215, 291 (1950).

8. R. P. Feynman, Statistical Mechanics (Addison-Wesley, Reading, MA, 1972).

9. R. P. Feynman, Quantum Electrodynamics (Addison-Wesley, Reading, MA, 1961).

10. S. Fujita and S. Godoy, Quantum Statistical Theory of Superconductivity, (Plenum, New York,

1996), pp. 150–153.

11. S. Fujita and S. Godoy, Theory of High Temperature Superconductivity, (Kluwer, Dordrecht,

2001), pp. 54–58.

12. L. N. Cooper, Phys. Rev. 104, 1189 (1956).

Chapter 3

The BCS Ground State

We assume a generalized BCS Hamiltonian which contains the kinetic energies

of “electrons” and “holes”, and the pairing Hamiltonian arising from the phonon-

exchange attraction and the Coulomb repulsion. We follow the original BCS the-

ory to construct a many-pairon ground state and ﬁnd a ground state energy: W =

(1/2)N

+ w

), where N

is the total number of the pairons, and w

and

are respectively the ground state energies of “electron” ( j = 1) and “hole”

( j = 2) pairons. Energy gaps ⌬

are found in the quasi-electron excitation spectra:

( j)

≡ (

+⌬

)

1/2

3.1 Introduction

Lead (Pb) is a quadrivalent metal, which forms a fcc crystal, and it is a supercon-

ductor with the critical temperature T

= 7.19 K. Tin (Sn) is a trivalent fcc metal,

and it is a superconductor with T

= 3.72 K. We discussed the Fermi surface of

these metals in Book 1, Chapter 13. Both metals are known to have “electrons” and

“holes.” See Book 1, Sections 9.2, 13.3 and 13.4.

“Electrons”(“holes”) in the text are deﬁned as quasiparticles prossessing charge

e (magnitude) that circulate counterclockwise (clockwise) viewed from the tip of

the applied magnetic ﬁeld vector B. See Book 1, Section 10.2. This deﬁnition is

used routinely in semiconductor physics. We use quotation-marked “electron” to

distinguish it from the generic electron having the gravitational mass m

. A “hole”

can be regarded as a particle having positive charge, positive mass, and positive

energy. The “hole” does not, however, have the same effective mass m

∗

(magnitude)

as the “electron.” Hence “holes” are not true antiparticles like positrons. “Electrons”

and “holes” are the thermally excited particles near the Fermi surface depending on

the surface’s curvature sign. See Book 1, Section 10.2.

BCS deﬁned “electrons” and “holes” simply as quasiparticles above and below

the Fermi surface. This deﬁnition allows the presence of “electrons” and “holes”

for an ideal electron gas with a spherical Fermi surface. Hence, BCS’s and our

deﬁnitions are distinct from each other. Other differences will be pointed out later

in Sections 3.2 and 3.4.8.

S. Fujita et al., Quantum Theory of Conducting Matter,

DOI 10.1007/978-0-387-88211-6



Springer Science+Business Media, LLC 2009

30 3 The BCS Ground State

As we will see presently “electrons” and “holes” are necessary ingredients for

superconductivity. We shall discuss the ground state of a superconductor following

Bardeen, Cooper and Schrieffer [1] in this chapter.

3.2 The Reduced Hamiltonian

We ﬁrst review the BCS theory and construct a generalized BCS Hamiltonian, which

contains the kinetic energies of “electrons” and “holes,” and the pairing Hamiltonian

arising from the phonon–exchange attraction and the Coulomb repulsion. The the-

ory can be applied to a 2D system or a 3D system.

BCS assumed a Hamiltonian containing “electron” and “hole” kinetic energies

and a pairing interaction [1]. They also assumed a free-electron spherical Fermi

surface. However, if we assume a free electron model, we cannot explain why only

some, and not all, metals are superconductors. We must take the band structures of

electrons into consideration. In this section we set up and discuss a generalized BCS

Hamiltonian [2–6]. We make the following assumptions:

• In spite of the Coulomb interaction, there exists a sharp Fermi surface at 0 K for

the normal state of a conductor (the Fermi liquid model [3–9]).

• The phonon exchange attraction can generate Cooper pairs [10] near the Fermi

surface within a distance (energy) equal to Planck’s constant  times the Debye

frequency ω

• “Electrons” and “holes” with different effective masses (magnitude) are gener-

ated near the non-spherical Fermi surface, depending on the curvature sign.

• The pairing interaction strengths V

among and between “electron” (1) and

“hole” (2) pairons are given by



1, 2; i |U |3, 4; j





−V

−1

if |

| < ω

0 otherwise.

(3.1)

“Electrons” and “holes” are different quasiparticles, which will be denoted dis-

tinctly. Let us introduce the vacuum state



, creation and annihilation operators,

(1)†

, c

(1)

), and the number operators n

(1)

for “electrons” (

> 0):

(1)

≡ c

, c

(1)†

= c

†

, n

(1)

≡ c

(1)†

(1)

, c

(1)



= 0. (3.2)

For “holes” (

< 0), we introduce the vacuum state



, creation and annihila-

tion operators, (c

(2)†

, c

(2)

), and the number operators n

(2)

as follows:

(2)

≡ c

†

, c

(2)†

= c

, n

(2)

≡ c

(2)†

(2)

, c

(2)



= 0. (3.3)

Hereafter we adopt Dirac’s convention that a “hole” has a positive mass m

a positive energy 

(2)

≡|

| and the positive charge e. At 0 K there are only

3.2 The Reduced Hamiltonian 31

zero-momentum pairons of the lowest energy. The ground state ⌿ for the system

can then be described in terms of a reduced Hamiltonian:





(1)





(2)

−











(1)†

(1)



(1)†

(2)†



(2)

(1)



(2)

(2)†



. (3.4)

where v

≡ V

−1

, and b

( j)

are pair annihilation operators deﬁned by

(1)

≡ c

(1)

−k↓

(1)

k↑

, b

(2)

≡ c

(2)

k↑

(2)

−k↓

; (3.5)

the primes on the last summation symbols in Equation (3.4) indicate the restriction

that

0 <

(1)

≡ 

< ω

for “electrons”

0 <

(2)

≡|

| < ω

for “holes.” (3.6)

Let us drop the pairing Hamiltonian altogether in Equation (3.4). We then have

the ﬁrst two sums representing the kinetic energies of “electrons” and “holes.” These

energies (

(1)

,

(2)

) are positive by deﬁnition. The lowest energy of this system,

called the Bloch system, is zero, and the corresponding ground state is characterized

by zero “electrons” and zero “holes.” This state will be called the physical vacuum

state of the Bloch system. We shall look for the ground state of the generalized BCS

system whose energy is negative.

We now examine the physical meaning of the pairing interaction. Consider part

of the interaction terms in Equation (3.4):

−v

(1)†



(1)

, −v

(2)



(2)†

. (3.7)

The ﬁrst term generates a transition of the “electron” pair from (k ↑, −k ↓)

to (k



↑, −k



↓). This transition is represented by the k-space diagrams in Fig. 3.1.

Such a transition may be generated by the emission of a virtual phonon with momen-

tum q = k



− k (−q) by the down (up)-spin “electron” and subsequent absorption

by the up (down)-spin “electron” as shown in Fig. 3.1 (a) and (b). Note: These

two processes are distinct, but yield the same net transition. As we saw earlier in

Section 2.3 the phonon exchange generates an attractive correlation between two

“electrons” whose energies are nearly the same. The Coulomb interaction generates

a repulsive correlation. The effect of this interaction can be included in the strength

. Similarly, the exchange of a phonon induces a change of states between two

“holes,” and it is represented by the second term in Equation (3.7).

Fujita S., Ito K., Godoy S. Quantum Theory of Conducting Matter - Superconductivity

Подождите немного. Документ загружается.