Ferrarese G. (editor) Wave Propagation
Подождите немного. Документ загружается.


94
come to be known
as
the
inverse
scattering
method
in
the
context
of
sol
itons.
We
can do no more
here
than
outline
the
ideas
that
are
involved.
The
basic
problem
to
be
considered
is
how a
general
solution
of
the
KdV
equation
o
(27)
subject
to
arbitrary
initial
data
u(x,O)
• uO(x) may be
obtained.
The
factor
-6
is
included
here
for
convenience,
but
it
may
easily
be removed by a
trivial
transformation
if
required
.
In
essence,
the
approach
to
this
question
by Gardner
et
al
.
proceeded
as
follows
.
When
v
satisfies
the
u
modified
KdV
equation
v - 6v
2v
+ v •
0,
t x xxx
they
noticed
that
the
quantity
u which
is
given
by
v
2
+ v ,
x
satisfies
the
KdV
equation
u - 6uu + u •
O.
t x xxx
Equation
(29)
is
a
Riccati
equation
for
v
if
we
consider
u
to
be
given.
Therefore,
we can use
the
well
known
transformation
which
linearizes
the
Riccati
equation,
This
gives
(28)
(29)
(30)
(31)
o ,
(32)
where u
is
a
solution
of
the
KdV
equation
(30).
This
ia
a
natural
extension
of
the
Hopf-Cole
transformation
since
the
KdV
equation
has
a
third
order
space
derivative
and
it
is
one
order
higher
than
that
of
the
Burgers'
equation.
However,
if
we
merely
use
(32)
in
the
KdV
equation
we
obtain
a
complicated
result
that
is
not
useful
.
Now
the
KdV
equation
is
Galilean
invariant,
and
so
allows
the
rep
lacemen
ts
u ... u - )., x ... x + 6),
t,
so
tha
t (32) can be
generalised
to

95
(33)
This
is
simply
the
eigenvalue
problem
for
the
Schr6dinger
equation
for
ljJ
with
the
"potential"
u, where u
is
the
solution
we
are
seeking
.
Equation
(33)
differs
essentially
from
the
eigenvalue
problem
of
the
Scbr6dinger
equation
in
quantum
mechanics
because,
as
u must be a
solution
to
the
KdV
equation,
it
is
time-dependent.
That
is,
the
time
should
be
considered
as
a
parameter
in
(33).
In
other
words,
it
is
required
that
(33)
must
hold
at
every
instant
with
u(x,t)
at
that
same
instant.
Thus,
generally
speaking,
the
eigenvalues
A would be
expected
to
be
time-dependent.
Rather
surprisingly,
after
some
calculation,it
can be shown
that
they
are
time
independent
(and
constant),
provided
u
decreases
sufficiently
rapidly
at
infinity
.
Let
us deduce
the
relationship
between
the
KdV
equation
(30) and
the
Schrodinger
equation
(33) .
If
we
let
u
~
0
at
infinity,
the
KdV
equation
3
O~
gives
the
dispersion
relation
w + k •
0,
and
the
phase
velocity
A
p
becomes _k
2•
For
.suf f i c i ent l y
small
lui,
therefore,
we
have
a
plane
wave
propagating
in
the
negative
direction.
For
large
values
of
lui,
the
nonlinearity
dominates
to
give
rise
to
solitons.
The
linear
approximation
is
also
valid
at
infinity,
since
lui
becomes 0
at
infinity.
In
the
case
of
solitons,
the
wave
decreases
exponentially
at
infinity
and k becomes
purely
imaginary
with
k • i
A.
K 2 > 0 Thus a
soliton
K
p
' p
P •
propagates
in
the
positive
direction.
On
the
other
hand,
in
the
case
of
the
Schr6dinger
equation
(33),
it
follows
that
-ljJ
xx
~
AljJ
for
sufficiently
I I
±1kx 2
small
u,
and we
obtain
ljJ
~
e , A •
k.
This
approximation
is
still
valid
for
an
arbitrary
value
of
u,
provided
k
is
thought
to be
sufficiently
large.
2
For a bound
state,
the
eigenvalue
becomes A • -K
p
< 0,
i.e.
k • iK
p'
and ljJ
decreases
exponentially
at
infinity.
Therefore
we can deduce
that
one bound
state
corresponds
to
one
soliton.
In
fact,
if
we
substitute
the
soliton
solution

96
u • (34)
at
t • 0
into
(33) and
solve
the
eigenvalue
problem we can
get
only
the
bound
state
~
•
-K
2/
4•
P
Thus we can
see
that
the
eigenvalue
~
corresponds
to
the
speed
of
a
soliton
K 2.
If
the
"potential"
u<x,O)
(our
initial
data)
provides
N-bound
p
states,
the
solution
u(x,t)
as t + m
is
given
by
N-solitons
propagating
with
speeds
four
times
as
large
as
the
value
of
each
eigenvalue
and by
wavetrains
decreasing
algebraically
with
respect
to
time
. The
wavetrains
can
be
determined
in
relation
to
the
Bcattered
8tate
for
the
potential
u(x,O).
This
is
one
of
the
most
important
re.ult.
an4,
in
,eneral,
the
far
field
of
purely
dispersive
systems
for
which the I4V
aquacion
i.
cha
far
f1eld
equation
may
as t + m be
approximated
by
M-~~11ton.,
For a
given
arbitrary
valul
of
u(x,O),
u(x,c)
can be
obcained
exactly
by
the
following
procedure:
(1)
Direct
problem
Find
the
discrete
eigenValue
~
for
I
,ivan
pocenCial
u(x.O)
of
the
Schr6dinger
equation
(33) and
&1.0
find
the
.eatt.rin,
date
at
Ixl • m
for
the
wave
function
~
(i.e.,
the
refl.ction
or
tranam1s.1on
coefficients
for
u(x.O» .
(2)
Time
evolution
of
scattering
data
Find
the
time
evolution
of
the
scattering
data
and
the
asymptotic
form
of
~
at
Ixl • m .
(3)
Inverse
problem
Find
the
potential
u(x,t)
in
terms
of
the
scattering
data
at
time
t.
This
potential
is
then
the
exact
solution
to
the
KdV
equation
(30)
subject
to
the
arbitrary
initial
data
u(x.O)
• uO(x).
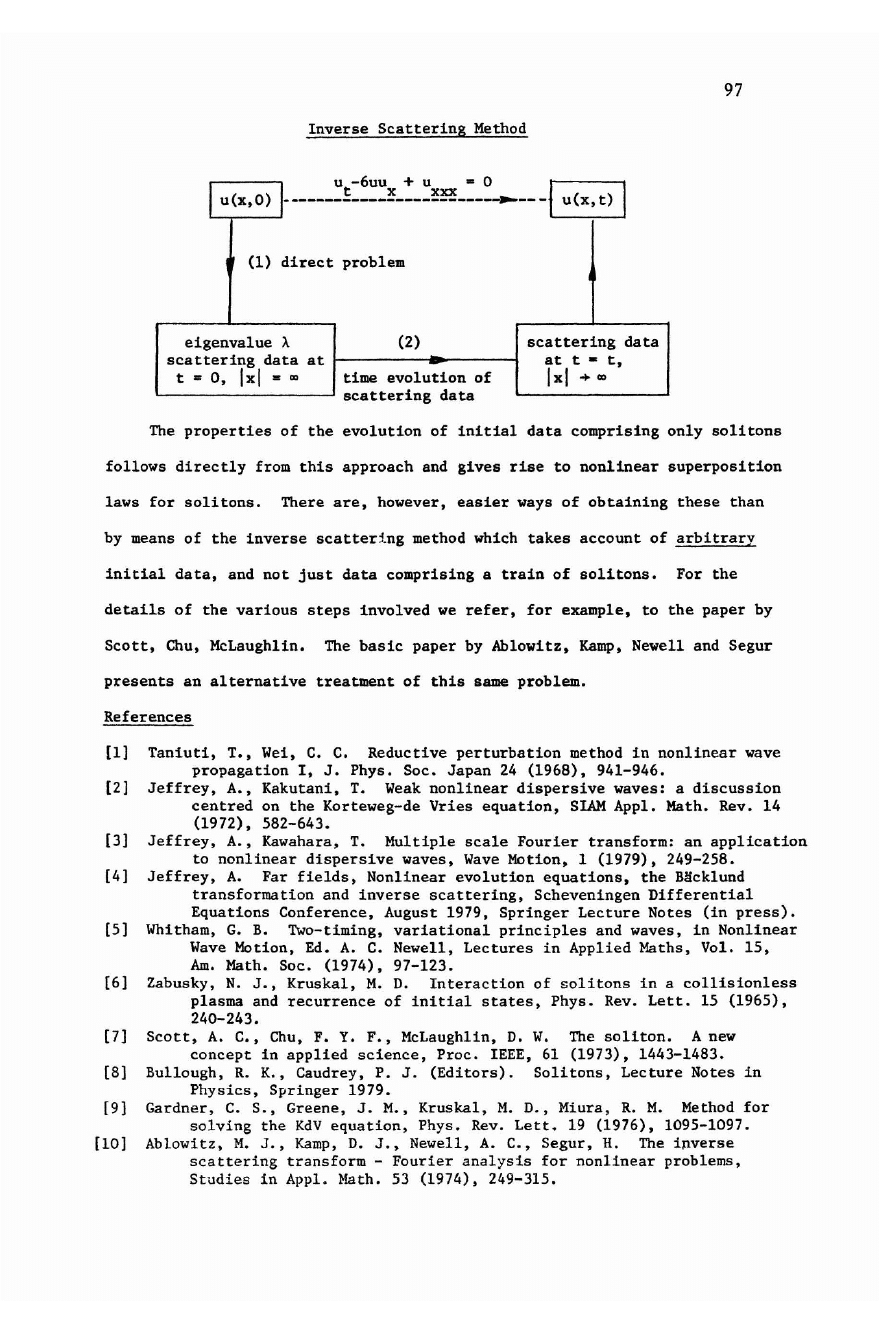
97
Inverse
Scattering
Method
g
u
-6uu
+ u '" 0
~
t x
xxx
I u
(x,
0)
1-
---------------------
------
- u
(x,
t)
(1)
direct
problem
eigenvalue
A (2)
scattering
data
scattering
data
at
at
t ..
t,
t ..
0,
Ixl
..
ell
time
evolution
of
Ixl
-+ ell
scatterin
data
The
properties
of
the
evolution
of
initial
data
comprising
only
solitons
follows
directly
from
this
approach
and
gives
rise
to
nonlinear
superposition
laws
for
solitons
.
There
are,
however,
easier
ways
of
obtaining
these
than
by means
of
the
inverse
scatter
ing
method which
takes
account
of
arbitrary
initial
data,
and
not
just
data
comprising
a
train
of
solitons.
For
the
details
of
the
various
steps
involved
we
refer,
for
example,
to
the
paper
by
Scott,
Chu,
Mclaughlin.
The
basic
paper
by
Ablowitz,
Kamp,
Newell and
Segur
presents
an
alternative
treatment
of
this
same
problem.
References
[1)
Taniuti,
T.,
Wei, C. C.
Reductive
perturbation
method
in
nonlinear
wave
propagat
ion
I,
J.
Phys. Soc.
Japan
24
(1968),
941-946.
[2)
Jeffrey,
A.,
Kakutani,
T. Weak
nonlinear
dispersive
waves: a
discussion
centred
on
the
Korteweg-de
Vries
equation,
SIAM
Appl. Math. Rev. 14
(1972),
582-643.
[3)
Jeffrey
, A. , Kawahara, T.
Multiple
scale
Fourier
transform:
an
application
to
nonlinear
dispersive
waves,
Wave
Motion, 1
(1979),
249-258.
[4)
Jeffrey,
A.
Far
fields,
Nonlinear
evolution
equations,
the
B~cklund
transformation
and
inverse
scattering,
Scheveningen
Differential
Equations
Conference,
August 1979,
Springer
Lecture
Notes
(in
press).
(5) Whitham, G. B. Two-timing,
variational
principles
and waves ,
in
Nonlinear
Wave Motion, Ed. A. C.
Newell,
Lectures
in
Applied
Maths, Vol.
15,
Am
. Math. Soc. (1974) , 97
-123.
[6) Zabusky, N.
J.,
Kruskal,
M.
D.
Interaction
of
solitons
in
a
collisionless
plasma and
recurrence
of
initial
states,
Phys.
Rev.
Lett.
15
(1965),
240-243.
(7)
Scott,
A.
C.,
Chu, F. Y. F
.,
McLaughlin, D.
W.
The
soliton.
A new
concept
in
applied
science,
Proc.
IEEE, 61
(1973),
1443-1483.
[8) Bu11ough, R. K., Caudrey, P.
J.
(Editors).
Solitons,
Lecture
Notes
in
Physics,
Springer
1979 .
[9)
Gardner,
C.
S.,
Greene, J . M.,
Kruskal,
M.
D.,
Miura,
R. M. Method
for
solving
the
KdV
equation,
Ph
ys.
Rev.
Lett.
19
(1976),
1095-1097.
(10)
Ab1ow
itz.
M.
J.,
Kamp,
D. J
.,
Newell,
A.
C.,
Segur,
H. The
inverse
scattering
transform
-
Fourier
anal
ysis
for
nonlinear
problems,
Stud
ies
in
App1
. Math. 53
(1974),
249-315.

CENTRO
INTERNAZIONALE
MATEMATICO
ESTIVO
(C.I.M.E.)
ONDES
ASYMPTOTIQUES
YVONNE
CHOQUET-BRUHAT

101
ONDES
ASYHPTOTIQUES.
Yvonne
Choquet-Bruhat.
I.M.T.A.
Universite
Paris
VI
Dedie a
la
memoire de
Carlo
Cattaneo.
Introduction.
Nous
allons,
dans
ces
le~ons,
exposer
les
grandes
lignes
de
la
methode
ge-
nerale
de
construction
des ondes
asymptotiques
et
approchees
pour
les
syste-
mes
d'equations
aux
derivees
partielles.
Le
procede
utilise
a son
origine
dans
la
methode W.K.B (Wentzel-Kramers
-Br
illouin)
consi
stant
a
chercher
pour
une
equation
differentielle
une
solution
de
la
forme Ae
i
¢ ou A
est
une
ampli-
tude
lentement
variable
et
¢ une
phase
rapidement
oscillatoire.
Dans
la
premiere
le~on
nous
exposerons
la
construction
de sol ut i ons
des
equations
de Maxwell
qui
donn
ent,
en
premiere
approximation,
les
lois
de
l'optiqu
e
geometriques.
Dans
le
s
le
~ons
II
et
III
nous expo
se
rons ,
dans
le

cas
d'un
systeme
du
le~
ordre,
la
theorie
genera1e
de
J.
Leray
des
deve
loppe-
ments
as)~ptotiques
pour
les
equations
lineaires,
dans
la
1e~on
IV nous mon-
trerons
comment
les
resultats
sont
modifies
quand
les
equations
ne
sont
pas
1ineaires,
et
nous
donnerons
dans
1a
l
e ~
o
n
V une
application
aux ondes
dans
les
f1uides
parfaits
re1ativistes.
Dans
la
le~on
VI nous
formulerons
en termes de
geometrie
symplect
ique
les
constructions
effectuees;
dans
1es
1e~ons
VII
et
VIII
nous
etudierons
les
pa-
rametrisations
des
varietes
1agrangiennes
et
nous
construirons
un
developpe-
ment
asymptotique
au
voisinage
d'une
caustique.

103
I
LES
EQUATIONS
DE
MAXWELL
ET
L'OPTIQUE
GEOHETRlQUE.
Les
solut
ions
asymptotiques
dont
nous
allons
parler
dans
ces
conferences
ont
leur
origine
dans
les
travaux
de Debye, Sommerfeld
et
Runge
pour
retrou-
ver
les
lois
de
l'optique
geometrique
a
partir
de
la
theorie
electromagneti-
que.
On a
montre
depuis
que
cette
methode de
solution
s'appliquait
a beaucoup
d'autres
prob
lemes
et
permettait
d'obtenir
des
renseignements,
tant
qualita-
tifs
que
quantitatifs
sur
des
phenomenes
physiques
varies
rapidement
oscil-
lants.
Pour
justifier
l'introduction
des
developpements
asymptotiques
generaux
que nous
etudierons
par
la
suite
nous
allons
considerer
d'abord
Ie
cas
origi-
nal
des
equations
de Maxwell.
1 -
Milieux
isotropes
non
conducteurs.
Les
equations
de Maxwell
verifiees
par
Ie
champ
electrique
E
et
Ie
champ ma-
gnetique
H
dans
un
dielectrique
parfait
homogene
s'ecrivent
:
(1-1)
(1-2)
rot
H-
aE
.
j
€
~
rot
E +
~
aH
.
~
0

104
ou j
est
Ie
vecteur
courant
e1ectrique;
€
et
~
sont
des
constantes.
Prenons
pour
j
Ie
courant
produit
par
un
dipole
situe
a
l'origine,
de
fre-
quence w.
Alors
j
s'ecrit,
en
representation
complexe
(Ie
courant
reel
est
.1t
e
j)
OU
o(x)
est
1a
mesure
de
Dirac
a
l'origine
(de
l'espace
m
3
)
et
Mun
vecteur
donne de
(3(si
M
est
reel,
Ie
dipole
est
dit
polarise
rectilignement).
Si
on
cherche
un regime
"stationnaire",
solution
des
equations
de Maxwell,
c'est-a-dire
de 1a forme, en
representation
complexe
E •
u(x)
itut
e
H •
v(x)
iwt
e
3
xE.1R ,
t~\R.
on
trouve
que
les
vecteurs
complexes u
et
v
doivent
verifier
1es
equations
(1-3)
(1-4)
rot
v - i e III u • M 0
rot
u + i u III V • 0
on
introduit
Ie
potentie1
vecteur
A en
posant
v »
rot
A
d I
apres
(1-4)
,
div
v •
0),
alors
u
est
de 1a forme,
d'apres
(J-4)
u - - i jJ III A +
grad
V
Vest
appe1e
potentiel
sca1aire.
On
Ie
choisit
en
lui
imposant
la
relation
dite
"condition
de
jauge
de
Lorentz"
(1-5
)
alors
(
1-5
(1-6)
)
devient
V • _._J_
div
A
~W€
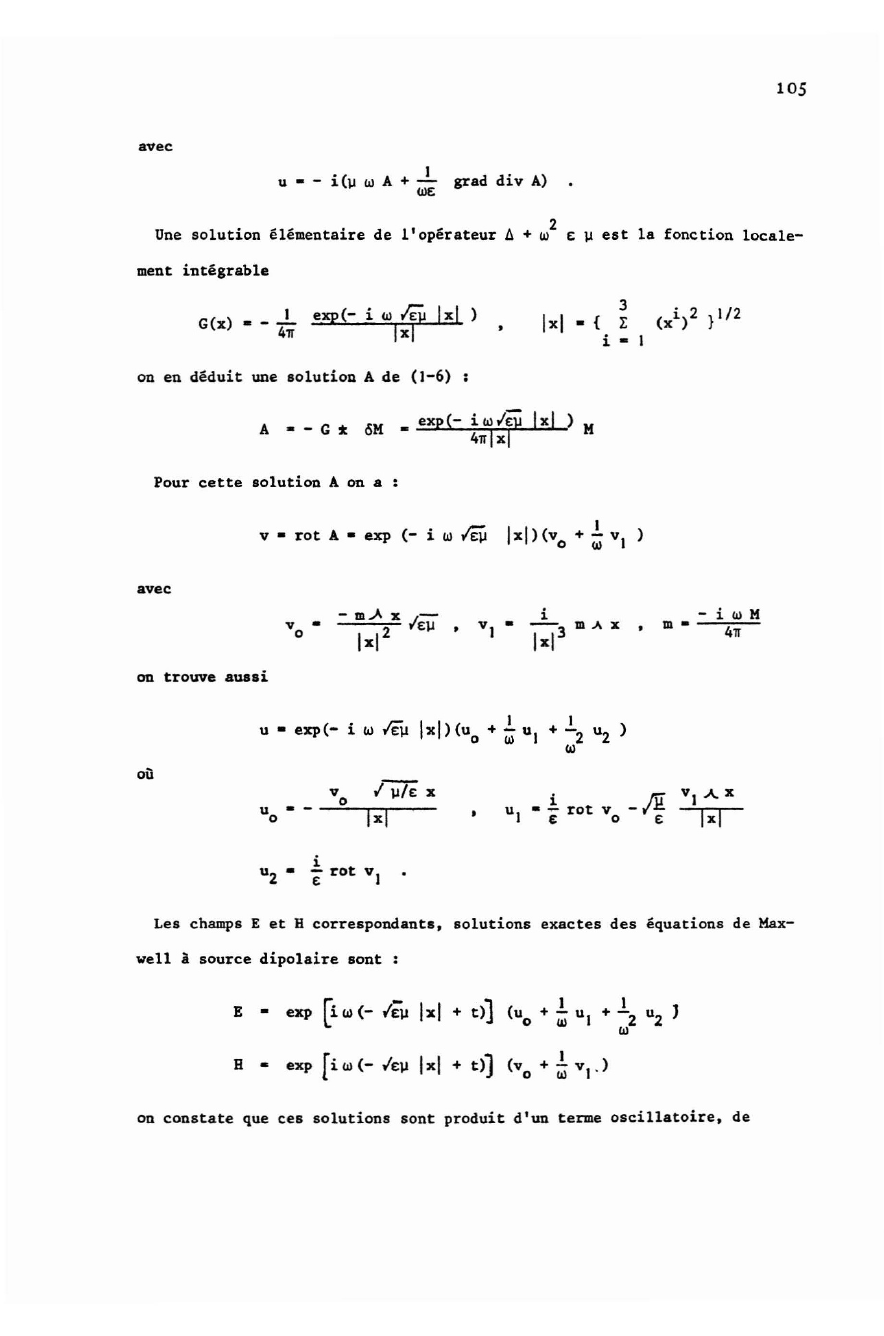
105
avec
u • -
i(~
w A + JL grad
div
A)
WE
2
Une
solution
elementaire
de
l'operateur
A+ W E
~
est
la
fonction
locale-
ment
integrable
I
exp(-
i w
lEU
Ixl
G(x)
• -
4n
Ixl
on en
deduit
une
solution
A de (1-6)
3
Ixl • { E
i •
A • -
G:t
oM •
exp(-
iw,/£ji
Ixl ) M
4nlxl
Pour
cette
solution
A on a
v •
rot
A • exp
(-
i w
~
avec
on
trouve
aussi
v •
o
- m/<. x
,-
2 yEll
Ixl
v •
1
i
~3
mAX
m·
i w H
4n
ou
v
.,
~/£
X
o
Ixl
.
Iii
V1AX
u
.!.
rot
v - {l!.
I £ 0 £ Ixl
Les champs
E
et
H
correspondants.
solutions
exactes
des
equations
de Max-
well
a
source
dipolaire
sont
:
r c: I
I'
1 +.!. }
E • exp
Liw(-
veu X +
t)J
(u
o
+;;;
u
1
w
2
u
2
H • exp
[iw(-"£~
Ixl
+
t»)
(v
o
+~vl
')
on
constate
que
ces
solutions
sont
produit
d'un
terme
oscillatoire.
de
