Doktorov E.V., Leble S.B. A Dressing Method in Mathematical Physics
Подождите немного. Документ загружается.

8.4 Ablowitz–Ladik equation 249
and [J
+
(n, z)]
11
does not depend on z in this limit. Hence, the column J
[1]
+
is analytic for |z| < 1, i.e., inside the unit circle at the z-plane. In the same
way we can show that the columns J
[1]
−
and J
[2]
+
areanalyticfor|z| > 1, i.e.,
outside the unit ci rcle. Let us define a matrix function
Φ
+
(n, z)=
!
J
[1]
−
,J
[2]
+
"
which is analytic for |z| > 1 (the C
out
domain) and solves the spectral equation
(8.55). It follows from the conjugation formulas (8.60) and (8.61) that the rows
(J
−
)
−1
[1]
and (J
+
)
−1
[2]
are analytic for |z| < 1 (the C
in
domain). As a result, the
matrix function
Φ
−1
−
(n, z)=
(J
−
)
−1
[1]
(J
+
)
−1
[2]
(n, z) (8.64)
is analytic as a whole in C
in
and solves the adjoint spectral problem. From
the definition (8.57) of the scattering matrix we have
Φ
+
=(J
[1]
−
,J
[2]
+
)=(a
+
J
[1]
+
+ z
−2n
J
[2]
+
,J
[2]
+
)=J
+
E
n
S
+
E
−n
,
S
+
=
a
+
0
b
+
1
,
and similarly
Φ
+
= J
−
E
n
S
−
E
−n
,S
−
=
1 b
−
/v
0 a
+
/v
.
Therefore,
det Φ
+
=detJ
+
det S
+
=detJ
−
det S
−
= v
+
(n) a
+
(z). (8.65)
Analogously,
Φ
−1
−
= E
n
T
+
E
−n
J
−1
+
= E
n
T
−
E
−n
J
−1
−
,
T
+
=
a
−
/v b
−
/v
01
,T
−
=
10
b
+
a
−
,
and
det Φ
−1
−
(n, z)=v
−1
−
(n)a
−
(z).
Asymptotic formulas for analytic solutions are derived directly from those
for the Jost functions. In particular, at z →∞
Φ
+
=
J
−11
J
+12
J
−21
J
+22
−→
lim
z→∞
J
−11
0
0 lim
z→∞
J
+22
.
From (8.62) J
(0)
−11
(n +1)=J
(0)
−11
(n)=J
(0)
−11
(n − 1) = ... = 11and
J
(0)
+22
(n)=ρ
−1
n
J
(0)
+22
(n +1)=ρ
−1
n
ρ
−1
n+1
J
(0)
+22
(n +2)=...=
∞
l=n
ρ
−1
l
= v
+
(n).
250 8 Dressing via local Riemann–Hilbert problem
Therefore, at z →∞
Φ
+
(n, z) −→ Φ
(0)
+
(n)=
10
0 v
+
(n)
. (8.66)
In the same way we derive at z → 0
Φ
−1
−
−→ Φ
−1
−(0)
=
v
−1
−
(n)0
01
.
Hence, det Φ
+
→ v
+
(n)atz →∞, which gives from (8.65) a
+
(z) → 1inthe
same limit. Similarly, a
−
(z) → 1atz → 0.
The last thing we should do with the analytic solutions is to obtain a
conjugation formula for them. Writing out (8.60) J
−1
±
(n, z)=v
−1
±
(n) J
†
±
(n, ¯z)
in components, we get
J
−1
−
(n, z)
11
= v
−1
−
J
†
−
(n, ¯z)
11
= v
−1
−
J
∗
−
(n, ¯z)
11
,
J
−1
−
(n, z)
12
= v
−1
−
J
†
−
(n, ¯z)
12
= v
−1
−
J
∗
−
(n, ¯z)
21
,
J
−1
+
(n, z)
21
= v
−1
+
(n)
J
∗
+
(n, ¯z)
12
,
J
−1
+
(n, z)
22
= v
−1
+
(n)
J
∗
+
(n, ¯z)
22
.
Inserting these relations into (8.64) yields
Φ
−1
−
(n, z)=
J
−1
−[1]
(n, z)
J
−1
+[2]
(n, z)
=
J
−1
−11
J
−1
−12
J
−1
+21
J
−1
+22
(n, z)
=
v
−1
−
J
∗
−11
v
−1
−
J
∗
−21
v
−1
+
J
∗
+12
v
−1
+
J
∗
+22
(n, ¯z)=
v
−1
−
0
0 v
−1
+
!
J
[1]
−
,J
[2]
+
"
†
(n, ¯z)
=
v
−1
−
0
0 v
−1
+
Φ
†
+
(n, ¯z).
As a result, the conjugation formula for the analytic solutions takes the form
Φ
†
+
(n, z)=B(n) Φ
−1
−
(n, ¯z),B(n)=
v
−
(n)0
0 v
+
(n)
. (8.67)
8.4.3 RH problem
As might be expected, the functions Φ
+
and Φ
−1
−
enter the RH problem
Φ
−1
−
(n, z)Φ
+
(n, z)=E
n
G(z)E
−n
, |z| =1, (8.68)
G = T
+
S
+
= T
−
S
−
=
1 b
−
/v
b
+
1
,
with the contour being the unit circle |z| = 1. The normalization of the RH
problem is noncanonical and is given by (8.66). It should be noted that there
8.4 Ablowitz–Ladik equation 251
exists a possibility for the AL equation to formulate the RH problem with
the standard normalization but at the cost of nonlinear dependence of the
spectral problem on the potential Q
n
[182].
As we know, solito ns correspond to the RH problem with zeros. Suppose
Φ
+
has zeros at some points ±z
j
∈ C
out
, j =1,...,N, i.e., det Φ
+
(±z
j
)=0,
and det Φ
−1
−
(±¯z
l
)=0,±¯z
l
∈ C
in
, l =1,...,N. Because of the modifi cation
of the involution property, we should match a definition of the projector P
j
with the conjugation formula (8.67). In other words, we define the projector
as
P
j
=
|jj|B
j|B|j
,
with the matrix B defined in (8.67). Owing to the parity property (8.58), we
have |−j = σ
3
|j.
Exactly as for the MNLS equation, we bring the matrix functions Φ
±
to a
factorizable representation Φ
±
= φ
±
Γ , with the dressing factor Γ written as
Γ (n, z)=11 −
N
j,l
1
z − ¯z
l
|j
D
−1
jl
l|B, (8.69)
Γ
−1
(n, z)=11+
N
j,l
1
z − z
j
|j
D
−1
jl
l|B,
with the matrix elements
D
lj
= l|
B
z
j
− ¯z
l
|j. (8.70)
Now what about a coordinate dependence of the vector |j? As regards
n-depend ence, we have
Φ
+
(n +1,z)|j, n +1 =0=[E(z
j
)+Q
n
] Φ
+
(n, z
j
)E
−1
(z
j
)|j, n +1.
Comparing this with the spectral equation (8.55), we can take E
−1
(z
j
)|j,
n +1 = |j, n,or
|j, n = E
n
(z
j
)|
˜
j,
where |
˜
j is an n-independent (but t-dependent) vector. It follows from (8.56)
that |j, n
t
= Ω(z
j
)|j, n. Therefore, the coordinate dependence of the vector
|j is given by
|j = E
n
(z
j
)e
Ω(z
j
)
|j
0
, |j
0
=const. (8.71)
Finally, we find from the identity det Φ
+
(z
j
) = 0 that zeros do not depend on
n and t.Zeros±z
j
and ±¯z
l
and vectors |j comprise the discrete part of the
RH problem. Once again, where solitons were concerned, i.e., G(z)=11, we
can cho ose the leading-order term Φ
(0)
+
(8.66) of the asymptotic expansion as
a solution of the regular RH problem φ
−1
−
φ
+
= 11.

252 8 Dressing via local Riemann–Hilbert problem
In accordance with (8.63), (8.66), and Φ
+
= φ
+
Γ ,asolutionu
n
(t)ofthe
AL equation can be retrieved from the solution of the RH problem as
u
n
(t)=− lim
z→∞
(zΦ
+
)
12
Φ
+22
= −
Φ
(1)
+12
Φ
(0)
+22
= −
Γ
(1)
12
v
+
(n)
. (8.72)
Hence, to obtain a soliton solution of the AL equation, we should find the
dressing factor Γ .
8.4.4 Ablowitz–Ladik soliton
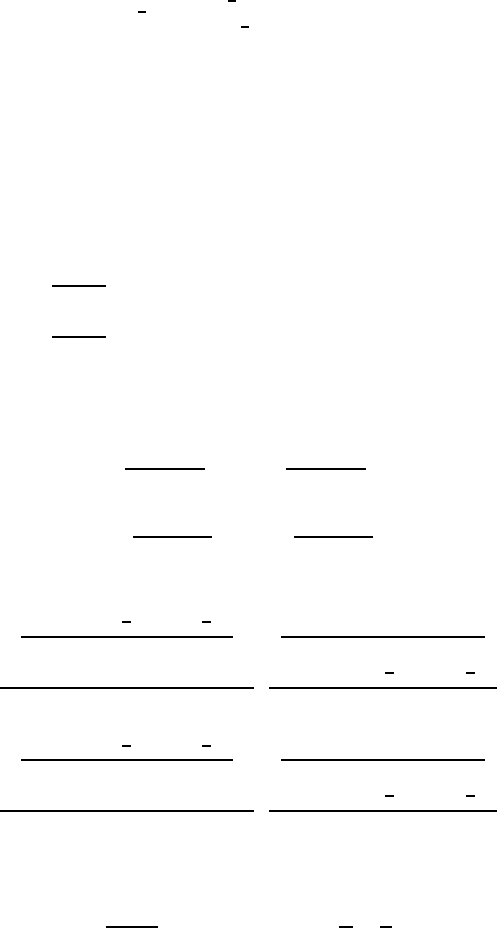
As we know, four zeros ±z
1
and ±¯z
1
correspond to a single soliton solution
of the AL equation (Fig. 8.5). To obtain the AL soliton, we will be guided by
(8.72). For discrete RH data, it is possible to express v
+
(n) entering (8.72)
in terms of Γ (n, z = 0 )—just in the same way as we expressed Φ
(0)
+
through
Γ (k =0)inthecaseoftheMNLSequation. Indeed, because for solitons
G(z)=11, we have Φ
+
= Φ
−
. Hence, Φ
+
→ diag(v
−
(n), 1) as z → 0, owing to
(8.66). Now we obtain from Φ
+
= Φ
(0)
+
Γ
Γ (n, z)=
10
0 v
−1
+
Φ
+
,Γ(n, 0) =
10
0 v
−1
+
v
−
0
01
=
v
−
0
0 v
−1
+
.
Hence, v
−1
+
(n)=Γ
22
(n, 0) and the reconstruction formula takes the form
u
n
(t)=−Γ
(1)
12
(n)Γ
22
(n, 0). (8.73)
Besides, we can express the matrix B (8.67) in terms of Γ (n, 0):
B =diag
Γ
11
(n, 0),Γ
−1
22
(n, 0)
.
Z
Z
1
Z
1
-Z
1
-Z
1
1
Fig. 8.5. Typical arrangement of zeros corresponding to the A blowitz–Ladik soliton
8.4 Ablowitz–Ladik equation 253
Now we will calculate the dressing factor Γ .Denotingz
1
=exp[(1/2)(μ +ik)]
and (p
1
/p
2
)=exp(a +iϕ), where p
1
and p
2
are components of the constant
vector |j
0
, we find from (8.71) the vector |j explicitly:
|j =e
1
2
(a+iβ)
e
1
2
(x
n
+iϕ
n
)
e
−
1
2
(x
n
+iϕ
n
)
. (8.74)
Here
x
n
= μn − 2t sinh μ sin k + a, ϕ
n
= kn +2t(cosh μ cos k − 1) + ϕ.
It should be noted that the vector |j (8.74) has the same structure as the
corresponding vector for the NLS a nd MNLS equations; see (8.31). As regards
the dressing factor Γ , it follows from (8.69) with N =1,z
2
= −z
1
,and
¯z
2
= −¯z
1
that
Γ (n, z)=11 −
1
z − ¯z
1
|n(D
−1
)
11
)n|B + σ
3
|n(D
−1
)
21
n|B
(8.75)
−
1
z +¯z
1
|n(D
−1
)
12
n|Bσ
3
+ σ
3
|n(D
−1
)
22
n|Bσ
3
.
Calculating matrix elements D
lj
(8.70) and taking into account that det Γ
(n, 0) = exp(2μ), we obtain from (8.75)
Γ (n, z)=
11 −
sinh μ
2(z − ¯z
1
)
˜
F
−
(n) −
sinh μ
2(z +¯z
1
)
˜
F
+
(n), (8.76)
Γ
−1
(n, z)=11+
sinh μ
2(z − z
1
)
F
−
(n)+
sinh μ
2(z + z
1
)
F
+
(n),
where
˜
F
−
(n)=
⎛
⎜
⎜
⎝
exp
μ(n −
1
2
− x)+
i
2
k
cosh μ(n − 1 − x)
exp [ik(n − x)+iα − μ]
cosh μ(n − 1 − x)
exp [−ik(n − 1 − x) − iα + μ]
cosh μ(n − x)
exp
−μ(n −
1
2
− x)+
i
2
k
cosh μ(n − x)
⎞
⎟
⎟
⎠
,
F
−
(n)=
⎛
⎜
⎜
⎝
exp
μ(n −
1
2
− x)+
i
2
k
cosh μ(n − x)
exp [ik(n − x)+iα − μ]
cosh μ(n − 1 − x)
exp [−ik(n − 1 − x) − iα + μ]
cosh μ(n − x)
exp
−μ(n −
1
2
− x)+
i
2
k
cosh μ(n − 1 − x)
⎞
⎟
⎟
⎠
,
˜
F
+
(n)=−σ
3
˜
F
−
(n)σ
3
,F
+
(n)=−σ
3
F
−
(n)σ
3
.
Here
x(t)=2t
sinh μ
μ
sin k + x
0
,x
0
= −
a
μ
−
3
2
,

254 8 Dressing via local Riemann–Hilbert problem
α(t)=2t(cosh μ cos k +
k
μ
sinh μ sin k − 1) + α
0
,α
0
= β −
ak
μ
− k.
As a result, we obtain from (8.73) the AL soliton solution [8]:
u
n
(t)=exp[ik(n − x)+iα]
sinh μ
cosh μ(n − x)
.
The AL soliton depends on four constant real parameters μ, k, x
0
,andα
0
which determine the soliton mass 2μ, its group velocity v
gr
=2(sinhμ/μ)sink,
soliton maximum position x(t), and p hase α(t).
8.5 Three-wave resonant interaction equations
In this section we shall deal with the problem of three-wave resonant inter-
action in nonlinear quadratic media, one of the classic examples of successful
application of the IST to an actual physical problem. Three wave packets (en-
velopes) are involved in this process, the central frequencies and wave vectors
obeying the re sonance c onditions
ω
3
= ω
1
+ ω
2
, k
3
= k
1
+ k
2
. (8.77)
The physical nature of the wave packets can be arbitrary. The interaction of
the type (8.77) describes, e.g., a decay of wave 3 (pumping wave) into waves
1 and 2 (secondary waves). Such a process occurring in a stable medium is
exemplified by generation of harmonics in nonlinear o ptics [349] and decay
instability in plasma [226]. In unstable media, e.g., in plasma, processes of the
so-called explosive instability type are possible when the r e sonance conditions
take a rather different form:
ω
1
+ ω
2
+ ω
3
=0, k
1
+ k
2
+ k
3
=0.
The (1+1)-dimensional form of the three-wave resonant interaction (in the
following, the three-wave equations) for the case of the decay instability can
be given as
u
1t
+ v
1
u
1x
=iγ¯u
2
u
3
,
u
2t
+ v
2
u
2x
=iγ¯u
1
u
3
, (8.78)
u
3t
+ v
3
u
3x
=iγu
1
u
2
,
while for the explosive instability they are written sli ghtly differently:
u
1t
+ v
1
u
1x
=iγ¯u
2
¯u
3
,
u
2t
+ v
2
u
2x
=iγ¯u
1
¯u
3
, (8.79)
u
3t
+ v
3
u
3x
=iγ¯u
1
¯u
2
.
8.5 Three-wave resonant interaction equations 255
Here u
j
and v
j
are the scaled envelope and group velocity of the jth wave, and
γ is the interaction constant. In what follows we restrict ourselves to (8.78).
The case of the explosive instability (8.79) is treated in the same manner.
A possibility to solve the three-wave equations by means of the IST was
discovered by Zakharov and Manakov [471, 472]. The detailed analysis of the
three-wave equations can be found in [229, 234, 354]. From the point of view
of the RH problem, we will see that because an associated spectral problem
is realized with 3 × 3 matrices, some subtle details arise when determining
analytic properties of Jost solutions.
Before proceeding to solving (8.78), we discuss some peculiarit ies inherent
in these equations. The main one is the absence of dispersion in the model,
i.e., the lack of second-order derivatives in x in (8.78). The reason is that
the three-wave resonant interaction has a very short characteristic time scale
compared with that for dispersive effects. In other words, the time needed
for a three-wave interaction to e x h i b it a considerable effect on the system is
much shorter that the time required for dispersion to manifest itself. Let us
recall that in the case of the NLS model, it is the dispersion that leads to a
separation between solitons and linear waves (radiation) b ecause of the decay
of the continuous spectrum in time. In contrast, for the three-wave equations
the continuous spectrum is considered on an equal footing with the solitons:
it remains with solitons for long times and mixes nonlinearly with them.
Nevertheless, as for the NLS solitons, the three-wave soliton solutions can
be obtained in a closed form. Indeed, we can discriminate between solitons
and radiation on the basis of the properties of the RH problem associated with
the three-wave equations. Namely, there exists a subset of the RH problem
data for which a system of singular integral equations reduces to the algebraic
ones. Solutions of these algebraic equations are called solitons of the three-
wave equations. At the same time, as a manifestation of the aforementioned
mixing of solitons and radi ation, different envelopes can in general exchange
with solitons and radiation, provided that the total numb er of solitons is
preserved. Moreover, as we showed in Sect. 3.7, the previous statements still
hold for more general (non-Abelian) three-wave system.
8.5.1 Jost solutions
Let us start to solve (8.78). Those equations allow the Lax representation
ψ
x
=i(kJ + U )ψ, ψ
t
=i(kI + V )ψ, (8.80)
where J and I are diagonal matrices with constant real entries,
J =diag(a
1
,a
2
,a
3
),a
1
>a
2
>a
3
,I=diag(b
1
,b
2
,b
3
).
U and V are 3 ×3 matrices with zero diagonal. We suppose that the potential
U falls fast enough for |x|→∞,
∞
−∞
|u
ij
(x)|dx<∞∀i, j;asusual,k is the
spectral parameter. The compatibility condition for (8.80) has the form
[J, V ]=[I, U],U
t
− V
x
+i[U, V ]=0. (8.81)

256 8 Dressing via local Riemann–Hilbert problem
Then equations (8.78) are obtained from (8.81) after the following identifica-
tions:
u
1
=
u
12
(a
1
− a
2
)
1/2
,u
2
=
u
23
(a
2
− a
3
)
1/2
,u
3
=
u
13
(a
1
− a
3
)
1/2
,
α
ij
=
b
i
− b
j
a
i
− a
j
,v
1
= −α
12
,v
2
= −α
23
,v
3
= −α
13
,v
ij
= α
ij
u
ij
,
γ =
a
1
b
3
− a
3
b
1
+ a
3
b
2
− a
2
b
3
+ a
2
b
1
− a
1
b
2
[(a
1
− a
2
)(a
1
− a
3
)(a
2
− a
3
)]
1/2
,
as well as the reduction U
†
= U and, as a consequence, V
†
= V . So, explicitly
the matrices U and V are written as
U =
⎛
⎝
0 u
12
u
13
¯u
12
0 u
23
¯u
13
¯u
23
0
⎞
⎠
,V= −
⎛
⎝
0 v
1
u
12
v
3
u
13
v
1
¯u
12
0 v
2
u
13
v
3
¯u
13
v
2
¯u
23
0
⎞
⎠
.
To formulate the RH problem with the canonical normalization, we introduce
the matrix function χ = ψE
−1
,whereE =exp(ikJx). Then the spectral
problem is written as
χ
x
=ik[J, χ]+iUχ. (8.82)
Let J
±
(k, x) be the Jost solutions to the spectral problem (8.82), J
±
→ 11at
x →±∞. Then the scattering matrix S(k) is defined as before: J
−
(k, x)=
J
+
(k, x)ES(k)E
−1
.
8.5.2 Analytic solutions
As usual, we write the spectral equation for the Jost solutions in the integral
form:
J
±
(k, x)=11+
x
±∞
dy e
ikJ(x−y)
U(y)J
±
(k, y)e
−ikJ(x−y)
. (8.83)
From (8.83) we can readily ascertain that the columns J
[1]
+
and J
[3]
−
are analytic
in the upper half plane Im k>0, while J
[1]
−
and J
[3]
+
are analytic in the lower
half plane Im k<0. As regards the second columns J
[2]
±
, they do not allow an
analytic continuation off the real axis Im k = 0. The reason for such behavior is
evident: while the first and the third columns have in the integrand (8.83) the
expo n ential factors with the differences a
i
−a
j
of the same sign [(a
2
−a
1
) < 0
and (a
3
− a
1
) < 0 for the first column, and (a
1
− a
3
) > 0and(a
2
− a
3
) > 0
for the third column], the second column contains the differences of opposite
signs [(a
1
− a
2
) > 0and(a
3
− a
2
) < 0].
In order to apply the RH problem to solve (8.78), we should construct a
matrix solution to (8.82) which will possess as a whole the definite analytic
properties in the k-plane. To this end, we address ourselves to the adjoint
spectral problem
˜
ψ
x
= −i(kJ + U
T
)
˜
ψ, (8.84)
8.5 Three-wave resonant interaction equations 257
with transpose matrix U
T
. It is easy to see that if ψ is a solution to (8.80), then
(ψ
−1
)
T
is a solution to the adjoint sp ectral problem (8.84). For the problem
(8.84) we can also introduce the Jost solutions
˜
J
±
→ 11atx →±∞,which
obey the equation
˜
J
±x
= −ik[J,
˜
J
±
] − i U
T
˜
J
±
,
and a scattering matrix R(k) for the adjoint spectral problem is defined by
means of
˜
J
−
(k, x)=
˜
J
+
(k, x)ER(k)E
−1
, R =(S
−1
)
T
.
Now we can prove that the columns
˜
J
[1]
−
and
˜
J
[3]
+
are analytic for Im k>0,
while the columns
˜
J
[1]
+
and
˜
J
[3]
−
areanalyticforImk<0. So, it is natural
to construct a vector column being the vector pr oduct of the vector columns
˜
J
[1]
−
and
˜
J
[3]
+
. Being by construction analytic in the upper half plane, it can be
considered as the needed second column with the definite analytic behavior.
More exactly, the vector column
J
′
[2]
=
!
˜
J
[1]
−
×
˜
J
[3]
+
"
exp[−ik(a
1
+ a
2
+ a
3
)x]
is a solution to (8.82) and the matrix function
Φ
+
=
!
J
[1]
+
,J
′
[2]
,J
[3]
−
"
(8.85)
is a solution to (8.82) and is analytic as a whole in the upper half plane
Im k>0.
Just as in the preceding sections, we can represent Φ
+
in the form Φ
+
=
J
+
ES
+
E
−1
,where
S
−
=
⎛
⎝
r
11
00
r
12
s
33
0
r
13
−s
32
1
⎞
⎠
,S
+
=
⎛
⎝
1 −r
21
s
13
0 r
11
s
23
00s
33
⎞
⎠
.
Here s
jk
and r
jk
are entries of the matrices S and R, respectively. Besides,
we have S
+
= SS
−
.
8.5.3 RH problem
As in the case of the NLS equation, there is the involution J
†
±
(
¯
k)=J
−1
±
(k)
which permits us to construct the matrix function Φ
−1
−
(k) analytic in the lower
half plane, Φ
−1
−
(k)=Φ
†
+
(
¯
k). Thereby, we are in a position to formulate the
RH problem,
Φ
−1
−
(k, x) Φ
+
(k, x)=E(k, x)G(k)E
−1
(k, x),Φ
±
→ 11atk →∞, (8.86)

258 8 Dressing via local Riemann–Hilbert problem
with
G(k)=S
†
+
(k)S
+
(k)=
⎛
⎝
1 −r
21
s
13
−¯r
21
1 −|r
33
|
2
¯s
32
¯s
13
s
32
1
⎞
⎠
.
The contour defining the RH problem coincides with the real axis Im k =0
and the normalization is canonical.
8.5.4 Solitons of three-wave equations
Our intention is to obtain a soliton solution of the three-wave equations; hence,
we should consider the RH problem with zeros and for G(k)=
11. The discrete
spectral data of the RH problem (8.86) are given by zeros k
j
≡ ξ
j
+iη
j
of the
matrix function Φ
+
(8.85), det Φ
+
(k
j
)=0,Imk
j
> 0, and vector |j.Forthe
one-soliton solution the matrix Φ
+
reduces to the dressing factor Γ having
the form
Γ = 11 −
k
1
−
¯
k
1
k −
¯
k
1
P, (8.87)
where the projective 3 × 3matrix
P =
|p
1
|
2
+ |p
2
|
2
+ |p
3
|
2
−1
⎛
⎝
|p
1
|
2
p
1
¯p
2
p
1
¯p
3
p
2
¯p
1
|p
2
|
2
p
2
¯p
3
p
3
¯p
1
p
3
¯p
2
|p
3
|
2
⎞
⎠
(8.88)
is composed from the components of the vector |1 =(p
1
,p
2
,p
3
)
T
. Substitut-
ing the asymptotic decomposition Γ = 11+k
−1
Γ
(1)
+ ..., k →∞into (8.82),
we reconstruct the potential U from the solution of the RH problem (8.86),
U = −[J, Γ
(1)
], or, as follows from (8.87),
U =(k
1
−
¯
k
1
)[J, P ]. (8.89)
Therefore, the problem of finding the soliton solution reduces to finding the
projective matrix P . Its coordinate dependence is determined by the evolution
equations for the vector |1:
|1
x
= −ik
1
J|1, |1
t
= −ik
1
I|1.
Hence,
p
j
=exp[−i(ξ
1
+iη
1
)(a
j
x + b
j
t)]p
(0)
j
,
where the complex parameters p
(0)
j
stand for the integration constants. These
constants are determined from the initial conditions at t =0.Thereby,wehave
the explicit expression for the matr ix P (8.88). Substituting it into (8.89), we
obtain the soliton solution to the three wave equations:
u
ij
=2iη
1
(a
i
−a
j
)p
(0)
i
¯p
(0)
j
exp{−i(ξ
1
+iη
1
)[(a
i
−a
j
)x+(b
i
−b
j
)t]}∆
−1
1
, (8.90)
