Desurvire E. Classical and Quantum Information Theory: An Introduction for the Telecom Scientist
Подождите немного. Документ загружается.


21.2 Relative, joint, and conditional entropy, and mutual information 437
21.2 Relative, joint, and conditional entropy, and mutual information
The properties of VN entropy (henceforth to be referred to simply as “entropy”) are
listed in this section, along with those of related entropy variants that represent the
quantum counterparts of similar definitions in classical theory.
To recall the basics, the previous section showed that the VN entropy is nonneg-
ative (S(ρ) ≥ 0), is zero for systems in pure states (S(ρ) = S(|ψ ψ|) = 0), and has
a maximum of log n for systems of dimension n made of a uniform superposition of
states (S(ρ) = log n). Finally, the entropy is invariant under basis transformation, i.e.,
S(TρT
+
) = S(ρ), where T is a unitary matrix, which illustrates that quantum informa-
tion is an incompressible feature in quantum systems.
I shall now describe more properties of VN entropy.
Relative entropy
Our starting point in the investigation is the definition of relative entropy. This notion is
the quantum equivalent to the classical relative entropy, or Kullback–Leibler distance,
described in Chapter 5. Assume two quantum systems with density operators ρ and σ .
The relative entropy between these two systems, noted S(ρσ )isgivenby:
S(ρσ ) = tr(ρ log ρ) −tr(ρ log σ ). (21.19)
For any operator, the kernel is the space described by the set of eigenvectors having zero
for eigenvalues, and the support is the space described by the set of eigenvectors with
nonzero eigenvalues (the union of kernel and support being the complete eigenvector
space). In the definition in Eq. (21.17), the term tr(ρ log σ ) is finite only in the case
where the support of ρ does not intersect with the kernel of σ, or is fully included in the
support of σ (meaning (ρ log σ )
ii
≥ 0 for all diagonal matrix elements). In the contrary
case, we have −tr(ρ log σ ) =+∞, and the relative entropy is infinite. As a key property
of relative entropy, we have
S(ρσ ) ≥ 0, (21.20)
where the equality stands uniquely for the case ρ = σ . Such a property is referred to as
Klein’s inequality. The basic proof of Klein’s inequality is provided in Appendix U. The
consequence is that for two quantum systems with density operators ρ and σ ,wehave
S(ρ) = tr(ρ log ρ) ≥ tr(ρ log σ ), a property that we shall use later when considering
additivity rules for entropy.
Composite system in pure state
Consider, next, a composite system made of two subsystems A, B in states |ψ
A
, |ψ
B
,
and assume that it is in a pure joint state, namely |ψ=|ψ
A
|ψ
B
.Callρ
A
,ρ
B
the
density operators of the subsystems A, B. Then we have the property
S(ρ
A
) = S(ρ
B
). (21.21)

438 Quantum information theory
The proof of this property is based on the Schmidt decomposition, as described in
Appendix V. According to the Schmidt decomposition (which remains to be familiarized
with from this appendix), any pure joint state of a composite system can be expressed
in the form
|ψ=
i
x
i
|i
A
|i
B
, (21.22)
where x
i
(i = 1 ...n) are nonnegative numbers (called Schmidt coefficients) satisfy-
ing the property
i
x
2
i
= 1, and where {|i
A
}, {|i
B
} are some orthonormal basis for
the subsystems A, B. Since |ψ is a pure state, its density operator is |ψψ|.From
Eq. (21.20), we obtain
|ψψ|=
3
i
x
i
i
A
|i
B
|
43
k
x
k
|k
A
|k
B
4
=
ik
x
i
x
k
|k
A
i
A
|⊗|k
B
i
B
|.
(21.23)
We now need to make sense of this result by introducing the notion of partial trace,
which applies to composite systems. Let |i
A
, |j
A
be any two basis states in the system
A, and likewise, let |k
B
, |l
B
be any two basis states in the system B. The partial trace
tr
A
(U) of the tensor operator U =|i
A
j
A
|⊗|k
B
l
B
| is then defined as:
tr
A
U = tr
A
(|i
A
j
A
|⊗|k
B
l
B
|)
≡ tr(|i
A
j
A
|) ×|k
B
l
B
|.
(21.24)
Likewise, the partial trace tr
B
(U) is defined as
tr
B
U = tr
B
(|i
A
j
A
|⊗|k
B
l
B
|)
≡ tr(|i
B
j
B
|) ×|k
A
l
A
|.
(21.25)
It should also be noted that for any pair of orthogonal states |i, |j, and by definition
of the tensor operator |ij |,wehavetr(|ij|) =i|j=δ
ij
. We now apply the above
definitions and this last property to calculate tr
A
|ψψ| and tr
B
|ψψ| based on the
result in Eq. (21.23). We obtain:
tr
A
|ψψ|=tr
A
3
ik
x
i
x
k
|k
A
i
A
|⊗|k
B
i
B
|
4
=
ik
x
i
x
k
tr
A
(|k
A
i
A
|) ×|k
B
i
B
|
=
ik
x
i
x
k
i
A
|k
A
×|k
B
i
B
|
=
ik
x
i
x
k
δ
ik
|k
B
i
B
|
=
i
x
2
i
|i
B
i
B
|
≡ ρ
B
,
(21.26)

21.2 Relative, joint, and conditional entropy, and mutual information 439
tr
B
|ψψ|=tr
B
3
ik
x
i
x
k
|k
A
i
A
|⊗|k
B
i
B
|
4
=
ik
x
i
x
k
|k
A
i
A
|×tr
B
(|k
B
i
B
|)
=
ik
x
i
x
k
|k
A
i
A
|×k
B
|i
B
=
ik
x
i
x
k
δ
ik
|k
A
i
A
|
=
i
x
2
i
|i
A
i
A
|
≡ ρ
A
.
(21.27)
In these two equations, the identification in the right-hand side with the subsystem density
operators ρ
A
,ρ
B
comes from the aforementioned property of the Schmidt coefficients,
i.e.,
i
x
2
i
= 1, making, de facto, {x
2
i
} the corresponding probability distribution. We
must now realize that this result is quite interesting. Indeed, the only assumption that a
composite system is in a pure state, thus, implies that ρ
A
= ρ
B
, and hence the property
S(ρ
A
) = S(ρ
B
), as was claimed in Eq. (21.21). The examples described at the end of
this section provide more opportunities to apply the technique of partial tracing to the
determination of subsystem entropy.
Subadditivity inequality and quantum joint entropy
Assume a composite system AB made of two subsystems A, B.Letρ
AB
,ρ
A
,ρ
B
be
the density operators of the system and its subsystems. The corresponding entropies
S(ρ
AB
), S(ρ
A
), S(ρ
B
) are then linked by the subadditivity inequality:
S(ρ
AB
) ≤ S(ρ
A
) + S(ρ
B
), (21.28)
where the equality stands when ρ
AB
= ρ
A
⊗ ρ
B
, which corresponds to two subsystems
with uncorrelated information. The entropy of the composite system, S(ρ
AB
), which is
the quantum joint entropy, is, therefore, less than or equal to the sum of the subsys-
tem entropies. There is no surprise here, since this property appears to be the quan-
tum equivalent of that concerning the Shannon entropy H(X, Y ) of joint sources (see
Chapter 5), i.e.
H(X, Y ) ≤ H (X) + H (Y ). (21.29)
The apparent equivalence between the classical and quantum definition of joint entropy,
as intuitive and innocuous as it may appear, is in fact deceiving. We shall immediately
realize this fact by considering the case where AB is in a pure state. From the previous
property, we must have S(ρ
A
) = S(ρ
B
), but also S(ρ
AB
) = 0, since AB is in a pure
state. Thus, regardless of the information contained in subsystems A or B, which may
be finite, the joint system AB contains no information! Such a possibility is definitely
nonclassical and counterintuitive. Indeed, referring to the Venn diagrams in Fig. 5.2,

440 Quantum information theory
where H (X), H (Y ) are represented by sets with H (X, Y ) = H (X) ∪ H(Y ) there is no
possibility for H (X, Y ) =∅(empty set), except in the limiting case H (X) = H (Y ) =∅.
I shall now prove the subadditivity inequality introduced in Eq. (21.28). Basically, it
is a consequence of Klein’s inequality, which was discussed previously. We have shown
that for any pair of quantum systems of equal dimension and having density operators
ρ,σ, Klein’s inequality can be translated into
−S(ρ) = tr(ρ log ρ) ≥ tr(ρ log σ )
↔ S(ρ) ≤−tr(ρ log σ ).
(21.30)
Substituting ρ = ρ
AB
and σ = ρ
A
⊗ ρ
B
into the last inequality yields:
S(ρ
AB
) ≤−tr[ρ
AB
log(ρ
A
⊗ ρ
B
)]. (21.31)
It is left as a relatively easy exercise to show that:
log(ρ
A
⊗ ρ
B
) = log(ρ
A
) ⊗ I
B
+ I
A
⊗ log(ρ
B
), (21.32)
where I
A
, I
B
are the identity matrices of the subsystems A, B. Using this property
in Eq. (21.31), and also the additive and commutative properties of the trace (see
Eq. (17.32)) we obtain:
S(ρ
AB
) ≤−tr{ρ
AB
[log(ρ
A
) ⊗ I
B
+ I
A
⊗ log(ρ
B
)]}
=−tr[ρ
AB
log(ρ
A
) ⊗ I
B
] − tr[I
A
⊗ log(ρ
B
)ρ
AB
].
(21.33)
We must now make sense of the traces involved in the right-hand side of this equation.
Recall first that ρ
AB
is the density operator of the composite system in state |ψ.Fromthe
discussion in the previous section, and in particular the result in Eq. (21.6), we have learnt
that the expectation value of any observable U ,isgivenbyU
AB
= tr(ρ
AB
U ). There is
no reason to assume that the same outcome would be obtained by the same measurement
applied just to subsystem A separately, namely U
A
= tr(ρ
A
U ) = tr(ρ
AB
U ) =U
AB
.
This is because in the joint system AB, the subsystem B may also contribute to positive
outcomes for the same observable. On the other hand, intuition dictates that there must
exist an operator U
such that the expected value of U
in the joint system AB and that
of U in the subsystem A do coincide, i.e.,
U
AB
=U
A
↔ tr(ρ
AB
U
) = tr(ρ
A
U ). (21.34)
It is a fine academic issue to show that U
= U ⊗ I
B
, which expands U to the full system
space, is, in fact, the only candidate to solve such a measurement reconciliation problem.
Here, we may just accept it as an intuitive postulate. Hence,
tr[ρ
AB
log(ρ
A
) ⊗ I
B
] = tr(ρ
A
log ρ
A
) ≡−S(ρ
A
)
tr[I
A
⊗ log(ρ
B
)ρ
AB
] = tr(ρ
B
log ρ
B
) ≡−S(ρ
B
),
(21.35)
to be followed by substitution in Eq. (21.33) to yield the inequality S(ρ
AB
) ≤ S(ρ
A
) +
S(ρ
B
), as claimed in Eq. (21.28), which is now established.
As also claimed earlier, the equality in Eq. (21.28) stands when (and only when)
ρ
AB
= ρ
A
⊗ ρ
B
, meaning that the entropies of the subsystems are additive, according

21.2 Relative, joint, and conditional entropy, and mutual information 441
to:
S(ρ
AB
) ≡ S(ρ
A
) + S(ρ
B
). (21.36)
Proving this equality is straightforward. Indeed, since ρ
AB
= ρ
A
⊗ ρ
B
, we can now
write S(ρ
AB
) ≡−tr[ρ
AB
log(ρ
A
⊗ ρ
B
)], and as we have seen from the results in
Eqs. (21.32) to (21.35), the right-hand side is identical to S(ρ
A
) + S(ρ
B
).
Quantum mutual information
As we have learnt from Chapter 5,themutual information H (X ; Y ) measures the amount
of information correlation between the two event sources X, Y , as defined by
H(X ; Y ) = H (X ) + H (Y ) − H (X, Y ). (21.37)
If the sources are uncorrelated, the equality in Eq. (21.29) stands and H(X ; Y )in
Eq. (21.37) is zero. We may define quantum mutual information S(ρ
A
; ρ
B
) through
S(ρ
A
; ρ
B
) = S(ρ
A
) + S(ρ
B
) − S(ρ
AB
). (21.38)
As in the classical case, S(ρ
A
; ρ
B
) represents the measure of information correlation
between the two quantum subsystems A, B. In the absence of any correlation (ρ
AB
=
ρ
A
⊗ ρ
B
), the equality in Eq. (21.28) stands, and S(ρ
A
; ρ
B
) is zero. From Eq. (21.28),
we note that S(ρ
A
; ρ
B
) ≥ 0 in all cases, meaning that the quantum mutual information
is always nonnegative. In the case where the joint system AB is in a pure state, we have
S(ρ
AB
) = 0 and S(ρ
A
) = S(ρ
B
), which implies that S(ρ
A
; ρ
B
) = 2S(ρ
A
) = 2S(ρ
B
).
Such a result is definitely nonclassical. Indeed, referring to the Venn diagrams in
Fig. 5.2, where H (X ), H (Y ) are represented by sets with H (X; Y ) = H (X) ∩ H (Y ),
there is no possibility for H(X ; Y ) = 2H (X)orH(X; Y ) = 2H (Y ), except in the limit-
ing case H (X) = H (Y ) =∅.
If two quantum systems have correlated information, we shall say from now on that
their quantum states are entangled. The end of this section will provide examples of
entangled states.
Conditional entropy
Given two subsystems A, B and their composite system AB,theconditional entropy
S(ρ
A
|ρ
B
) is defined as
S(ρ
A
|ρ
B
) = S(ρ
AB
) − S(ρ
B
), (21.39)
and, likewise, S(ρ
B
|ρ
A
) = S(ρ
AB
) − S(ρ
A
). This definition is the quantum equivalent
of the classical case concerning joint events from two sources X, Y , namely (see
Chapter 5):
H(X |Y ) = H (X, Y ) − H (X), (21.40)

442 Quantum information theory
and, likewise, H (Y |X) = H (X, Y ) − H (Y ). Where the information in the two subsys-
tems is uncorrelated (S(ρ
AB
) ≡ S(ρ
A
) + S(ρ
B
)), we have, from Eq. (21.39):
S(ρ
A
|ρ
B
) = S(ρ
A
)
S(ρ
B
|ρ
A
) = S(ρ
B
),
(21.41)
which translates that the information of one subsystem is not affected by the knowledge
of the information in the other subsystem, as expected in the uncorrelated case. Again,
while we observe a nice parallel between the classical and the quantum definitions
for conditional entropy, we should know for a fact (from preceding analysis) that it is
deceptive! Indeed, in the case where AB is in a pure state, we have S(ρ
AB
) = 0 and, from
Eq. (21.39), S(ρ
A
|ρ
B
) =−S(ρ
B
) ≤ 0 (as per the nonnegativity of S(ρ
B
)) and, likewise,
S(ρ
B
|ρ
A
) =−S(ρ
A
) ≤ 0. Hence, the quantum conditional entropy can be negative,
which is definitely a nonclassical feature. Since, in this case, S(ρ
A
) = S(ρ
B
), we also
have
S(ρ
A
|ρ
B
) =−S(ρ
A
)
S(ρ
B
|ρ
A
) =−S(ρ
B
),
(21.42)
which contrast with the correlated case expressed in Eq. (21.41).
Triangle inequality
The subadditivity inequality in Eq. (21.28) provided an upper bound to the joint entropy
S(ρ
AB
), namely S(ρ
AB
) ≤ S(ρ
A
) + S(ρ
B
). The triangle inequality,alsoreferredtoas
the Araki–Lieb inequality, provides a lower bound to S(ρ
AB
), according to
|S(ρ
A
) − S(ρ
B
)|≤S(ρ
AB
). (21.43)
To prove this property, assume the existence of a third system R, such as the composite
system ABR in a pure state. The system R is referred to as a purifying system for AB
(see Appendix W). From the subadditivity inequality we find
S(ρ
AR
) ≤ S(ρ
A
) + S(ρ
R
). (21.44)
Because ABR is in a pure state, we also find that S(ρ
AB
) = S(ρ
R
) and S(ρ
AR
) =
S(ρ
B
) (see earlier subsection on composite system in pure state). Substituting these
last two equalities into Eq. (21.44) we obtain S(ρ
AB
) ≥ S(ρ
B
) − S(ρ
A
). Since A and B
play a symmetric role, we also have S(ρ
AB
) ≥ S(ρ
A
) − S(ρ
B
), and, hence, S(ρ
AB
) ≥
±|S(ρ
A
) − S(ρ
B
)|, which proves the property in Eq. (21.43). It can be shown (but the
proof is not to be considered here) that the equality in Eq. (21.43) isgivenbythe
condition ρ
AR
= ρ
A
⊗ ρ
R
, which means that the A, R information is uncorrelated and,
therefore, that all possible correlation of A information with the rest of the world is
exclusively with B.

21.2 Relative, joint, and conditional entropy, and mutual information 443
Concavity of entropy and entropy of system in random states
Assume a quantum system that can be in any random mixed state |ψ
i
, according to
some known probability distribution p
i
. Each of these random states is associated with
a density operator ρ
i
.Theconcavity of a function f (x) corresponds to the property
f (x)≤f (x). Here, I shall establish that entropy is a concave function of the density-
operator variable ρ
i
, namely,
S(ρ)≤S(ρ), (21.45)
or, formally:
i
p
i
S(ρ
i
) ≤ S
3
i
p
i
ρ
i
4
. (21.46)
Furthermore, if one assumes that the set of operators ρ
i
has support on orthogonal
subspaces (meaning that the set of all possible eigenstates form an orthonormal basis),
an exact relation also exists between S(ρ) and S(ρ), which nicely relates to the
Shannon entropy of a classical source H (X ) =−
i
p
i
log p
i
, according to:
S(ρ) =S(ρ)+H(X), (21.47)
or, formally:
S
3
i
p
i
ρ
i
4
=
i
p
i
S(ρ
i
) + H (X). (21.48)
The property expressed in Eq. (21.47) or Eq. (21.48) can easily be interpreted as follows:
the quantum information of a system whose states are random equals the mean infor-
mation, as averaged over the individual states, plus the information on the probability
distribution, which is the Shannon entropy. Note that since H (X) is nonnegative, this
property also establishes the concavity of the entropy, as expressed in Eq. (21.45) or
Eq. (21.46). In the case where the probability distribution is uniform, the Shannon
entropy H (X) is maximal, meaning that there is maximal uncertainty as to which quan-
tum state the system is in. Such a situation also maximizes the entropy S(ρ)ofthe
system. The deterministic case where the system has only one possible quantum state
corresponds to H (X) = 0 and a minimum entropy.
The demonstration of the property expressed in Eq. (21.48) is relatively simple.
Indeed, let |λ
k
i
and λ
k
i
be the eigenstates and eigenvalues for each quantum state
associated with the density operator ρ
i
. Hence ρ
i
has diagonal matrix elements (ρ
i
)
kk
=
λ
k
i
. Next, given any i, k we observe that |λ
k
i
and p
i
λ
k
i
are eigenvectors and eigenvalues of

444 Quantum information theory
ρ=
j
p
j
ρ
j
,
3
making {|λ
k
i
} an orthonormal basis for ρ, regardless of the choice
of i. Hence, ρhas diagonal matrix elements ρ
kk
= p
i
λ
k
i
and (logρ)
kk
= log( p
i
λ
k
i
).
Using these different properties, we obtain
S(ρ) =−tr(ρlogρ)
=−
k
#
i
p
i
ρ
i
$
kk
(logρ)
kk
=−
ik
p
i
(ρ
i
)
kk
(
logρ
)
kk
=−
ik
p
i
λ
k
i
log
p
i
λ
k
i
=−
ik
λ
k
i
p
i
log p
i
−
ik
p
i
λ
k
i
log λ
k
i
=−
i
p
i
log p
i
k
λ
k
i
+
i
p
i
#
−
k
λ
k
i
log λ
k
i
$
≡ H (X) +
i
p
i
S(ρ
i
),
(21.49)
which proves Eqs. (21.46) and (21.47). As we have seen, the property thus estab-
lished rests on the assumption that the set of operators ρ
i
has support on orthog-
onal subspaces. If such a condition is not met, the equality in Eq. (21.47) or
Eq. (21.48) does not hold and the originator-source entropy H(X)isonlyanupper bound,
namely:
S(ρ) −S(ρ)≤H(X), (21.50)
or, formally:
S
3
i
p
i
ρ
i
4
−
i
p
i
S(ρ
i
) ≤ H (X). (21.51)
Here, for the sake of conciseness and to keep the momentum, we shall not go through
the detailed demonstration of the inequalities in Eqs. (21.50) and (21.51). The key point
is to remember that the equality in these relations is not guaranteed, except in the case
where the subspaces defined by ρ
i
are orthogonal. In all cases, the entropy H (X) remains
the upper bound. This general property for the concavity of entropy shallbeusedinthe
next section, concerned with the so-called Holevo bound.
This tedious inventory of entropy properties, which has been limited here to the most
elementary ones, now calls for a few illustrative examples. I shall consider three basic
possibilities for a composite quantum system AB made of two subsystems A, B, namely:
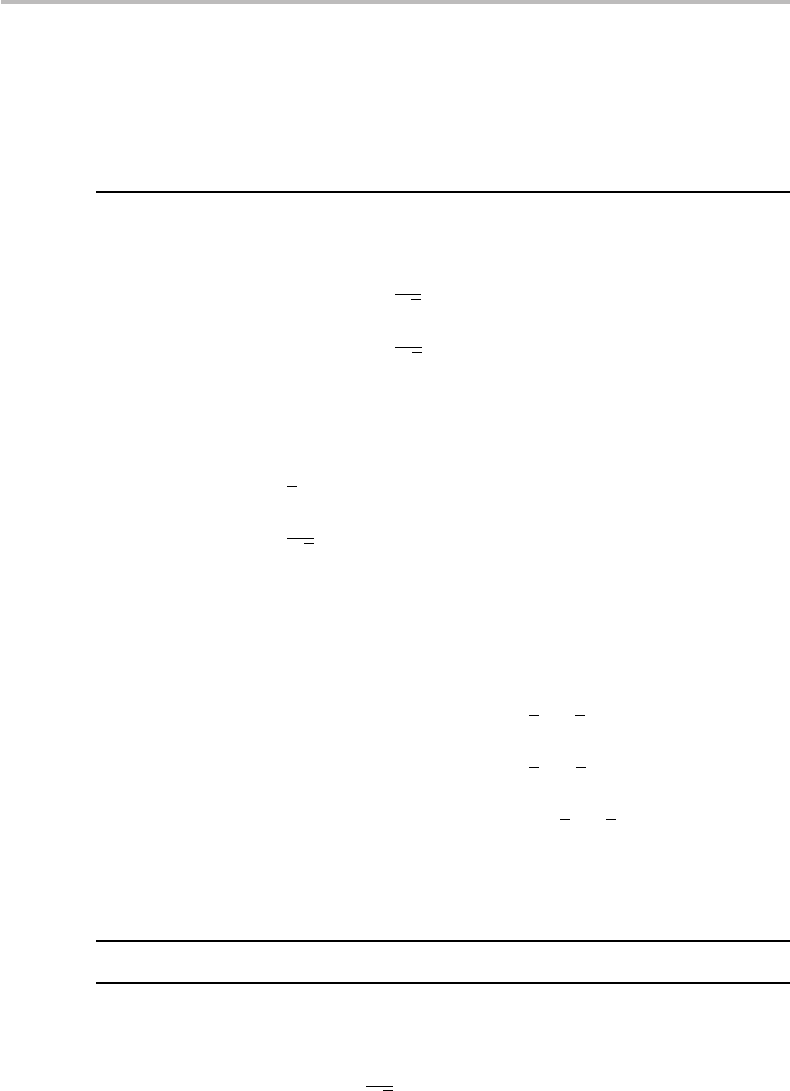
3
Indeed, we have
j
p
j
ρ
j
|λ
k
i
=
#
jl
p
j
λ
l
j
|λ
l
j
λ
l
j
|
$
|λ
k
i
=
jl
p
j
λ
l
j
|λ
l
j
λ
l
j
|λ
k
i
=
jl
p
j
λ
l
j
|λ
k
i
δ
ij
δ
kl
≡ p
i
λ
k
i
|λ
k
i
.
21.2 Relative, joint, and conditional entropy, and mutual information 445
(1) when the subsystem information is uncorrelated, (2) when it is correlated, and
(3) when the composite system is in a pure state. The last two examples will also
highlight the interest and implications of using partial tracing, and illustrate the concept
of state entanglement.
Example 21.1: Subsystems with uncorrelated information
Consider, for instance, two subsystems A, B in respective qubit states:
4
|ψ
A
=
1
√
2
(|0+|1)
A
|ψ
B
=
1
√
3
(|0+|1+|2)
B
.
(21.52)
The joint state of the composite system is assumed to be
|ψ
AB
=|ψ
A
⊗|ψ
B
=
1
2
(|0+|1)
A
⊗ (|0+|1+|2)
B
=
1
√
6
(|00+|01+|02+|10+|11+|12)
AB
.
(21.53)
It is clear from the above definitions that the three systems A, B, AB are expressed in
their respective eigenstate bases and, thus, have density matrices of ρ
A
= I
A
/2, ρ
B
=
I
B
/3, and ρ
AB
= I
AB
/6, respectively, with the property ρ
AB
= ρ
A
⊗ ρ
B
. We therefore
have
S(ρ
A
) =−tr[(I
A
/2) log(I
A
/2)] =−2 ×
1
2
log
1
2
≡ 1
S(ρ
B
) =−tr[(I
B
/3) log(I
B
/3)] =−3 ×
1
3
log
1
3
≡ 1.584
S(ρ
AB
) =−tr[(I
AB
/6) log(I
AB
/3)] =−6 ×
1
6
log
1
6
≡ 2.584,
(21.54)
which shows that S(ρ
AB
) = S(ρ
A
) + S(ρ
B
) and illustrates that entropy is additive
for subsystems with uncorrelated information (namely satisfying ρ
AB
= ρ
A
⊗ ρ
B
or
|ψ
AB
=|ψ
A
⊗|ψ
B
), or, equivalently, whose quantum states are not entangled.
Example 21.2: Subsystems with correlated information
Consider, for instance, a composite system AB with the joint state
|ψ
AB
=
1
√
6
(|00+2|10+|11)
AB
, (21.55)
4
For each subsystem, it is possible to choose the eigenstate basis {|i } in which each component ψ |i is in
phase with |i, hence ψ|i≥0, meaning that the example where all components are positive is actually not
restrictive.

446 Quantum information theory
which corresponds to the density operator
ρ
AB
=
1
6
(|0000|+4|1010|+|1111|). (21.56)
It is straightforward to check that tr(ρ
2
AB
) = 1/2 < 1, which illustrates that |ψ
AB
is
not a pure state. It is also a basic exercise to show that |ψ
AB
, like other states having
similar decomposition with any other nonzero coefficients, cannot be decomposed into
a tensor product of two subsystem states |ψ
A
, |ψ
B
. Because of this, we conclude that
the information in the two subsystems is correlated, or that the subsystem states are
entangled.
So far, we only know that the quantum information in system AB is:
S(ρ
AB
) =−2 ×(1/6) log(1/6) − 0 ×log(0) − 1 ×(4/6) log(4/6)
≡ 1.251.
(21.57)
Given the above assumptions, can we get any knowledge of the quantum information in
subsystems A, B? The answer is yes, if we use the tool of partial tracing. Indeed, we
may rewrite Eq. (21.55) as a sum of tensor products:
ρ
AB
=
1
6
(|00|
A
⊗|00|
B
+ 4|11|
A
⊗|00|
B
+|11|
A
⊗|11|
B
). (21.58)
Applying partial tracing over A from the definition in Eq. (21.24), while using the
property tr(|ii|) =i |i≡1, we obtain:
tr
A
(ρ
AB
) =
1
6
tr
A
(|00|
A
⊗|00|
B
+ 4|11|
A
⊗|00|
B
+|11|
A
⊗|11|
B
)
=
1
6
[tr
A
(|00|
A
⊗|00|
B
) + 4tr
A
(|11|
A
⊗|00|
B
) + tr
A
(|11|
A
⊗|11|
B
)]
=
1
6
[tr
A
(|00|
A
) ×|00|
B
+ 4tr
A
(|11|
A
) ×|00|
B
+ tr
A
(|11|
A
) ×|11|
B
]
=
1
6
(|00|
B
+ 4|00|
B
+|11|
B
)
=
1
6
(5|00|
B
+|11|
B
) ≡ ˆρ
B
. (21.59)
The operator ˆρ
B
= tr
A
(ρ
AB
) is referred to as the reduced density operator of subsystem
B. Likewise, we obtain
tr
B
(ρ
AB
) =
1
6
(|00|
A
+ 5|11|
A
) ≡ ˆρ
A
. (21.60)
For the sake of curiosity, let us now calculate the tensor product ˆρ
A
⊗ ˆρ
B
from
Eqs. (21.59) and (21.60). We obtain
ˆρ
A
⊗ ˆρ
B
=
1
36
(|00|
A
+ 5|11|
A
) ⊗ (5|00|
B
+|11|
B
)
=
1
36
(5|0000|+|0101|+25|1010|+5|1111|)
AB
.
(21.61)
