Desurvire E. Classical and Quantum Information Theory: An Introduction for the Telecom Scientist
Подождите немного. Документ загружается.

17.2 Quantum measurements and types 347
Substituting the expansion of |ψ, with complex coordinates x
i
, over the eigenstate
basis {|λ
i
}, we obtain for the measurement probability p(λ
m
) and corresponding post-
measurement state |ψ
:
p(λ
m
) =ψ|P
m
|ψ
=
3
n
i=1
λ
i
|
¯
x
i
4
|λ
m
λ
m
|
n
j=1
x
j
|λ
j
=
n
i=1
n
j=1
¯
x
i
x
j
δ
im
δ
jm
=|x
m
|
2
,
(17.57)
|ψ
=
1
p(λ
m
)
P
m
|ψ
=
1
x
m
|λ
m
λ
m
|
n
i=1
x
i
|λ
i
=
1
x
m
|λ
m
n
i=1
x
i
δ
im
≡|λ
m
.
(17.58)
We will use the above two results in Chapter 18, which concerns projective qubit
measurements.
Let us now analyze some interesting properties of the projective or von-Neumann
measurement.
Since each eigenvalue λ
m
has an associated measurement probability, p(λ
m
), we can
define the average value or mean of the observable measurement as follows:
A≡λ=
n
m=1
λ
m
p(λ
m
) =
n
m=1
λ
m
ψ|P
m
|ψ
=ψ |
n
m=1
λ
m
P
m
|ψ≡ψ|A|ψ.
(17.59)
The nice conclusion from the above result is that the average value of an observable A is
defined as the bracket ψ|A|ψ. Likewise, we can define the mean-square and variance
of the observable as follows:
A
2
≡λ
2
=
n
m=1
λ
2
m
p(λ
m
) ≡ψ |A
2
|ψ, (17.60)
A
2
≡λ
2
−λ
2
=A
2
−A
2
(17.61)
(the notation A corresponds to a real number, not an operator). We have, thus, obtained
the definitions of the two main statistical parameters characterizing any observable
measurement, namely the mean and the variance of the observed physical quantity. We
call the uncertainty of the observable A the measured standard deviation
√
A
2
= A.
Similar measurements can be performed with any other observable quantity B, with
uncertainty B. A fundamental result in quantum mechanics, known as the Heisenberg
uncertainty principle, states that given two Hermitian operators A, B the product of their

348 Quantum measurements
uncertainties measured in the system state |ψ must satisfy:
AB ≥
1
2
|ψ|[ A, B]|ψ|, (17.62)
where [A, B] = AB − BA is the commutator of A, B (see demonstration in Appendix
O). In the particular case (called conjugate observables) where [ A, B] =±i , and where
|ψ has a modulus of unity, we have
AB ≥
1
2
, (17.63)
which represents the more well-known form of the uncertainty principle. The key con-
clusion of this principle could be stated as follows: two independent measurements of
noncommuting observables cannot both reach arbitrary accuracy. The product of the
corresponding uncertainties has a nonzero lower bound given by the right-hand side of
Eq. (17.62), which we may call ε. Thus, a relatively accurate measurement of A, e.g.,
A = 1/N where N may be any large number, implies that the accuracy in the measure-
ment of B cannot be better than N ε.Thus,ifN →∞,wehaveA → 0, corresponding
to absolute accuracy in the observable A, while we have B →∞, corresponding to
an absolute “indetermination” of the observable B. It would be incorrect, however, to
conclude that in any case, a first measurement of A actually influences that of B, and the
reverse. The two measurements are assumed to be independent, both in terms of time
and order sequence. Only in the case where the two observables commute can the two
measurements reach arbitrary accuracy.
POVM measurements
A third type of measurement is referred to as positive-operator-valued-measure,or
POVM. Define a finite set of Hermitian operators {E
m
} with any E
m
being a posi-
tive operator (meaning ψ|E
m
|ψ≥0 for any normalized state |ψ), and altogether
satisfying the completeness relation
m
E
m
= I. (17.64)
The operators E
m
that satisfy the above conditions are then said to be POVM operators.
As we have seen in the previous section, any Hermitian operator A can be represented
by a diagonal matrix with nonnegative coefficients, hence, there exists a diagonal oper-
ator B =
√
A, such that B
2
= A, and which is also Hermitian (B
2
ii
is real, so B
ii
is).
Therefore, for each E
m
we can associate a Hermitian operator M
m
=
√
E
m
that satisfies
M
+
m
M
m
= E
m
, and consequently the two above POVM conditions. According to the
general definition, the set {M
m
} is, thus, a truly eligible set of measurement operators,
even if their number, with respect to the number n of pure states, is not specified. The
projection or von-Neumann measurements, with the set {M
m
=|λ
m
λ
m
|}being defined
by the n eigenstates of an observable, represent a specific case of POVM set.
I shall now illustrate an important application of POVM, which no other measurement
type can provide. Consider a simple example in the basis V ={|0, |1}. Assume the

17.2 Quantum measurements and types 349
Table 17.1 Probabilities associated with different POVM measurement possibilities when the input
state is either |ψ=|0 (left) or |ψ=|+(right).
Input state |ψ=|0 Input state |ψ =|+
p(1) =0|E
1
|0=0 p(1) =+|E
1
|+ =
u
2
p(2) =0|E
2
|0=
u
2
p(2) =+|E
2
|+ = 0
p(3) =0|(I − E
1
− E
2
)|0=1 −
u
2
p(3) =−|(I − E
1
− E
2
)|− = 1 −
u
2
three POVM operators:
E
1
= u|11|
E
2
= u
|0−|1
√
2
0|−1|
√
2
≡ u|−−|
E
3
= I − E
1
− E
2
,
(17.65)
with u =
√
2/(1 +
√
2). By summation of the above three equations, we immediately
observe that the completeness relation is satisfied. It is also easily verified that the three
operators E
1
, E
2
, E
3
are positive (it is left as an exercise to prove that in the general
case, the condition u ≤ 2/3 must be satisfied). We have, thus, defined a valid POVM set
of three measurement operators from the two-element basis V .
The following will show how the above POVM measurement can be applied. Assume
that the system state |ψ has been prepared in such a way that it is certain to be either
|ψ=|0 or |ψ=|+, to the exclusion of any other possibility. It is also assumed
that we have this key information about the two system-state possibilities, prior to any
measurement, but we don’t know, a priori, which ones the system chooses to be in. The
probabilities associated with our three possible measurements associated to each case
are summarized in Table 17.1.
First, the table indicates that our measurement fails to convey any information in two
cases:
r
If we use E
1
when the input is |ψ=|0;
r
If we use E
2
when the input is |ψ=|+.
This is because, in each case, the measurement operators project the input state on its
orthogonal counterpart, namely |1 or |−, respectively, resulting in the measurement
probabilities for E
1
of p(E
1
) =0|E
1
|0=0or p(E
2
) =+|E
2
|+ = 0, and showing
that these measurement have no possible outcomes.
Second, the table indicates that there is a finite probability u/2 that we obtain a
measurement
r
Of E
1
,ifweuseE
1
when the input is |ψ=|+;
r
Of E
2
,ifweuseE
2
when the input is |ψ=|0.
Then comes a nice subtlety in the interpretation of these two measurement possibilities.
Indeed, if we happen to measure E
1
the system cannot be in state |ψ =|0, since E
1
is

350 Quantum measurements
a projector on |1. In this case, we reach the absolute conclusion that the system must
have been in state |ψ=|+. Likewise, if we happen to measure E
2
, the system cannot
be in state |ψ=|+, since E
2
is a projector on |−. In this case, we reach the absolute
conclusion that the system must have been in state |ψ=|0. However, we obtain this
absolute information only with probability u/2, meaning that there is a finite probability
1 − u/2 that the two measurements fail to convey any information.
If we were to use the measurement E
3
, Table 17.1 shows that the measurement works
in all cases (|ψ=|0 or |+), but with a probability 1 −u/2. By definition, E
3
is a
projector on all states that are neither |1 or |−. Thus, the positive outcome of any E
3
measurement only tells us that the system state is neither |1or |−, but we already know
this for a fact, which represents no information! Therefore, there is no point in using E
3
as a means to measure the system state.
Let me, then, clarify what is meant by “positive outcome” and “failure” of any of the
above measurements, using a figurative analogy with a physical measurement. Compare
the system state |ψ to a light source that randomly emits in two possible color tones,
either A or B (standing for |0 or |+, respectively), these tones being invisible to the
naked eye. Our measurement consists of determining which color tone is emitted by the
source by observing it through a set of “magic filters,” called 1, 2, 3, (for E
1
, E
2
, E
3
).
Such filters have the following strange properties: filter 1 does not react to tone A,
while it makes tone B visible to the eye with probability p (or u/2); filter 2 does not
react to tone B, while it makes tone A visible to the eye with same probability p; and
filter 3 does not react to any tone other than A, B (but this is not useful here) but
makes tone A, B visible with probability 1 − p (or 1 −u/2). Basically, if we choose
either filter 1 or filter 2, we have a chance p of seeing something, and 1 − p of seeing
nothing!
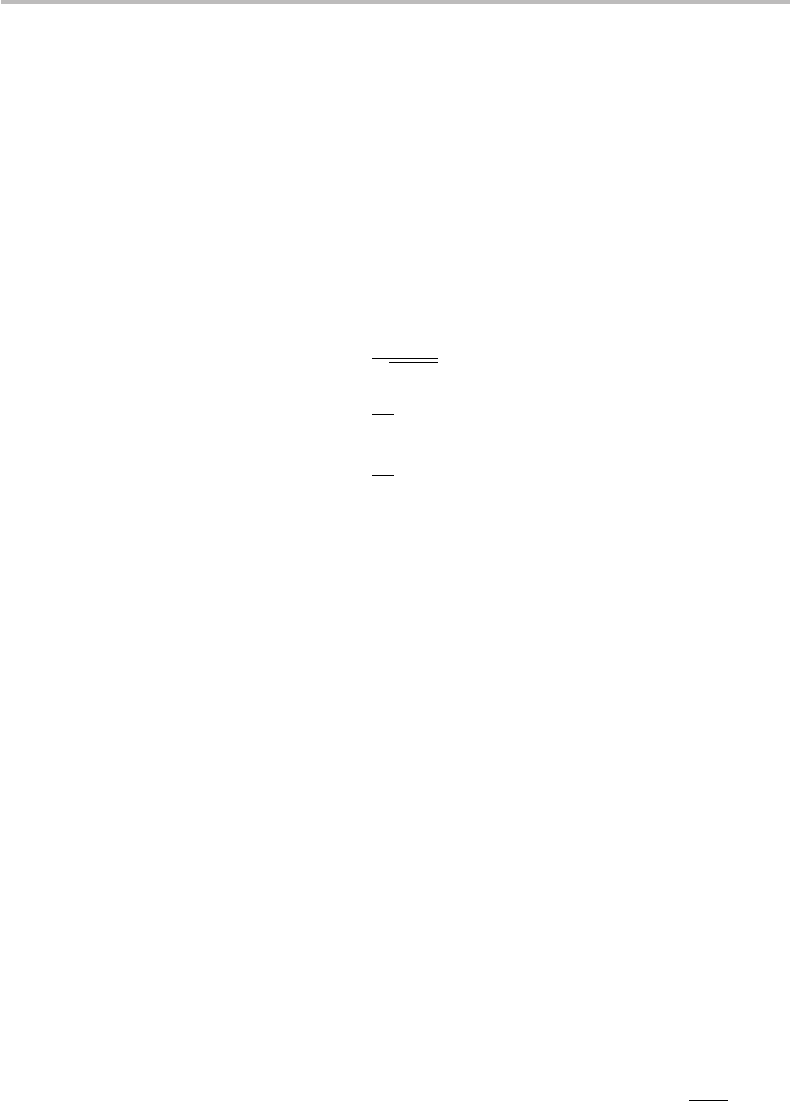
Figure 17.1 shows what we can see through the magic filters, according to the twelve
possible cases, namely, determined by the two source-tone possibilities A, B and our
three possible magic-filter choices 1, 2, 3. A bright spot indicates that we observe some-
thing, corresponding to a “positive” measurement. The absence of a spot, or dark image
indicates a “negative” or “failed” measurement. The figure shows that the combina-
tions (A, 1) and (B, 2) are certain to fail, while the other combinations ( A, 2) (B, 1)
or ( A, 3) (B, 3) have a finite chance, p or 1 − p, respectively, of succeeding. The suc-
cessful measurements in the two cases ( A, 2) or (B, 1), as marked with a cross (×),
correspond to absolute certainty that the source tone is A or B, respectively. The other
two successful measurements (A, 3) (B, 3), like all failed measurements, do not convey
any information, as mentioned earlier. The success of our measurement and the absolute
conclusion therein, is, thus, dependent on our filter choice (1 or 2), which is essentially
a matter of guesswork.
From any academic standpoint, it remains debatable whether the above fictitious
“physical measurement” through a set of “magic filters” may, in some way, help clarify
the essence of a true quantum POVM measurement. If it has any merit, however, it
helps to stress the point that quantum measurements are based on the observer’s choice
of a measurement operator (the magic filter). The measurement or observation of an
outcome may either succeed or fail (seeing or not seeing a spot). In the case of success,
17.3 Quantum measurements on joint states 351
A
1
2
3
1
p
p
B
p
1 −
p
1 −
p
1 −
p
1 −
p
p
x
x
0
1
0
Figure 17.1 Fictitious analogy of POVM measurement of tones A, B and a magic-filter set 1, 2, 3
to characterize a source. Given the tones A, B and choices 1, 2, 3, a positive observation of the
tone is characterized by the bright spot on the images shown, with various probabilities
0, 1, p, 1 − p. The two images marked with a cross (×) correspond to absolutely certain
measurements that the source tone is A or B.
the observer may or may not be able to derive an absolute conclusion as to what state
was observed (the source tone), depending on his or her choice.
Leaving fiction behind, we can conclude that POVM measurements make it pos-
sibletogetabsolutely certain information about the input state |ψ , despite the fact
that it is, a priori, unknown, just as the right measurement operators are unknown.
We have noted that while the corresponding measurements may sometimes fail, the
conclusion is never wrong when they succeed. This remarkable property is referred to
as unambiguous quantum state discrimination, of UQSD. Remarkably, while UQSD
has no classical explanation, or no counterpart in classical physics, it can be imple-
mented in actual physical measurements, for instance, in quantum cryptography (see
Chapter 25).
17.3 Quantum measurements on joint states
In Chapter 16, we introduced the concept of tensor states, which correspond to systems
being described by more than one quantum state, e.g., |ψ
1
and |ψ
2
. Here, we shall
call such systems composite systems. As we have seen, the corresponding state can be
written in the tensor form |ψ=|ψ
1
⊗|ψ
2
,or|ψ =|ψ
1
|ψ
2
, or, for short |ψ=
|ψ
1
,ψ
2
, and even, sometimes, |ψ=|ψ
1
ψ
2
, e.g., the notations |ψ =|i ⊗|j≡
|i|j ≡|i, j≡|ij are all equivalent. These tensor states are also referred to as joint
states. Regardless of the notation conciseness for joint states, however, it is important to

352 Quantum measurements
keep in mind the underlying tensor product concept, which is also associated with that
of tensor operators. Thus, the tensor operator U = A ⊗ B acts on the joint state |ψ=
|ψ
1
⊗|ψ
2
according to the rules U |ψ =A|ψ
1
⊗B|ψ
2
and ψ|U
+
=ψ
1
|A
+
⊗
ψ
2
|B
+
, hence, the imperative need of keeping the single quantum state notation in the
right order sequence inside the ket |·, and choose the appropriate notation for the tensor
products when operators are being used.
We are now interested in defining a generic measurement operator for joint states. The
following may look complicated at first but, as we shall see, the result makes complete
sense in view of what we have learnt from the previous section. Assume first a single-state
space V , as defined by the orthonormal basis V
n
={|x
i
} (for simplicity we identify
here the “space” and one corresponding “basis” with the same letter V ). Assume next
that {M
m
} represents a valid set of measurement operators for V (meaning that M
+
m
M
m
are positive and verify the completeness relation). For the set {M
m
} there is a space
M of orthonormal states {|m}, corresponding to each of the different possibilities of
post-measurement states resulting from the action of M
m
. I shall refer to M as an ancilla
space.Let|
˜
m be a fixed state of M, to be used as an arbitrary reference, which we
might index |
˜
m=|0. We can now work with the joint states |ψ|0=|ψ, 0 from the
extended space V ⊗ M of joint states |ψ|m=|ψ, m. Next, define the tensor operator
U whose action on |ψ, 0 results in:
U |ψ, 0=
m
M
m
|ψ, m. (17.66)
We then have for any two states |ψ, |ψ
:
ψ
, 0|U
+
U |ψ, 0=
k
m
ψ
, k|M
+
k
M
m
|ψ, m
=
k
m
ψ
|M
+
k
M
m
|ψδ
km
=
m
ψ
|M
+
m
M
m
|ψ
=ψ
|
m
M
+
m
M
m
|ψ≡ψ
|ψ.
(17.67)
The above result shows that the transformation U preserves the inner product of all
states |ψ, 0 as a unitary operator for the subspace V ⊗{|0}. Actually, we can make
U a unitary operator for the entire space V ⊗ M, which applies to any state |ψ, m,
6
6
Indeed, define U
as the sum of two projectors on the two orthogonal spaces V ⊗{|0} and V ⊗{|m = 0}
for any state |ψ∈V , as follows: U
= U |ψ, 0ψ, 0|+
m=0
|ψ, mψ, m|. It is clear that since U
is unitary, U
is unitary. We also have U
|ψ, 0=U
|ψ, 0 and, for k = 0, U
|ψ, k=U|ψ, 0ψ, k|+
m=0
|ψ, mψ, m|ψ, k=U δ
0k
+
m=0
|ψ, mδ
mk
≡|ψ, k.

17.3 Quantum measurements on joint states 353
according to the alternative definition:
U
k
|ψ, k=
m
(M
m
⊗|km|)|ψ, k
=
m
M
m
|ψ⊗(|km|k)
=
m
M
m
|ψ⊗(|kδ
mk
)
=
m
M
m
|ψ, m⊗(|kδ
mk
).
(17.68)
As an exercise, it is possible to check that ψ
, k
|U
+
k
U
k
|ψ, k≡ψ
|ψδ
kk
which shows
that the new definition of U (i.e., U
k
)inEq.(17.68) is indeed unitary over V ⊗ M.Having
found the above unitary operator U that conserves the inner product of all joint states
|ψ, k in V ⊗ M, we can now introduce the joint-state measurement (JSM) operator E
m
as
E
m
= U
+
P
m
U, (17.69)
with
P
m
= I
V
⊗|mm|, (17.70)
where I
V
is the identity operator on V and |mm|the projector over |m∈M.
7
Next, we
shall calculate the bracket function p(m) =ψ, k|E
m
|ψ, k, which, using Eq. (17.66)
with |0=|k,is:
p(m) =ψ, k|U
+
P
m
U |ψ, k
=
3
p
ψ, p|M
+
p
4
(I
V
⊗|mm|)
3
q
M
q
|ψ, q
4
=
p
q
ψ|M
+
p
M
q
|ψp|mm|q
=
p
q
ψ|M
+
p
M
q
|ψδ
pm
δ
mq
≡ψ |M
+
m
M
m
|ψ.
(17.71)
We immediately recognize from the result that p(m)isthemeasurement probability
associated with the operator M
m
on the state |ψ∈V , as defined in Eq. (17.46).After
Eq. (17.50), and writing the JSM operator E
m
= (U
+
P
+
m
)P
m
U , we obtain the joint
post-measurement state as the result of the transformation:
|ψ
, k
=
1
p(m)
P
m
U |ψ, k
=
1
ψ|M
+
m
M
m
|ψ
M
m
|ψ, m.
(17.72)
7
The full definition of the JSM operator actually being E
m
= ( P
m
U)
+
P
m
U = U
+
P
+
m
P
m
U ≡ U
+
P
m
U,
where we used the projector and Hermitian properties of P
m
.
354 Quantum measurements
This final result shows that after the measurement, the state |ψof space V is transformed
into
|ψ
=
1
ψ|M
+
m
M
m
|ψ
M
m
|ψ, (17.73)
while the ancilla state |k is transformed into |m. In the rest of this book, we will not
have to be concerned about the ancilla space M. Such a space is just used here as a
mathematical tool to allow the construction of a quantum measurement operator for joint
states. Bearing this in mind, we can now expand the conclusion to measurements of joint
states of any dimension from V
⊗n
⊗ M
⊗n
, by means of the n-ancilla space, M
⊗n
, i.e.,
|ψ
1
,ψ
2
,...,ψ
n
, k
1
, k
2
,...,k
n
=|ψ
1
⊗|ψ
2
⊗···⊗|ψ
n
⊗|k
1
⊗k
2
⊗···⊗|k
n
.
.(17.74)
We, thus, know that it is possible to define a measurement operator E
im
that can act on
the subspace pair |ψ
i
, |k
i
and collapse it into the pair M
m
|ψ
i
, |m, such that
E
im
|ψ
1
,ψ
2
,...,ψ
i
,...,ψ
n
, k
1
, k
2
,...,k
i
,...,k
n
=
|ψ
1
⊗|ψ
2
⊗···⊗
M
m
ψ
i
|M
+
m
M
m
|ψ
i
|ψ
i
⊗···|ψ
n
⊗|k
1
⊗k
2
⊗···⊗|k
i−1
⊗|m⊗|k
i+1
⊗···|k
n
.
(17.75)
We may just forget now about the ancilla bits and consider simply that
E
im
|ψ
1
,ψ
2
,...,ψ
i
,...,ψ
n
≡|ψ
1
⊗|ψ
2
⊗···⊗
M
m
ψ
i
|M
+
m
M
m
|ψ
i
|ψ
i
⊗···⊗|ψ
n
.
(17.76)
To provide an illustration, assume that M
m
=|mm|. Letting |ψ
i
=
n
x
(i)
n
|n,we
obtain
E
im
|ψ
1
,...,ψ
n
=|ψ
1
⊗|ψ
2
⊗···⊗
x
(i)
m
+
+
x
(i)
m
+
+
|m⊗···⊗|ψ
n
=
x
(i)
m
+
+
x
(i)
m
+
+
|ψ
1
,...,ψ
i−1
, m,ψ
i+1
,...,ψ
n
≡|ψ
1
,...,ψ
i−1
, m,ψ
i+1
,...,ψ
n
,
(17.77)
as obtained within an unobservable phase factor e
iδ
= x
(i)
m
/|x
(i)
m
|.
Finally, consider for simplicity two joint states in 2-state space V ⊗ V , namely |φ=
|φ
1
,φ
2
and |ψ=|ψ
1
,ψ
2
, and their superposition |χ with complex coefficients α, β
(satisfying |α|
2
+|β|
2
= 1):
|χ=α|φ+β|ψ=α|φ
1
,φ
2
+β|ψ
1
,ψ
2
. (17.78)
Owing to the linearity and distributiveness of the measurement operators, we have
E
1
|χ=E
1
(α|φ+β|ψ) = αE
1
|φ
1
,φ
2
+β E
1
|ψ
1
,ψ
2
=α|1,φ
2
+β|1,ψ
2
E
2
|χ=E
2
(α|φ+β|ψ) = αE
2
|φ
1
,φ
2
+β E
2
|ψ
1
,ψ
2
=α|φ
1
, 2+β|ψ
1
, 2
(17.79)

17.4 Exercises 355
and
E
1
E
2
|χ=E
2
E
1
|χ=α|1, 2+β|1, 2≡(α + β)|1, 2, (17.80)
which actually illustrates the great simplicity of joint-state measurements and their
properties. These can now be applied to the case of single and multiple qubit systems,
which are described in Chapter 18.
17.4 Exercises
17.1 (B): Calculate the moduli and scalar products of the 2-qubits
|q=i|00+|01−|11,
|q
=|00+|01−i|10+|11.
17.2 (B): Show that |qq| is the projector operator over the qubit |q.
17.3 (B): Determine the eigenstates and diagonal representations of the Pauli matrix
Y and Hadamard matrix H .
17.4 (T): Show that the transition operator T defined by its matrix element
T
xy
=x|y
in different orthogonal bases V
n
={|x} and W
n
={|y} satisfies the property
T
+
T = TT
+
= I.
Then show that T = T
+
in the general case.
17.5 (M): Show that two successive measurements from different operators M
m
and
L
l
(in this order) are equivalent to a single measurement of operator definition
K
lm
= L
l
M
m
.
17.6 (T): Show that the three operators {E
1
, E
2
, E
3
} defined by
E
1
= u|11|,
E
2
= v(|0−|1)(0|−1|),
E
3
= I − E
1
− E
2
,
form a complete POVM set over the space V ={|0, |1} if the constants u,v
satisfy the two conditions
0 < u ≤
2
3
,v=
u
2
.

18 Qubit measurements, superdense
coding, and quantum teleportation
This chapter is concerned with the measure of information contained in qubits. This can
be done only through quantum measurement, an operation that has no counterpart in the
classical domain. I shall first describe in detail the case of single qubit measurements,
which shows under which measurement conditions “classical” bits can be retrieved. Next,
we consider the measurements of higher-order or n-qubits. Particular attention is given
to the Einstein–Podolsky–Rosen (EPR) or Bell states, which, unlike other joint tensor
states, are shown being entangled. The various single-qubit measurement outcomes from
the EPR–Bell states illustrate an effect of causality in the information concerning the
other qubit. We then focus on the technique of Bell measurement, which makes it possible
to know which Bell state is being measured, yielding two classical bits as the outcome.
The property of EPR–Bell state entanglement is exploited in the principle of quantum
superdense coding, which makes it possible to transmit classical bits at twice the classical
rate, namely through the generation and measurement of a single qubit. Another key
application concerns quantum teleportation. It consists of the transmission of quantum
states over arbitrary distances, by means of a common EPR–Bell state resource shared
by the two channel ends. While quantum teleportation of a qubit is instantaneous, owing
to the effect of quantum-state collapse, it is shown that its completion does require
the communication of two classical bits, which is itself limited by the speed of light.
We then briefly consider the possibility of denser teleportation schemes, taking, for
example, the case of two qubits. Quantum entanglement is also shown to be applicable
to the teleportation quantum gates. This opens the perspective of distributed quantum
computing: the possibility to manipulate qubits from a distance, or, in a futuristic view,
to share the resources of remote quantum networks.
18.1 Measuring single qubits
This section describes the effect of quantum measurements on qubits. We shall first
recall some key results obtained in previous chapters, and apply the principles to the
case of single qubits and then joint qubit states, including the intriguing case of entan-
gled qubits.
In Chapter 17, we learnt that given a space of quantum states |ψ defined
by an orthonormal base V
n
={|x
i
}, there exist different possibilities of quantum-
measurement operator sets, called {M
m
}, corresponding to a number n of possible
