Blake A.J.(ed.) Crystal Structure Analysis

Подождите немного. Документ загружается.

72 Background theory for data collection

Exercises

1) State which of the following represent real-space or

reciprocal-space quantities:

(a) the structure factor, F;

(b) a space in which Miller indices, h, k, l are labelled;

(d) unit cell parameters, a, b, c, α, β, γ ;

(e) the representation of a part of a crystal structure

via a 2D diffraction pattern;

(f) diffractometer axes, x, y, z .

2) Below are the crystal data for a given compound.

Mo, M

= 476.54, orange spherical crystal

(0.4 mm diameter), monoclinic, space group C2/c, a =

20.240(2), b = 6.550(1), c = 19.910(4) Å, β =

90.101(3)

◦

, V = 2640.4(3) Å

, T = 150 K. 2253 unique

reﬂections were measured on a Bruker SMART CCD

area diffractometer, using graphite-monochromated

MoK

radiation (λ = 0.71073 Å). Lorentz and polar-

izationcorrectionswereapplied.Absorptioncorrections

were made by Gaussian integration using the calculated

attenuation coefﬁcient, μ = 0.44 mm

−1

. The struc-

ture was solved using direct methods and reﬁned by

full-matrix least-squares reﬁnement using SHELXL97

with 2253 unique reﬂections. During the reﬁnement,

an extinction correction was applied. Reﬁnement of

302 positional and anisotropic displacement parame-

ters converged to R

[I > 2σ(I)]=0.1654 and wR

[I >

2σ(I)]=0.3401 [w = 1/σ

)

] with S = 2.31

and residual electron density, ρ

min / max

=−5.43/4.30

eÅ

−3

(a) Calculate F(000).

(b) Using Bragg’s law, calculate d when the detector

lies at 2θ = 20

◦

construction, and the cosine rule to derive the

value of d.

(d) What percentage of the X-ray beam is absorbed by

the crystal? (Assume that, on average, the X-ray

path through a crystal diffracts at its centre).

(e) When indexing the crystal, the experimenter

could not be sure if the crystal was orthorhombic

or monoclinic. Given this, which crystal system

should the experimenter assume when setting up

the data-collection strategy? Explain why.

(f) The residual electron density is signiﬁcant;

indeed, the reﬁned model is poor. Assuming

that the problem lay at the data-reduction stage,

describe possible causes for this.

3) From the orientation matrix:

A =

⎛

⎝

0 0.250 0

0.125 0 0

00−0.100

⎞

⎠

calculate the unit cell parameters. About which axis is

the crystal mounted? Is this desirable?

Practical aspects of

data collection

Alexander Blake

6.1 Introduction

Unlike all other stages of a structure determination, the collection of

diffraction data occurs in real time, requiring the continuous and exclu-

sive use of valuable equipment. Whereas a reﬁnement that has gone

wrong can usually be repeated without causing signiﬁcant delay, an

abandoned or terminally ﬂawed data collection has wasted instrument

time irrevocably. The aim in collecting a dataset should always be to

obtain the best possible quality of data from the available sample within

a reasonable length of time. This requires not only preparing or demand-

ing the best possible crystal, a topic that is covered in Chapter 3, but also

choosing appropriate experimental conditions and parameters.

6.2 Collecting data with area-detector

diffractometers

Until the mid-1990s, nearly all single-crystal data collection for chemi-

cal crystallography applications was carriedout using four-circle(serial)

diffractometers. After a slow start when image plate (IP) area-detector

systems made some impact, the ﬁrst commercial area-detector instru-

ments based on charge-coupled devices (CCDs) were introduced in

1994. These have since become widely available from a number of sup-

pliers. They have dropped markedly in price and have now totally

displaced the four-circle instrument as the standard workhorse for data

collection. Having said this, the large installed base of serial instruments

means that, worldwide, they will be a declining source of diffraction

data for some time. The replacement of single-point by area detectors

has brought with it both advantages and disadvantages, but for most

purposes the former greatly outweigh the latter. They are summarized

below.

74 Practical aspects of data collection

Advantages:

+ simultaneous recording of many reﬂections,

+ faster data collection possible,

+ data-collection time independent of structure size,

+ high redundancy of symmetry-equivalent data possible,

+ rapid screening of samples,

+ not necessary to obtain correct orientation matrix and unit cell

before data collection,

+ complete diffraction pattern measured, not just around Bragg

reﬂection positions,

+ reduced probability of obtaining incorrect cell from a few initially

found reﬂections,

+ poor crystal quality and weaker diffraction can often be tolerated,

+ minimal crystal movement necessary, so easier to use low-

temperature and other accessories,

+ easy visualization of the diffraction pattern, so good for teaching

and training,

+ can obtain data on twinned or incommensurate crystals.

Disadvantages:

− possibly high capital and maintenance costs,

− high computing requirements, especially processing power and

data storage,

− need for careful corrections for non-uniformities and other effects,

− usually poor discrimination against other X-ray wavelengths, e.g.

harmonics,

− restricted detector size may lead to problems with large unit cells

and with Cu Kα X-rays,

− it may be expensive and difﬁcult to change radiation,

− upper limit on counting time per frame for CCD detectors,

− they are not efﬁcient for very small cells.

The major obvious feature of an electronic area detector is its ability to

record diffraction data over a substantial solid angle. As far as normal

Bragg diffraction is concerned, this means the simultaneous measure-

ment of a number of reﬂections: the number of reﬂections measured

simultaneously depends on the size of the unit cell as well as on the size

of the detector.In addition, an areadetector actuallyrecords the wholeof

the intercepted diffraction pattern and not just the Bragg reﬂections (i.e.

the whole of reciprocal space is observed, not just the regions immedi-

ately around each reciprocal lattice point). This can be useful for special

purposes, such as thedetection of twinning orthe study of incommensu-

rate structures. A related advantage is that it is not actually necessary to

establish the correctunit cell and crystal orientation matrix beforebegin-

ning data collection – although it is highly advisable to do so whenever

possible – because these can be found later from the stored images. (An

invalid orientation matrix on a four-circle diffractometer usually means

a useless set of data, because only the predicted reﬂection positions are

6.3 Experimental conditions 75

explored.) A corollary of this is the ability, where deemed necessary, to

re-process stored images using alternative indexing and/or peak proﬁle

parameters.

6.3 Experimental conditions

6.3.1 Radiation

The crystallographer may have some choice over the conditions under

which data are collected and one of these is the wavelength of the

radiation used, the most common choice in the home laboratory being

between copper (1.54184 Å) and molybdenum (0.71073 Å). Copper X-

ray tubes produce a higher ﬂux of incident photons (for the same power

settings) and these are diffracted more efﬁciently than molybdenum

radiation: copper radiation is therefore particularly useful for small or

otherwise weakly diffracting crystals, especially if absorption effects are

moderate. In addition, focusing optics provide greater enhancements

for the longer wavelength. For crystals with long unit cell dimensions,

reﬂections are further apart when the longer-wavelength copper radi-

ation is used and this can minimize reﬂection overlap. If you need to

determine the absolute conﬁguration, and your crystals do not contain

elements heavier than, say, silicon, then copper radiation is essential.

On the other hand, absorption effects are generally less serious with

molybdenum radiation and this can be crucial if elements of high

atomic number are present. Molybdenum radiation allows collection

of data to higher resolution and is likely to cause fewer restrictions if

low-temperature or other attachments are required. Changing radiation

requiressomeeffortand skill and you lose data-collection time. Theideal

situation is to have two diffractometers, one equipped with each radia-

tion, and a supply of suitable crystals so that both may be fully utilized.

Some diffractometer manufacturers offer hybrid Cu/Mo instruments

with two different X-ray tubes and their associated optics but only one

area detector: switching radiation takes little time and is automated, and

there is substantial cost saving because the most expensive component

of the diffractometer (the detector) is not duplicated.

The following illustrative examples may be helpful:

a well-diffracting organic compound containing iodine:

use Mo to minimize absorption

a poorly diffracting organic compound (CHNO):

use Cu to maximize diffracted intensity

an organic compound (CHNO) with b > 50 Å:

use Cu to minimize overlap

absolute conﬁguration on C

feasible only with Cu

most metal complexes, etc.

use Mo to minimize absorption

high-resolution studies

use Mo

76 Practical aspects of data collection

a weakly diffracting platinum complex

a ‘no-win’ situation?

Where a sample diffracts too weakly on a laboratory source to yield

enough observable data, and it is considered important enough, it could

be taken to a synchrotron radiation facility. In this event several advan-

tages can accrue: the wavelength can be tuned speciﬁcally to the needs

of the sample; the intensity typically represents at least a 100-fold gain

over that of a laboratory source; the resolution between reﬂections is also

generally greater. The disadvantage is that there is heavy competition

for such a rare resource and there is often a long wait for synchrotron

beam time. For this reason, it is worth considering whether more mod-

est enhancement would overcome the problem of weak diffraction: a

longer wavelength, a lower temperature or a more powerful laboratory

source may sufﬁce.

Lastly, when considering the most appropriate wavelength for a

particular experiment, you should also bear in mind the physical restric-

tions that will preclude data collection in some areas of reciprocal space:

these limitations include where the beam stop is positioned and where

accessories such as low-temperature devices, cryostats or high-pressure

cells are deployed. The restrictions are generally much more serious at

longer wavelengths, since higher 2θ values are required to achieve the

same resolution.

6.3.2 Temperature

If a reliable low-temperature system is available it is almost always

worthwhile considering data collection at low temperature (most low-

temperature devices will even produce slightly elevated temperatures

if this is required for a special experiment, while some have extended

upper temperature limits). The beneﬁts of low-temperature data collec-

tion can only be realized if the equipment is well aligned and correctly

set up: for example, icing can lead to the crystal moving or even being

lost. Reducing the temperature of the crystal can have many advan-

tages and is essential for crystals mounted using protective oil ﬁlms,

compounds melting below about 50

◦

C and those that are thermola-

bile. Reactive compounds may be stabilized long enough to allow data

collection. There are general advantages: at lower temperatures atomic

displacements are reduced and the intensities of reﬂections at higher

Bragg angles thereby enhanced, allowing the collection of better diffrac-

tion data at higher resolution. This reduction also minimizes librational

effects that can otherwise give artiﬁcially shortened bond lengths and

other systematic errors. One advantage of the reduction in temperature

is the relative ease with which disorder can be modelled: for example,

popular pseudo-spherical anions such as BF

−

,PF

−

, ClO

−

and SO

−

are

often badly disordered at room temperature but are either ordered or

their disorder is much easier to model at low temperature. The actual

temperature chosen is usually a compromise between the desire for the

lowest temperature and the increased risk of icing as the temperature

6.4 Types of area detector 77

is reduced. For routine work on molecular crystals, temperatures in the

range 100–200 K are typical. Phase changes appear to be a relatively rare

problem but cooling can have adverse effects on poor-quality crystals,

showing up in the splitting of reﬂections, in poor orientation matrices

and larger uncertainties on cell parameters. Sometimes the splitting can

be annealed out by increasing the temperature, for example from 150 K

to200K.Evenin these apparently unfavourablecasesalow-temperature

determination is often better than one at ambient temperature. The fact

that cooling methods typically involve rapid freezing of crystals on the

diffractometeropens up the question of what proportionof such crystals

are determined as metastable rather than as equilibrium phases.

6.3.3 Pressure

Although it is not used in routine experiments, the application of high

pressures provides a means of inducing structural change, including the

generation of new polymorphs that are inaccessible by any other means.

Atypical experiment involves applying pressure to material held within

a high-pressure cell, then studying the product in situ. Such experiments

are challenging, not least because the components of the high-pressure

cell severely limit how much of the sample’s diffraction pattern can be

recorded: these components also contribute strongly to the observed

diffraction pattern. Notable recent advances have included the appli-

cation to chemical crystallography of techniques originally developed

for mineralogy and physical crystallography (see for example Dawson

et al., 2004; Allan et al., 2006). This has recently been recognized by an

issue of Chemical Society Reviews (McMillan, 2006).

6.3.4 Other conditions

Considerations of crystal size, methods of mounting, choice of goniome-

ter head and optical centring have been mentioned previously but are

worth stressing again as they can seriously affect the outcome of the

experiment. The collimator selected should allow the entire crystal to

be immersed in the X-ray beam but its diameter should not be exces-

sive, as this will contribute to scattering by the air, resulting in increased

background levels. This scattering is more serious with copper than

with molybdenum radiation. The effects of an oversized crystal are also

strongly dependent on the elements in the sample: it has been shown

that with only light elements (e.g., Z ≤ 8) present, the use of large

(∼2 mm) crystals does not cause serious problems and may be beneﬁ-

cial because of the enhanced intensities that can be measured, or because

the data-collection time can be reduced (Görbitz, 1999).

6.4 Types of area detector

6.4.1 Multiwire proportional chamber (MWPC)

In proportional counters each incident X-ray photon causes an ioniza-

tion in the detector gas, and hence a current in a high-potential wire.

78 Practical aspects of data collection

MWPCs usually have one set of parallel wires and a second set at right

angles, and a current is induced in one or more wires of each set for

each X-ray photon. Thus, both the time and position at which the photon

arrives areknown. The MWPC has the advantages over other areadetec-

tors that the output signal is instantaneous (time resolution is ideal),

there is no inherent noise in the detector, and the counting efﬁciency is

high. There is, however, a problem with parallax, particularly at shorter

wavelengths, reducing the spatial precision, and the overall count rate

is limited by the dead time, which affects the detector as a whole for

every single recorded photon. MWPCs have not been used signiﬁcantly

for chemical crystallography.

6.4.2 Phosphor coupled to a TV camera

The phosphor ﬁrst converts incident X-rays to visible light. Fibre optics

provide the coupling that takes this light from the phosphor to the

low-light-level TV camera where it is detected. This type of detector

also gives an instantaneous readout, but the active area is relatively

small, as is the dynamic range, and the signal-to-noise ratio is poorer

than for other area detectors. The TV system most widely used was the

Enraf-Nonius FAST; its principal application for chemical crystallogra-

phy was in the EPSRC National Crystallography Service at Cardiff up to

1998. It is no longer commercially available, and is therefore of historical

interest only.

6.4.3 Image plate (IP)

Instead of converting X-rays to visible light, the phosphor in these

devices stores the image in the form of trapped electron colour cen-

tres. These are later ‘read’ by stimulation from visible laser light (which

causes them to emit their own characteristic light for detection by a

photomultiplier) and then erased by strong visible light before another

exposure to X-rays. The main advantages of image plates are that they

are available in large sizes and are relatively inexpensive; they also have

a high recording efﬁciency and a high spatial resolution (Fig. 6.1). The

one major disadvantage is the need for a separate read-out process,

which requires minutes rather than seconds. Faster image-plate systems

offeronesolutionbutanother method of increasingthe time available for

X-ray exposure is the use of two or more plates, so that one is recording

an image while another is being read: however, this adds considerably

to the cost and complexity.

6.4.4 Charge-coupled device (CCD)

This type of detector, more familiar in video cameras and other mass-

market applications, is a semiconductor in which incident radiation

produces electron-hole pairs; the electrons are trapped in potential wells

and then read out as currents. For various reasons, direct recording of

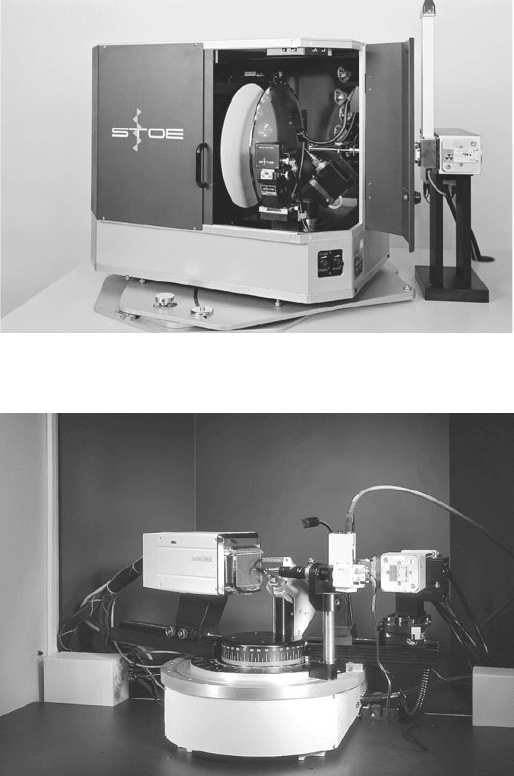
6.4 Types of area detector 79

Fig. 6.1 An image-plate system.

Fig. 6.2 A CCD area-detector diffractometer.

X-rays is not usually carried out: instead, a phosphor is coupled through

ﬁbre optics to the CCD chip, which is cooled to reduce the inherent elec-

tronic noise level due to thermal excitation of electrons. Efﬁcient record-

ing, a high dynamic range and a low noise level, and a read-out time

measured in seconds or fractions of a second, combine to give the CCD

some clear advantages as a rapid area detector, but the size is limited

by the size and quality of chips available. Of the area-detector technolo-

gies now in use, this has the best potential for further development,

and considerable effort is being made in producing larger and more

sensitive chips without signiﬁcantly increasing the read-out time. Com-

mercial CCD systems for chemical crystallography are now available

from several major ﬁrms (Fig. 6.2). The next few years are likely to see

80 Practical aspects of data collection

the wider use of so-called ‘pixel arrays’, another form of solid-state

detector that should be able to record X-rays directly (i.e. without con-

version to visible light) and over a larger area. These detectors are under

active development (see for example http://pilatus.web.psi.ch/pilatus.htm)

and have a number of highly attractive features including no read-

out noise, excellent S/N ratio, no dark current (so no need to cool the

detector), read-out times of a few milliseconds per frame, very high

(20 bit) dynamic range, high quantum efﬁciency, energy discrimina-

tion and ﬂuorescence suppression. The X-ray shutter is open during

the collection, with ﬁne time slicing being done by the detector elec-

tronics, enabling rapid data collections (e.g., 4 s) and time-resolved

studies.

Area detectors do not just offer the possibility of collecting diffraction

data more quickly, although that is generally perceived as their main

advantage. They also make feasible experiments that are beyond the

scope of the old serial diffractometers, through higher sensitivity and

the recording of the whole pattern. Much of the following applies to any

type of area detector, but it will refer speciﬁcally to CCD systems, since

these are the most widely used in chemical crystallography.

6.5 Some characteristics of CCD

area-detector systems

An area detector, whether based on a CCD or an alternative technol-

ogy, is only one component of a single-crystal X-ray diffractometer. It

needs to be combined with a goniometer for mounting and moving the

crystal sample, a source of X-rays, and electronic and computing control

systems.

Although an area detector records a number of diffracted beams

simultaneously, it is still necessary to rotate the crystal in the X-ray beam

in order to access all the available reﬂections. In most chemical crystal-

lography systems, the detector is offset to one side rather than being

held perpendicular to the incident X-ray beam, so that a higher maxi-

mum Bragg angle can be observed for a single detector position. Typical

designs and conﬁgurations give data to a maximum Bragg angle θ of

around 25−30

◦

, appropriate for a ‘routine’ structure determination with

a more than adequate data/parameter ratio if Mo-Kα radiation is used.

The two-dimensional nature of the detector means that it is not neces-

sary to bring all reﬂections into a horizontal plane (assuming the ω-axis

is considered vertical and the incident X-ray beam horizontal), so less

movement of the crystal is required in order to access all reﬂections. The

relative advantages and disadvantages of different goniometer designs,

with one, two or three available rotations for the crystal, can be debated.

However, with only one rotation axis, for some low-symmetry crystal

systems not all reﬂections may be measurable with the particular crys-

tal orientation chosen. There is also no general agreement about the

6.5 Some characteristics of CCD area-detector systems 81

choice of rotation axis. Not having a full χ-circle does give consider-

ably more freedom for attachments such as low-temperature devices or

high-pressure cells.

Corrections for a number of factors need to be applied to raw CCD

images. These are usually fully integrated into commercial systems.

Some of the corrections are listed here.

6.5.1 Spatial distortion

The demagniﬁcation of the diffraction image from the phosphor to

the CCD chip via the ﬁbre-optic taper used in many systems is never

perfectly linear and to scale. The mapping of CCD pixels to original

face-plate positions needs to be calibrated and a correction then applied

to every image. The calibration can be made, for example, by recording

a pattern of X-rays from an amorphous scatterer or a ﬂuorescent sample

through an accurately machined grid of ﬁne holes placed over the detec-

tor face. It is valid until the phosphor-taper-CCD assembly is changed

in some way.

6.5.2 Non-uniform intensity response

Equal incident X-ray intensity at different points on the detector face

may lead to unequal numbers of electrons at the corresponding pixels

on the CCD, for various reasons involving the different components of

the system. Calibration involves recording a uniform intensity ‘ﬂood

ﬁeld’ and measuring the CCD image for this.

6.5.3 Bad pixels

Minor faults in CCD production can include individual pixels or even

rows of pixels that do not respond correctly to incident light. Substantial

faults mean an unusable chip, but a few bad pixels can be tolerated and

ﬂagged as bad, particularly in systems in which pixels are ‘binned’ by

combining 2 × 2 or other groups of pixels rather than using all pixels

individually.

6.5.4 Dark current

Thermal excitation leads to the generation and trapping of electrons in

the CCD pixel wells even when there is no incident light, and this slowly

builds up a background ‘dark image’ on the detector, which will be read

out superimposed on the true image. The effect is minimized by cooling

the CCD (typically to a temperature between −45 and −80

◦

C) and can

be corrected by recording a dark-current image (i.e. without any X-rays)

for the same time as a normal exposure and subtracting this from each

measured frame. The dark-current image is temperature dependent and

needs to be measuredfor theappropriatelength of time, preferably aver-

aged over multiple recordings to reduce statistical ﬂuctuations. Even