Articolo G.A. Partial Differential Equations and Boundary Value Problems with Maple V
Подождите немного. Документ загружается.

This page intentionally left blank

CHAPTER 6
The Diffusion Equation in Two
Spatial Dimensions
6.1 Introduction
In Chapter 3, we examined the heat or diffusion partial differential equation in only one spatial
dimension. Here, we examine the diffusion equation in two spatial dimensions. Thus, we will
be considering problems with a total of three independent variables: the two spatial variables
and time. The method of separation of variables will be used, and we shall see the development
of two independent Sturm-Liouville problems—one for each of the two spatial variables. The
final solutions will have the form of a double Fourier series. We will consider problems in both
the rectangular and the cylindrical coordinate systems.
6.2 Two-Dimensional Diffusion Operator in Rectangular
Coordinates
In the one-dimensional diffusion equation, as discussed in Chapter 3, the second-order partial
derivative with respect to the spatial variable x can be viewed as the first term of the Laplacian
operator. Thus, an extension of this Laplacian operator to higher dimensions is natural, and it
can be shown that heat or diffusion phenomena in two dimensions can be described by the
following nonhomogeneous partial differential equation in the rectangular coordinate system:
∂
∂t
u(x, y, t) = k
∂
2
∂x
2
u(x, y, t) +
∂
2
∂y
2
u(x, y, t)
+h(x, y, t)
where, for the situation of heat phenomena, u(x, y, t) denotes the spatial-time-dependent
temperature and the constant k, called “thermal diffusivity,” incorporates the thermal
characteristics of the medium such as thermal conductivity, specific heat, and mass density.
The term h(x, y, t) accounts for the time-rate of input of any external heat source into the
medium. We have assumed the medium to be isotropic and uniform so that the preceding
thermal coefficients are spatially invariant, and we treat them as constants.
© 2009, 2003, 1999 Elsevier Inc. 339

340 Chapter 6
Most of the example problems that we will be considering deal with thin plates with no
extension along the z-axis and whose lateral surfaces are insulated; thus, we have no
z-dependent terms in the Laplacian.
The partial differential equation for diffusion or heat phenomena is characterized by the fact
that it relates the first partial derivative with respect to the time variable with the second partial
derivatives with respect to the spatial variables.
We can write this nonhomogeneous equation in terms of the linear heat or diffusion operator as
L(u) = h(x, y, t)
The diffusion operator in rectangular coordinates in two spatial dimensions reads
L(u) =
∂
∂t
u(x, y, t) −k
∂
2
∂x
2
u(x, y, t) +
∂
2
∂y
2
u(x, y, t)
If there are no external heat sources in the system, then h(x, y, t) = 0, and the preceding
nonhomogeneous partial differential equation reduces to its corresponding homogeneous
equivalent
L(u) = 0
A typical homogeneous diffusion problem in two spatial dimensions reads as follows. We seek
the temperature distribution u(x, y, t) in a thin rectangular plate over the finite two-dimensional
domain D ={(x, y) |0 <x<1, 0 <y<1}. The lateral surfaces of the plate are insulated, so
there is no heat loss through the lateral surface. The boundaries y = 0 and y = 1 are fixed at
temperature zero, the boundary x = 0 is insulated, and the boundary x = 1 is losing heat by
convection into a surrounding medium at temperature zero. There are no heat sources in the
system, the initial temperature distribution f(x, y) is given following, and the thermal
diffusivity of the plate material is k = 1/10.
The diffusion homogeneous partial differential equation reads
∂
∂t
u(x, y, t) =
∂
2
∂x
2
u(x, y, t) +
∂
2
∂y
2
u(x, y, t)
10
The given boundary conditions on the problem are type 2 at x = 0, type 3 at x = 1, type 1 at
y = 0, and type 1 at y = 1.
u
x
(0,y,t)= 0 and u
x
(1,y,t)+u(1,y,t)= 0
and
u(x, 0,t)= 0 and u(x, 1,t)= 0

The Diffusion Equation in Two Spatial Dimensions 341
The initial condition function is
u(x, y, 0) = f (x, y)
We now examine the general procedures for the solution to this homogeneous partial
differential equation over finite domains, with homogeneous boundary conditions, using the
method of separation of variables.
6.3 Method of Separation of Variables for the Diffusion
Equation in Two Dimensions
The homogeneous diffusion equation for a uniform system in two dimensions in rectangular
coordinates can be written
∂
∂t
u(x, y, t) −k
∂
2
∂x
2
u(x, y, t) +
∂
2
∂y
2
u(x, y, t)
= 0
This can be rewritten in the more familiar form
∂
∂t
u(x, y, t) = k
∂
2
∂x
2
u(x, y, t) +
∂
2
∂y
2
u(x, y, t)
Generally, one seeks a solution to this problem over the finite two-dimensional rectangular
domain D ={(x, y) |0 <x<a,0 <y<b} subject to the homogeneous boundary conditions
κ
1
u(0,y,t)+κ
2
u
x
(0,y,t)= 0
κ
3
u(a, y, t) +κ
4
u
x
(a,y,t)= 0
κ
5
u(x, 0,t)+κ
6
u
y
(x, 0,t)= 0
κ
7
u(x, b, t) +κ
8
u
y
(x,b,t)= 0
and the initial condition
u(x, y, 0) = f(x, y)
In the method of separation of variables, as done in Chapter 3, the character of the equation is
such that we can assume a solution in the form of a product as
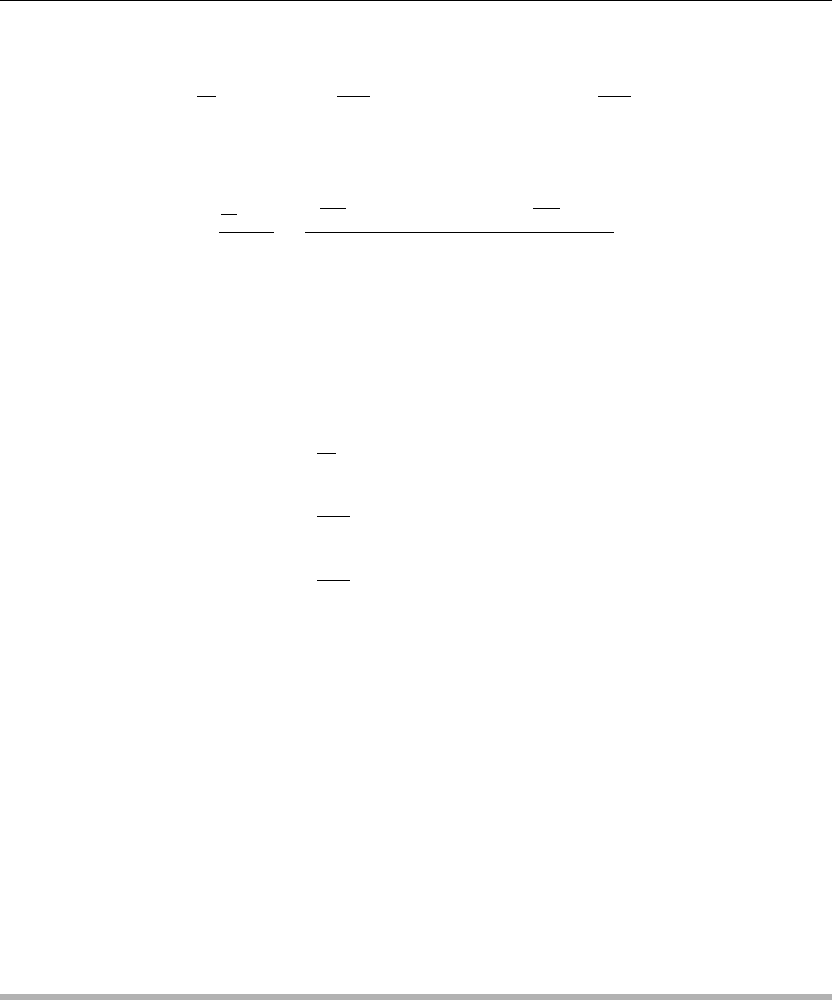
u(x, y, t) = X(x)Y(y)T(t)
Because the three independent variables in the partial differential equation are the spatial
variables x and y, and the time variable t, then the preceding assumed solution is written as a
product of three functions; one is an exclusive function of x, one is an exclusive function of y,
and the last an exclusive function of t.
342 Chapter 6
Substituting this assumed solution into the partial differential equation gives
X(x) Y(y)
d
dt
T(t)
= k
d
2
dx
2
X(x)
Y(y) T(t) +X(x)
d
2
dy
2
Y(y)
T(t)
Dividing both sides of the preceding by the product solution yields
d
dt
T(t)
kT(t)
=
d
2
dx
2
X(x)
Y(y) +X(x)
d
2
dy
2
Y(y)
X(x)Y(y)
Because the left-hand side of this equation is an exclusive function of t and the right-hand side
an exclusive function of both x and y, and the variables x, y, and t are independent, then the
only way the preceding equation can hold for all values of x, y, and t is to set each side equal to
a constant. Doing so, we get the following three ordinary differential equations in terms of the
separation constants λ,α, and β:
d
dt
T(t) +kλ T(t) = 0
d
2
dx
2
X(x)+αX(x)= 0
d
2
dy
2
Y(y) +βY(y)= 0
with the coupling equation
λ = α +β
The preceding differential equation in t is an ordinary first-order linear homogeneous
differential equation for which we already have the solution from Section 1.2. The second
and third differential equations in x and y are ordinary second-order linear homogeneous
differential equations of the Euler type for which we already have the solutions from
Section 1.4. We easily recognize each of the two spatial differential equations as being
Sturm-Liouville types.
6.4 Sturm-Liouville Problem for the Diffusion Equation
in Two Dimensions
The second and third differential equations given in the previous section, in the spatial
variables x and y, each must be solved subject to their respective homogeneous spatial
boundary conditions.
We first consider the x-dependent differential equation over the finite interval
I ={x |0 <x<a}. The Sturm-Liouville problem for the x-dependent portion of the diffusion

The Diffusion Equation in Two Spatial Dimensions 343
equation consists of the ordinary differential equation
d
2
dx
2
X(x)+αX(x)= 0
along with the respective corresponding regular homogeneous boundary conditions, which
read as
κ
1
X(0) +κ
2
X
x
(0) = 0
and
κ
3
X(a)+κ
4
X
x
(a) = 0
We recognize this problem in the spatial variable x to be a regular Sturm-Liouville eigenvalue
problem where the allowed values of α are called the system “eigenvalues” and the
corresponding solutions are called the “eigenfunctions.” Note that this ordinary differential
equation is of the Euler type and the weight function is w(x) = 1. The indexed eigenvalues and
corresponding eigenfunctions are given, respectively, as
α
m
,X
m
(x)
for m = 0, 1, 2, 3,....
These eigenfunctions can be normalized and the corresponding statement of orthonormality,
with respect to the weight function w(x) = 1, reads
a
0
X
m
(x)X
r
(x) dx = δ(m, r)
Here, δ(m, r) is the Kronecker delta function defined earlier. We now focus on the third
differential equation in the spatial variable y subject to the homogeneous spatial boundary
conditions over the finite interval I ={y |0 <y<b}. The Sturm-Liouville problem for the
y-dependent portion of the diffusion equation consists of the ordinary differential equation
d
2
dy
2
Y(y) +βY(y)= 0
along with the respective corresponding regular homogeneous boundary conditions, which
read
κ
5
Y(0) +κ
6
Y
y
(0) = 0
and
κ
7
Y(b) +κ
8
Y
y
(b) = 0

344 Chapter 6
Again, we recognize this problem in the spatial variable y to be a regular Sturm-Liouville
eigenvalue problem where the allowed values of β are called the system “eigenvalues” and the
corresponding solutions are called the “eigenfunctions.” Note that this ordinary differential
equation is of the Euler type and the weight function is w(y) = 1. The indexed eigenvalues and
corresponding eigenfunctions are given, respectively, as
β
n
,Y
n
(y)
for n = 0, 1, 2, 3,....
Similar to the x-dependent eigenfunctions, the preceding eigenfunctions can be normalized and
the corresponding statement of orthonormality, with respect to the weight function w(y) = 1,
reads
b
0
Y
n
(y)Y
s
(y)dy = δ(n, s)
Here, δ(n, s) is the Kronecker delta function defined earlier. The statements made in Section
2.2 about regular Sturm-Liouville eigenvalue problems in one dimension can be extended to
two dimensions here; that is, the sum of the products of the eigenfunctions X
m
(x) and Y
n
(y)
form a “complete” set with respect to any piecewise smooth function f(x, y) over the finite
two-dimensional domain D ={(x, y) |0 <x<a,0 <y<b}.
Finally, we focus on the solution to the time-dependent differential equation, which reads
d
dt
T(t) +kλT(t) = 0
From Section 1.2 on first-order linear differential equations, the solution to this time-dependent
differential equation in terms of the allowed eigenvalues λ
m,n
is
T
m,n
(t) = C(m, n) e
−kλ
m,n
t
where, from the coupling equation, we have
λ
m,n
= α
m
+β
n
for m, n = 0, 1, 2, 3,....The coefficients C(m, n) are unknown arbitrary constants.
Thus, by the method of separation of variables, we arrive at an infinite number of indexed
solutions for the homogeneous diffusion partial differential equation, over a finite interval,
given as the product
u
m,n
(x,y,t)= X
m
(x)Y
n
(y)C(m, n) e
−kλ
m,n
t
for m = 0, 1, 2, 3,..., and n = 0, 1, 2, 3,....

The Diffusion Equation in Two Spatial Dimensions 345
Because the partial differential operator is linear, any superposition of solutions to the
homogeneous equation is also a solution to the problem. Thus, we can write the general
solution to the homogeneous partial differential equation as the double-infinite sum
u(x, y, t) =
∞
n=0
∞
m=0
X
m
(x)Y
n
(y)C(m, n) e
−kλ
m,n
t
This is the double Fourier series eigenfunction expansion form of the solution to the partial
differential equation. The terms of the preceding double series form the “basis vectors” of the
solution space of the partial differential equation. Similar to a partial differential equation in
only one dimension, there are an infinite number of basis vectors in the solution space, and we
say the dimension of the solution space is infinite.
DEMONSTRATION: We seek the temperature distribution in a thin rectangular plate over the
finite two-dimensional domain D ={(x, y) |0 <x<1, 0 <y<1}. The lateral surfaces of the
plate are insulated. The boundaries y = 0 and y = 1 are fixed at temperature 0, the boundary
x = 0 is insulated, and the boundary x = 1 is losing heat by convection into a surrounding
medium at temperature 0. The initial temperature distribution f(x, y) is given below, and the
thermal diffusivity of the plate material is k = 1/10.
SOLUTION: The two-dimensional diffusion partial differential equation reads
∂
∂t
u(x, y, t) =
∂
2
∂x
2
u(x, y, t) +
∂
2
∂y
2
u(x, y, t)
10
The given boundary conditions on the problem are type 2 at x = 0, type 3 at x = 1, type 1 at
y = 0, and type 1 at y = 1.
u
x
(0,y,t)= 0 and u
x
(1,y,t)+u(1,y,t)= 0
and
u(x, 0,t)= 0 and u(x, 1,t)= 0
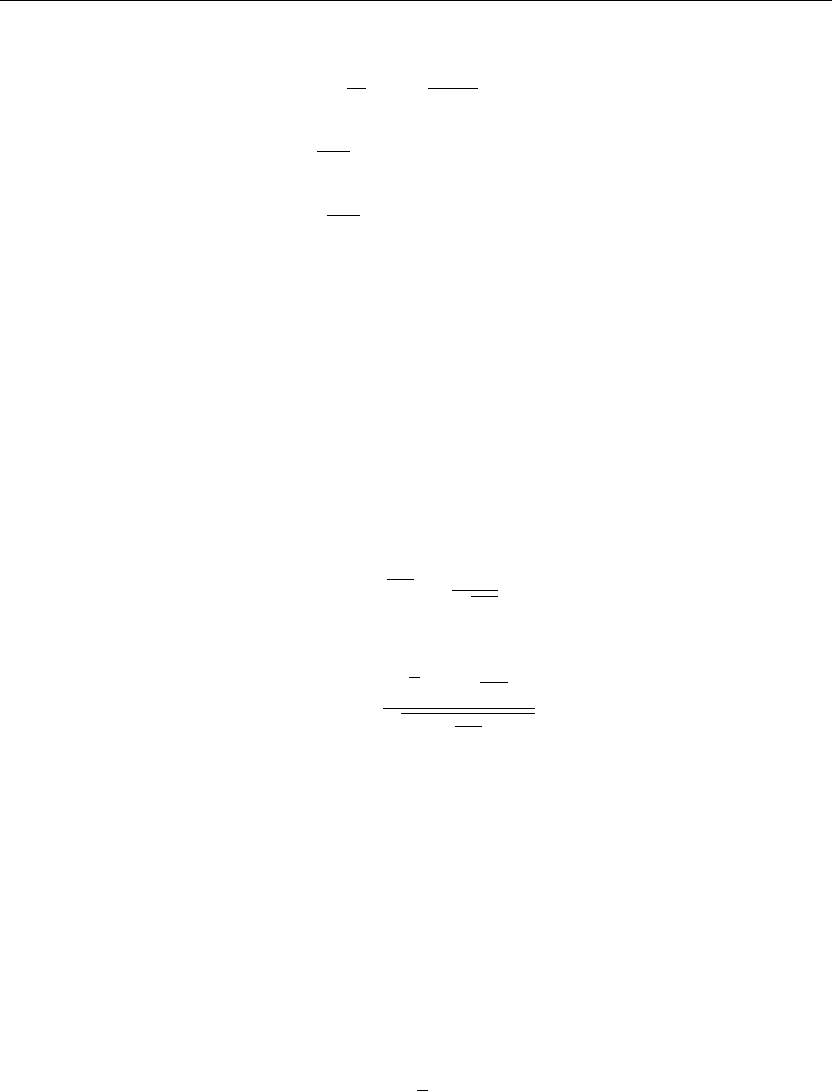
The initial condition function is given as
u(x, y, 0) = f(x, y)
346 Chapter 6
From the method of separation of variables, we obtain the three ordinary differential equations
d
dt
T(t) +
λT(t)
10
= 0
d
2
dx
2
X(x)+αX(x)= 0
d
2
dy
2
Y(y) +βY(y)= 0
with the coupling equation
λ = α +β
We first focus on the solution for the two spatial differential equations. For the x equation, we
have a differential equation of the Euler type, and the corresponding boundary conditions are
type 2 at the left and type 3 at the right
X
x
(0) = 0 and X
x
(1) +X(1) = 0
This same boundary value problem was considered in Example 2.5.5 in Chapter 2. The allowed
eigenvalues are determined from the roots of the equation
tan
√
α
m
=
1
√
α
m
and the corresponding orthonormal eigenfunctions are
X
m
(x) =
√
2 cos
√
α
m
x
sin
√
α
m
2
+1
for m = 1, 2, 3,....
Similarly, for the y equation, we have a differential equation of the Euler type, and the
corresponding boundary conditions are type 1 at the left and type 1 at the right
Y(0) = 0 and Y(1) = 0
The allowed eigenvalues and corresponding orthonormalized eigenfunctions, obtained from
Example 2.5.1 in Chapter 2, are
β
n
= n
2
π
2
and
Y
n
(y) =
√
2 sin (nπy)
for n = 1, 2, 3,....

The Diffusion Equation in Two Spatial Dimensions 347
For each of the two spatial equations, the statements of orthonormality, with their respective
weight functions, are
1
0
2 cos
√
α
m
x
cos
√
α
r
x
sin
√
α
m
2
+1
sin
√
α
r
2
+1
dx = δ(m, r)
and
1
0
2 sin(nπy) sin(sπy) dy = δ(n, s)
Finally, the time-dependent differential equation has the solution
T
m,n
(t) = C(m, n) e
−
λ
m,n
t
10
where
λ
m,n
= α
m
+n
2
π
2
for m = 1, 2, 3,... and n = 1, 2, 3,....The coefficients C(m, n) are arbitrary constants.
The final eigenfunction series expansion form of the solution is constructed from the sum of
the products of the time-dependent solution and the preceding x- and y-dependent
eigenfunctions. Forming this sum, we get
u(x, y, t) =
∞
n=1
⎛
⎜
⎝
∞
m=1
2C(m, n)e
−
λ
m,n
t
10
cos
√
α
m
x
sin(nπy)
sin
√
α
m
2
+1
⎞
⎟
⎠
The coefficients C(m, n) are yet to be determined from the initial condition function f(x, y).
6.5 Initial Conditions for the Diffusion Equation in
Rectangular Coordinates
We now consider evaluation of the coefficients from the initial conditions on the problem. At
time t = 0 we have
u(x, y, 0) = f(x, y)
Substituting this into the following general form of the solution
u(x, y, t) =
∞
n=0
∞
m=0
X
m
(x)Y
n
(y)C(m, n)e
−kλ
m,n
t
