Agoston M.K. Computer Graphics and Geometric Modelling: Mathematics

Подождите немного. Документ загружается.

C.6 The Tensor and Exterior Algebra 879

so that

Because we do not have to worry about parenthesizing tensor products by Theorem

C.6.2(3), this construction generalizes to produce a unique linear transformation

Deﬁnition. The map T

ƒ T

ƒ ···ƒ T

is called the tensor product of the maps T

Now, although it is clear from the deﬁnition that tensor products have to do with

multilinear maps, we want to describe a much more fundamental relationship

between the two. See Theorem C.6.6 below. First of all we shall deﬁne a parallel

algebra structure on multilinear maps.

Deﬁnition. If f Œ L

(V) and g Œ L

(V), then deﬁne f ƒ g Œ L

r+s

(V) by

(C.7)

for v

Œ V. The map f ƒ g is called the tensor product of f and g.

Notation. Let L(V) denote the direct sum of the vector spaces L

(V), k ≥ 0.

C.6.3. Theorem. The tensor product operation ƒ deﬁned by equation (C.7) turns

L(V) into an algebra called the algebra of real-valued multilinear maps on V

Proof. This theorem is the analog of Theorem C.6.2(1–3). One can easily prove that

ƒ is associative and that the distributive laws hold.

C.6.4. Theorem. There is a unique vector space isomorphism

(C.8a)

with the property that

(C.8b)

for all g Œ L

(V) and v

Œ V.

Proof. This is an easy consequence of the universal factorization property of the

tensor product.

C.6.5. Theorem. Let U and V be vector spaces.

(1) There is a unique isomorphism

j :* * *UV UVƒÆƒ

()

vv v v v v

12 1 2

, ,...,

()

ƒ ƒ◊◊◊ƒ

()

y :*,LT

()

fg f g

r r rs r r rs

()( )

++ ++

vv vv v vv v v v

12 1 12 1

, ,..., , ,..., , ,..., ,..., ,

TT T

kk k12 1 2 1 2

ƒ ƒ◊◊◊ƒ ƒ ƒ◊◊◊ƒ Æ ƒ ƒ◊◊◊ƒ:.UU U VV V

TT T T

1212 1 21 2

()()

=ƒ

()

uu u u,.

880 Appendix C Basic Linear Algebra

deﬁned by the condition that

for all aŒU*, bŒV*, u Œ U, and v Œ V.

(2) There is a unique isomorphism

(C.9a)

deﬁned by

(C.9b)

for all a

Œ V* and v

Œ V.

Proof. To prove part (1), note that the universal factorization property of tensor

products implies that j is induced by the bilinear map

(C.10a)

deﬁned by

(C.10b)

To show that j is an isomorphism, show that the vectors j(u

ƒ v

) are linearly inde-

pendent for some dual basis u

for U* and v

for V*. See [AusM63] or [KobN63]. Part

(2) is an easy generalization of part (1).

Because of Theorem C.6.5 we shall not distinguish between tensor products like

U* ƒ V* and (U ƒ V)* whenever it is convenient. Note that another way to prove the

existence of an isomorphism between these spaces is to show that ((U ƒ V)*,f) is a

tensor product for U and V, where f is the map in (C.10b). The same comment applies

with regard to the existence of an isomorphism between T

(V*) and (T

V)*. We did

not do this because for us it is convenient to be explicit about the formula (C.9b) for

the isomorphism j.

Using the isomorphism y and j in Theorems C.6.4 and C.6.5(2), respectively, gives

us an isomorphism

(C.11)

C.6.6. Theorem. The isomorphisms in (C.11) induce an isomorphism of algebras

(C.12a)

where

(C.12b)

for all a

Œ V*.

Fa a a a a a

12 12

ƒ ƒ◊◊◊ƒ

()

= ƒ ƒ◊◊◊ƒ

F :* ,TLVV

()

 :* .TL

f ab a b,.

()

()()

uv uv

f: * * *UV UV¥Æƒ

()

ja a a a a a

12 12 1122

ƒ ƒ◊◊◊ƒ

()

ƒ ƒ◊◊◊ƒ

()

()()

◊◊◊

()

kk kk

vv v v v v

j :* *TT

()

ja b a bƒ

()

()()

uv uv

C.6 The Tensor and Exterior Algebra 881

Proof. This is an easy exercise working through the deﬁnitions. Note that the left side

of equation (C.12b) has a tensor product of simply “elements” or symbols a

that happen

to belong to V* whereas the right side is a tensor product of maps as deﬁned by (C.7).

Because multilinear maps are more intuitive than abstract tensor products,

Theorem C.6.6 justiﬁes our always treating T(V*) as if it were L(V). Notice, however,

that we had to use the dual space V* to make this identiﬁcation.

Now we move on to a deﬁnition of exterior algebras. Let S

be the group of per-

mutations of {1,2, . . . ,k}. Any element s of S

induces a unique isomorphism

(C.13a)

satisfying

(C.13b)

Deﬁnition. A tensor a in T

V is said to be alternating if s(a) = sign(s)a, for all sŒ

. A linear transformation

is said to be alternating if T s=sign(s)T, for all sŒS

Now, by Theorem C.6.6 we can identify T

(V*) with (T

V)*.

C.6.7. Theorem. A tensor in T

(V*) is alternating if and only if it corresponds to an

alternating linear transformation in (T

V)* under the natural isomorphism j deﬁned

by Theorem C.6.5(2).

Proof. See [AusM63].

Deﬁnition. The alternation map Alt:T

V Æ T

V is deﬁned by

C.6.8. Theorem.

(1) The alternation map Alt:T

V Æ T

V is a linear transformation.

(2) If aŒT

V, then Alt(a) is an alternating tensor.

(3) If aŒT

V is an alternating tensor, then Alt(a) =a.

Proof. This is an easy exercise.

Theorem C.6.8 shows that Alt

= Alt, so that Alt is a projection of T

V onto the

subspace of alternating tensors.

Alt

sign if k

the identity map if k

()

≥

: VWÆ

ss s

vv v v v v vV

12 1 2

ƒ ƒ◊◊◊ƒ

()

= ƒ ƒ◊◊◊ƒ Œ

(

)

(

)

(

)

kki

s :T T

VVÆ

882 Appendix C Basic Linear Algebra

C.6.9. Theorem. Let T :T

V Æ W be a linear transformation. The map T is alter-

nating if and only if T(ker Alt) = 0.

Proof. See [AusM63].

Deﬁnition. Let V be a vector space. Deﬁne the k-fold exterior product of V, denoted

by E

V, to be the subspace of alternating tensors in T

V, that is, using Theorem

C.6.8(2),

Clearly, E

V = V.

Next, we would like to deﬁne a product for alternating tensors that maps alter-

nating tensors to alternating tensors. The tensor product of two alternating tensors is

unfortunately not always an alternating tensor, but all we have to do is project back

into the set of alternating tensors using the Alt map.

Deﬁnition. Deﬁne a map

(C.14)

The map Ÿ is called the exterior or wedge product.

The ugly factorials are added here in order to avoid them in other places, such as

in the deﬁnition of the volume element for differential forms.

C.6.10. Theorem. Let V be an n-dimensional vector space.

(1) The exterior product for V is bilinear, that is,

for w

, wŒE

(V), h

, hŒE

(V), and a Œ R.

(2) If wŒE

(V), hŒE

(V), and qŒE

(V), then

In particular, the exterior product is associative and

whqw hq w hqŸ

()

Ÿ= Ÿ Ÿ

()

ƒƒ

()

rst

Alt

!!!

ww hwhwh

whh whwh

whw h wh

12 1 2

()

Ÿ= Ÿ+ Ÿ

ŸŸ

()

=Ÿ +Ÿ

Ÿ=Ÿ = Ÿ

()

aaa

wh whŸ=

()

Alt

()

:E E E

rs rs

VV V

E Alt T T k

kkk

VVR

VVV

()

Õ≥

C.6 The Tensor and Exterior Algebra 883

for a

Œ E

(V).

(3) If wŒE

(V) and hŒE

(V), then

In particular, if a, bŒE

(V), then aŸb=- bŸaand aŸa=0.

(4) If sŒS

and a

Œ E

(V), then

(5) Let a

, a

,..., anda

form a basis for E

(V). If 1 £ k £ n, then the set of all

is a basis for E

(V). It follows that E

(V) has dimension for 0 £ k £ n.

(6) If k > n, then E

(V) = 0.

Proof. The proofs in [Spiv65] for alternating multilinear maps readily translate to

our situation here.

Deﬁnition. Let EV denote the direct sum of the vector spaces E

(V), k ≥ 0. The exte-

rior product Ÿ makes EV into an algebra called the exterior algebra or Grassmann

algebra of V.

C.6.11. Theorem. Let V be a vector space and V* its dual space. For k ≥ 1, there is

a unique isomorphism

such that

for a

Œ V* and v

Œ V = E

(V).

Proof. See [AusM63].

C.6.12. Theorem. Let V and W be vector spaces. A linear transformation T:V Æ W

induces a unique linear transformation

such that

ET E E

kk k

: VWÆ

Fa a a a

12 12

Ÿ Ÿ◊◊◊Ÿ

()

Ÿ Ÿ◊◊◊Ÿ

()

()()

kkij

vv v vdet ,

F :* *EE

()

aa a

ii i k

ii i n

1Ÿ Ÿ◊◊◊Ÿ £ < <◊◊◊< £,,

aa a saa a

ss s12 12

(

)

(

)

(

)

Ÿ Ÿ◊◊◊Ÿ =

()

Ÿ Ÿ◊◊◊Ÿ

sign .

wh hwŸ=-

()

Ÿ1

aa a aa a

12 1 2

Ÿ Ÿ◊◊◊ = ƒ ƒ◊◊◊ƒ

()

k Alt!

884 Appendix C Basic Linear Algebra

for v

Œ V.

Proof. See [AusM63].

Deﬁnition. The map E

T in Theorem C.6.12 is called the k-fold exterior product of T.

Theorem C.6.6 showed the relationship between tensor algebras and multilinear

map algebras. Now we want to show how alternating tensors are related to special

types of multilinear maps in a similar way.

Given a permutation sŒS

, deﬁne

Deﬁnition. Let V and W be vector spaces. A multilinear map

is said to be alternating if T s=(sign s)T for all sŒS

. The set of such alternating

maps will be denoted by L

(V;W). If W = R, then L

(V;W) will be abbreviated to L

(V).

It is easy to show that L

(V;W) is a vector space. By deﬁnition,

The well-known properties of the determinant function lead to the standard example

of an alternating mulilinear map.

Deﬁnition. The map

deﬁned by

is called the determinant map of R

Clearly, det ŒL

The next theorem shows that, like the tensor product, the k-fold exterior product

could have been deﬁned in terms of a universal factorization property with respect to

alternating multilinear maps. Let

det , ,..., , , ,..., ,..., , ,..., detaa a aa a aa a a

n n nl n nn ij11 12 1 21 22 2 2

()( )( )()

()

det : RR

kk kk

L and LVW VW V V;; .

()

() ()

()

: VWÆ

ss s

vv v v v v

12 1 2

, ,..., , ,..., .

()

() () ( )

s : VV

ET T T T

vv v v v v

12 1 2

Ÿ Ÿ◊◊◊Ÿ

()

Ÿ◊◊◊Ÿ

()

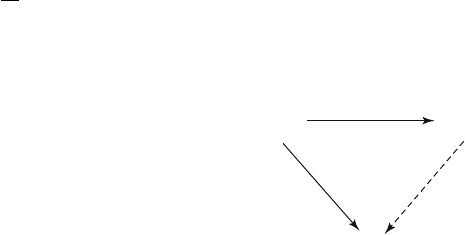
C.6 The Tensor and Exterior Algebra 885

(C.15)

be the composite of the maps

C.6.13. Theorem. Let g :V

Æ W be an alternating multilinear map. Then there is

a unique linear transformation h:E

V Æ W so that g = h  l. In fact, the map

deﬁnes an isomorphism between alternating multilinear maps on V

and linear maps

on E

V. As a special case we get an isomorphism

where

(C.16)

for all g Œ L

(V) and v

Œ V.

Proof. See Figure C.5. The theorem is an easy consequence of Theorem C.6.9. For

a proof see [AusM63].

Theorem C.6.1 and C.6.2 remain true if we replace “multilinear map” with “alter-

nating multilinear map”.

Alternating multilinear maps admit a product very much like the alternating

tensor product.

Deﬁnition. The alternation map

is deﬁned by

Alt T

sign T if k

Tifk

()

≥



Alt L L

: VV

()

vv v v v v

12 1 2

, ,...,

()

Ÿ Ÿ◊◊◊Ÿ

()

y :*,L

EVV

()

ghÆ

VVV

Alt

æÆææÆææ .

l : VV

EÆ

Figure C.5. The universal property of the space E

886 Appendix C Basic Linear Algebra

C.6.14. Theorem.

(1) The alternation map Alt:L

(V) Æ L

(V) is a linear transformation.

(2) If aŒL

(V), then Alt(a) is an alternating multilinear map.

(3) If aŒL

(V) is an alternating multilinear, then Alt(a) =a.

Proof. This is easy and proved like Theorem C.6.8.

Theorem C.6.14 implies that

and is a direct summand of L

(V). We can deﬁne a product, called the exterior or wedge

product,

(C.17)

by the same formula (C.15) that we used for the exterior algebra. Theorem C.6.10

holds verbatim for alternating multilinear maps. This is exactly how Spivak ([Spiv65])

develops the exterior algebra.

Deﬁnition. An element of L

(V) is called an exterior k-form on V. Let LV denote the

direct sum of the vector spaces L

(V), k ≥ 0. The exterior product Ÿ makes LV into

an algebra called the algebra of exterior forms on V.

Finally, we already know from Theorem C.6.11 and Theorem C.6.13 that there are

natural isomorphisms

(C.18)

so that E

(V*) and L

(V) are isomorphic.

C.6.15. Theorem. The isomorphisms in (C.18) induce an isomorphism of algebras

(C.19a)

where

(C.19b)

for all a

Œ V*.

Proof. This is another easy exercise working through the deﬁnitions. Note that the

left side of Equation (C.19b) has an exterior product of simply “elements” or symbols

that happen to belong to V* whereas the right side is an exterior product of maps

as deﬁned by (C.17).

Theorems C.6.6 and C.6.15 can be summarized compactly by saying that we have

a commutative diagram

Fa a a a a a

12 12

Ÿ Ÿ◊◊◊Ÿ

()

= Ÿ Ÿ◊◊◊Ÿ

FL:* ,E VV

()

kkk

VVV**,

()

: LL L

rs rs

VV V

Alt LVV

()

C.6 The Tensor and Exterior Algebra 887

of inclusion maps i and algebra isomorphisms F.

We ﬁnish this section with a few more facts about L(V).

C.6.16. Theorem. Let V be an n-dimensional vector space and V* its dual. If a

V*, then the element a

Ÿa

Ÿ ...Ÿa

ŒL

(V) satisﬁes

for all v

Œ V.

Proof. This is a corollary of Theorem C.6.11.

Finally, if T : V Æ W is a linear transformation, then the induced map

on dual spaces deﬁnes

C.6.17. Theorem. Under our identiﬁcations, the map E

(T*) induces the map

deﬁned by

Proof. One simply has to carefully work through all the appropriate identiﬁcations.

It is the tensor algebra TV* and the exterior algebra EV* that are the most inter-

esting because they formalize the algebra of multilinear maps and the algebra of alter-

nating multilinear maps, respectively, which are needed for deﬁning differential forms.

We ﬁnish with one ﬁnal observation. One can use the exterior algebra to deﬁne

determinants. For example, let V be an n-dimensional vector space and

a linear transformation. We know that the vector space E

V has dimension 1. There-

fore, the linear transformation

ET E E

nn n

: VVÆ

T: VVÆ

TTTT

* , ,..., , ,..., , , .aa a

()( )

()() ( )()

()

Œvv v v v v W v V

12 1 2

*: LLWV

()

ET E E

kk k

*: * *.

() ( )

()

T* : * *WVÆ

aa a a

12 12

Ÿ Ÿ◊◊◊Ÿ

()()

kkij

vv v v, ,..., det

()

æÆæ

()

»»

()

æÆæ

()

888 Appendix C Basic Linear Algebra

has the form

(C.20)

for some unique constant d. This constant is of course the determinant of T and one

could make equation (C.20) the basis of a deﬁnition of the determinant. This can also

be used to deﬁne the determinant of a matrix. Advanced books on algebra that discuss

multilinear maps often use this approach to determinants because, having developed

the machinery of tensors one then gets many of the properties of determinants for

almost “free.”

ET d

()

= ,