Agoston M.K. Computer Graphics and Geometric Modelling: Mathematics
Подождите немного. Документ загружается.

5.6.5. Theorem. (The Intermediate Value Theorem) Let X be a topological space
and f:X Æ R a continuous map. Assume that f(x
1
) < f(x
2
) for some x
1
, x
2
僆 X. If c is
a real number so that f(x
1
) < c < f(x
2
), then there is an x 僆 X with f(x) = c.
Proof. See [Eise74].
Definition. A connected subset of a topological space X that is not properly con-
tained in any connected subset of X is called a component of X.
A more intuitive way to express the notion of component is to say that a compo-
nent is a maximal connected subset.
A simpler notion of connected is:
Definition. Let X be a topological space. We say that X is path-connected if for any
two points p, q 僆 X, there is a continuous map f: [0,1] Æ X with f(0) = p and f(1) =
q. The map f is called a path from p to q. A maximal path-connected subset of a top-
ological space X is called a path-component of X.
5.6.6. Theorem. Let f:X Æ Y be a continuous map from a path-connected space
onto a space Y. Then Y is path-connected.
Proof. See [Eise74].
5.6.7. Theorem. A path-connected space is connected.
Proof. See [Eise74].
Connected does not imply path-connected in general, so that the notion of path-
connected is stronger. For “nice” spaces however these concepts are identical.
5.6.8. Theorem. A topological manifold is connected if and only if it is path-
connected.
Proof. See [Eise74].
5.7 Homotopy
We have talked about how topology studies properties of spaces invariant under
deformations (rubber sheet geometry). This section studies deformations of
mappings.
Definition. Let f, g:X Æ Y be continuous maps. A homotopy between f and g is a
continuous map
h: XY¥
[]
Æ01,
5.7 Homotopy 309

such that h(x,0) = f(x) and h(x,1) = g(x) for all x 僆 X. In that case, we shall also say
that f is homotopic to g and write f g.
If we define f
t
:X Æ Y by f
t
(x) = h(x,t), then we can see that the existence of h is
equivalent with a one-parameter family of maps connecting f and g and we can think
of h as deforming f into g. See Figure 5.12(a).
5.7.1. Example. Consider the maps f, g:D
2
Æ D
2
given by f(p) = 0 and g(p) = p.
The map h:D
2
¥ [0,1] Æ D
2
defined by h(p,t) = tp is a homotopy between them. In
other words, the identity map of D
2
is homotopic to a constant map.
5.7.2. Theorem. The homotopy relation is an equivalence relation on the con-
tinuous maps from one topological space to another.
Proof. We must show that the relation is reflexive, symmetric, and transitive.
Reflexivity: If f :X Æ Y is a continuous map, then h:X ¥ [0,1] Æ Y defined by h(x,t)
= f(x) is a homotopy between f and f.
Symmetry: Let f, g:X Æ Y be continuous maps and assume that h:X ¥ [0,1] Æ Y is
a homotopy between f and g. Define k:X ¥ [0,1] Æ Y by k(x,t) = h(x,1-t).
Then k is a homotopy between g and f.
Transitivity: Let f, g, h:X Æ Y be continuous maps and assume that a, b:X ¥ [0,1]
Æ Y are homotopies between f and g and g and h, respectively. Define
g:X ¥ [0,1] Æ Y by
Then g is a homotopy between f and h.
The theorem is proved.
ga
b
xt x t t
xt t
,,, ,
,, ,.
()
=
()
Œ
[]
=-
()
Œ
[]
2012
21 121
310 5 Point Set Topology
Y
h(X¥0)
p ¥ [0,1]
p ¥ 0
p ¥ t
p ¥ 1
X¥0
X¥1
X¥[0,1]
h(X¥t)
h(X¥1)
h
1
t
0
h
Y
y
0
y
1
y
t
= h(p,t)
(a)
(b)
Figure 5.12. Homotopies between maps.

Definition. If f:X Æ Y is a continuous map, then the homotopy class of f, denoted
by [f], is the equivalence class of f with respect to . The set of homotopy classes of
maps from X to Y will be denoted by [X,Y].
If X consists of a single point p, then a homotopy between two maps f, g: {p} Æ
Y is just a path in Y from the point y
0
= f(p) to the point y
1
= g(p). See Figure 5.12(b).
In particular, it is easy to see that the set of homotopy classes [{p},Y] is in one-to-one
correspondence with the path-components of Y.
Definition. A continuous map f:X Æ Y is called a homotopy equivalence if there is
a continuous map g:Y Æ X with g f 1
X
and f g 1
Y
. In this case we shall write
X Y and say that X and Y have the same homotopy type.
5.7.3. Theorem. Homotopy equivalence is an equivalence relation on topological
spaces.
Proof. This is straightforward.
Since the general homeomorphism problem is much too difficult except in certain
very special cases, a weaker classification is based on homotopy equivalence.
Definition. A space is said to be contractible if it has the homotopy type of a single
point.
5.7.4. Example. The unit disk D
n
is contractible. To see this we show that it has the
same homotopy type as the point 0. Define maps f:D
n
Æ 0 and g: 0 Æ D
n
by f(p) = 0
and g(0) = 0. Clearly, f g = 1
0
. Define h:D
n
¥ [0,1] Æ D
n
by h(p,t) = tp. Then h is a
homotopy between g f and the identity map on D
n
, and we are done. Another way to
state the result is to say that both f and g are homotopy equivalences.
Definition. A subspace A of a space X is called a retract of X if there exists a con-
tinuous map r:X Æ A with r(a) = a for all a in A. The map r is called a retraction of
X onto A.
If x
0
is any point in a space X, the constant map r(x) = x
0
shows that any point
of a space is a retract of the space. A less trivial example is
5.7.5. Example. The unit circle in the plane is a retract of the cylinder
(5.10)
because we have the retraction r(x,y,z) = (x,y,0).
Definition. Let A be a subspace of a space X. A deformation retraction of X onto A
is a continuous map h:X ¥ I Æ X satisfying
and
h h allxxxA xX,,,, ,01
()
=
()
ŒŒ
X =
()
+= Œ
[]
{}
x y z X Y and z,, ,
22
101
o
o
o
o
5.7 Homotopy 311
In this case A is called a deformation retract of X.
The argument in Example 5.7.4 also shows that the map f:D
n
Æ 0 defined by
f(p) = 0 is a deformation retraction of D
n
onto 0.
5.7.6. Example. The unit circle is a deformation retract of the cylinder X defined
by equation (5.10). To see this simply define h:X ¥ I Æ X by h((x,y,z),t) = (x,y,(1-t)z).
5.7.7. Theorem. Let A be a subspace of a space X. If A is a deformation retract of
X, then the inclusion map i:A Æ X is a homotopy equivalence. In particular, A and
X have the same homotopy type.
Proof. Let h:X ¥ I Æ X be a deformation retraction of X onto A. Define f: X Æ A by f(x)
= h(x,1). Since f i=1
A
and h is homotopy between i f and 1
X
, we are done.
Intuitively speaking, a subset A is a deformation retract of a space X if we can
shrink X down to A without “cutting” anything. We shall see later (Corollary 7.2.3.3
and Theorem 7.2.3.4) that a circle does not have the same homotopy type as a point.
Therefore, no point of the circle is a deformation retract of the circle. The only way
to “shrink” the circle to a point would be to cut it first.
Often it is convenient to talk about “pointed” homotopies, or more generally
“relative homotopies.”
Definition. The notation f:(X,A) Æ (Y,B) will mean that f is a map from X to Y and
f(A) 債 B.
Definition. Let f, g:(X,A) Æ (Y,B) be continuous maps. A homotopy between f and
g relative A is a continuous map
such that h(x,0) = f(x), h(x,1) = g(x), and h(a,t) 僆 B for all x 僆 X, a 僆 A, and
t 僆 [0,1]. In that case, we shall also say that f is homotopic to g relative A and write
f
A
g.
5.7.8. Theorem. The homotopy relation
A
is an equivalence relation on the set of
continuous maps f:(X,A) Æ (Y,B).
Proof. The proof is similar to the proof of Theorem 5.7.2. We just have to be careful
that the homotopies keep sending A to B.
Definition. The set of homotopy classes of maps f:(X,A) Æ (Y,B) with respect to
the equivalence relation
A
will be denoted by [(X,A),(Y,B)].
A natural question to ask at this point is how many homotopy classes of maps
there are between spaces in general and what this number measures. We are not ready
h:XY¥
[]
Æ01,
o
o
h allaa aA,, .1
()
=Œ
312 5 Point Set Topology
to answer such a question yet and will have to wait until Chapter 7, but the reader
may appreciate one glimpse into the future. Given a map
the degree of f is, intuitively, the number of times that f winds the circle around itself.
(In Section 7.5.1 we shall give another definition of the degree of f.) Define
by f
n
(cosq,sinq) = (cosnq,sinnq). Then f
n
has degree n. It turns out that all maps of
the circle to itself are homotopic to one of these maps and two maps are homotopic
only if they have the same degree, so that there is a bijection between the homotopy
classes of maps of the circle to itself and the integers.
5.8 Constructing Continuous Functions
There are many situations where one wants to define continuous functions on a
topological space satisfying certain properties. This brief section describes two very
fundamental theorems that deal with the existence of certain functions, which in turn
can be used to construct many other functions. We shall give one application having
to do with the existence of partitions of unity at the end of the section.
For a topological space to have the continuous functions we want it needs
to satisfy a special property. It is worth isolating this property and giving it a
name.
Definition. A topological space X is said to be normal if, given two disjoint
closed sets A and B in X, there exist disjoint open sets containing A and B,
respectively.
The condition that a space be normal is somewhat technical, like being Hausdorff,
but fortunately the spaces of interest to us satisfy this property.
5.8.1. Theorem.
(1) Any metrizable space is normal.
(2) Any compact Hausdorff space is normal.
Proof. See [Eise74].
5.8.2. Theorem. (The Urysohn Lemma) Let X be a normal space and assume that
A and B are two closed subsets of X. Then there exists a continuous function f:X Æ
[0,1] such that f takes the value 0 on A and 1 on B.
Proof. See [Jäni84].
f
n
:SS
11
Æ
f:SS
11
Æ ,
5.8 Constructing Continuous Functions 313
5.8.3. Theorem. (Tietze Extension Lemma) Let X be a normal space. Then any
continuous function f:A Æ [a,b] or f: A Æ R defined on a closed subset A of X can
be extended to a continuous function F:X Æ [a,b] or F :X Æ R, respectively.
Proof. See [Jäni84].
The next concept enables one to localize problems and will be used in later
chapters.
Definition. A partition of unity on a topological space X is a collection F of contin-
uous real-valued functions satisfying the following:
(1) For all j 僆 F and x 僆 X, 0 £j(x) £ 1.
(2) Every point in X has a neighborhood on which all but a finite number of func-
tions in F vanish.
(3) For every x in X
(Note that by condition (2) this is a finite sum for each x.)
If x is a cover of X, we say that the partition of unity F is subordinate to x if each
function in F vanishes outside some set in x.
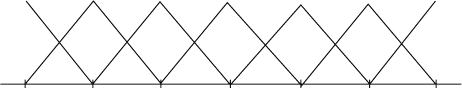
5.8.4. Example. Define functions
by
and
b
n
(x) = b(x - n).
It is easy to check that the collection of functions b
n
(x) is a partition of unity on R.
See Figure 5.13. This partition of unity is subordinate to the open cover
b x x for x
x for x
elsewhere
()
=+ Œ-
[]
=- + Œ
[]
=
110
101
0
,,,
,,,
,,
bb
n
, : RRÆ
j
j
x
()
=
Œ
Â
F
1.
314 5 Point Set Topology
b
n–1
(x) b
n+1
(x)
b
n
(x)
n–3 n–2 n–1 n+1 n+2 n+3n
Figure 5.13. A partition of unity
for R.

of R.
It would be good to know what condition on a topological space guarantees the
existence of partitions of unity.
Definition. A cover of a space is locally finite if every point in the space has a neigh-
borhood that meets only finitely many elements of the cover. A Hausdorff space is said
to be paracompact if every open cover admits a locally finite subcover.
5.8.5. Lemma. Every paracompact space is normal.
Proof. See [Jäni84].
5.8.6. Theorem. A Hausdorff space is paracompact if and only if every open cover
admits a partition of unity subordinate to it.
Proof. The only hard part is showing that paracompact implies the existence of the
stated partitions of unity. Because of Lemma 5.8.5 one can use Urysohn’s lemma to
construct the desired partition of unity. See [Jäni84].
The next obvious question is: which spaces are paracompact?
5.8.7. Theorem. The following types of topological spaces are paracompact:
(1) Compact Hausdorff spaces
(2) Topological manifolds
(3) Metrizable spaces
Proof. Part (1) is trivial. Part (2) is also not hard. For (3) see [Schu68].
5.9 The Topology of P
n
Projective space P
n
is not only one of the really important spaces in mathematics but
it also serves as an excellent example of a nontrivial topological space. This section
looks at its purely topological properties. We shall return to it later in Chapter 8 to
look at its manifold properties and again in Chapter 10 where its algebraic properties
come to the fore.
Recall the (set theoretic) definition of P
n
given in Section 3.4, namely,
(5.11)
where ~ is the equivalence relation on R
n+1
- 0 defined by p ~ cp, for c π 0. In Chapter
3 we did not say anything about its topology, but actually, when we talk about P
n
as
a topological space, we always assume that it has been given the quotient space top-
ology that is defined by equation (5.11). What does this space really “look” like top-
PR 0
nn
=-
()
+1
~,
nn n-+
()
Œ
{}
11, Z
5.9 The Topology of P
n
315
ologically? There are quite a few different definitions that all lead to the same space
(up to homeomorphism). Each gives a little different insight into its structure.
A second definition of P
n
:P
n
is the set of lines through the origin in R
n+1
.
Justification: Except for the fact that the origin is missing, the equivalence class
[x
1
,x
2
,...,x
n+1
] is just such a line through the origin, so that there is a natural one-
to-one correspondence of points. (The topologies are assumed to match under this
correspondence.)
A third definition of P
n
:P
n
is the unit sphere S
n
with antipodal points identified,
that is,
where p ~-p.
Justification: The relation ~ which relates points of S
n
to their antipodal points is
an equivalence relation, and the map
where D =|(x
1
,x
2
,...,x
n+1
)|, is clearly a homeomorphism.
A fourth definition of P
n
:P
n
is the unit disk D
n
in the plane with antipodal points
on its boundary identified, that is,
where ~ is induced from the relations p ~-p for p 僆 S
n-1
.
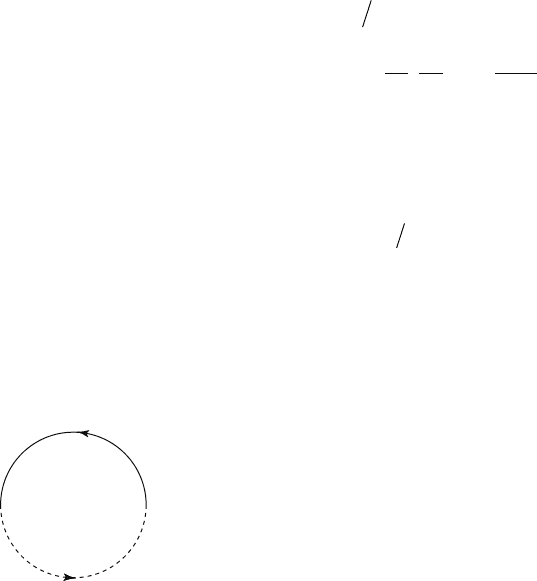
Justification: See Figure 5.14 where the labels and arrows are trying to indicate the
identifications. The boundary of the upper hemisphere S
n
+
is just S
n-1
. It is easy to see
PD
nn
= ~,
xx x
x
D
x
D
x
D
n
n
12 1
12 1
, ,..., , ,...,
+
+
[]
Æ
È
Î
Í
˘
˚
˙
R0 S
nn+
-
()
Æ
1
~/~,
PS
nn
= /~,
316 5 Point Set Topology
a
a
disk with antipodal boundary
points identified
Figure 5.14. Visualizing the projective plane.

that P
n
can be thought of as S
n
+
/~, where ~ is a restricted version of the equivalence
relation in the third definition above, namely, p ~-p for p 僆 S
n-1
. Our characteriza-
tion now follows from the observation that the only identifications that are taking
place are on the boundary of S
n
+
and that the interior of S
n
+
projects in a one-to-one
fashion onto the interior of the disk D
n
.
In the case of the projective plane, there is another well-known identification.
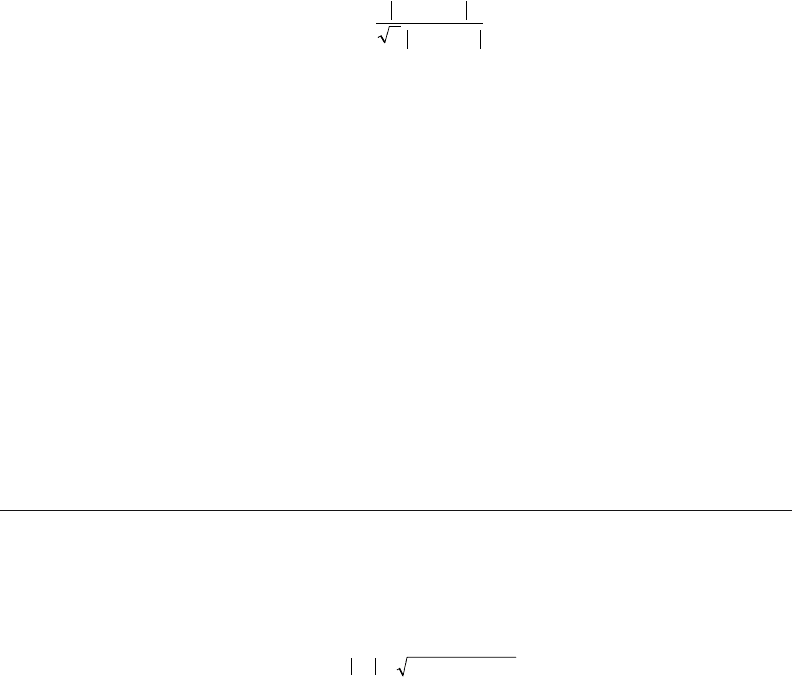
A fifth definition of P
2
: The projective plane is the union of the Moebius strip and
a disk where we identify their boundaries, which are just circles.
Justification: Consider the shaded region in Figure 5.15, which is a “collar” of the
boundary of the unit disk. A little thought should convince the reader that under
the identification described in the fourth definition, this shaded region is just the
Moebius strip.
We now have four different ways of looking at the topological space P
n
(five,
in the case of the projective plane). In each case we used a quotient topology of
Euclidean space. Alternatively, one can define this topology by defining a metric on
P
n
.
Definition. Let p = [x] and q = [y] be points of P
n
, where x, y 僆 R
n+1
. Define the
distance between p and q, denoted dist (p,q), by
It is easy to see that dist(p,q) is well defined and does not depend on the repre-
sentatives x and y that are chosen for p and q, respectively. It is just the angle between
the two “lines” p and q. The function dist(p,q) is in fact a metric on P
n
and makes P
n
into a metric space.
5.9.1. Example. Let L be the line in R
2
defined by -x + 2y + 1 = 0. To find the
points of P
2
that are “near” the ideal point L
⬁
associated to the family of lines
parallel to L.
Solution. Let p = [X,Y,Z] be any point of P
2
. Since L
⬁
= [-2,-1,0], a simple com-
putation using the definition of distance shows that the distance d between the two
points satisfies
dist d where d and dpq
xy
xy
,, .
()
==
∑
££cos 0 2p
5.9 The Topology of P
n
317
Moebius
strip
disk
a
a
Disk glued to Moebius strip
along their boundary
Figure 5.15. Another way to visualize the projective plane.
If p corresponds to a real point (x,y), that is, p = [x,y,1], the only way that d will go
to zero is if x and y both get arbitrarily large and (x,y) is near the line y - 2x = 0. This
follows from the Cauchy-Schwarz inequality. Similarly, the only ideal points close to
L
⬁
are points p = [X,Y,0] with X close to -2 and Y close to -1.
5.9.2. Theorem. N-dimensional projective space P
n
is a compact, connected,
metrizable topological manifold.
Proof. The compactness and connectedness follows from Lemma 5.3.18 and Theo-
rems 5.4.6 and 5.5.2 using the third and fourth definition of P
n
. We postpone showing
that P
n
is a manifold to Section 8.13, where we will in fact show that it is a differen-
tiable manifold.
Finally, we note that any hyperplane in P
n
is homeomorphic to P
n-1
. In particu-
lar, the subspace of ideal points is homeomorphic to P
n-1
.
5.10 EXERCISES
Section 5.2
5.2.1. Prove that every metric on a finite set is the discrete metric.
5.2.2. Prove that if a vector space V has an inner product <,>, then the function
defines a metric on V.
5.2.3. Prove that equation (5.1) defines an inner product on C
0
([0,1]).
5.2.4. Prove that the function d
1
defined by equation (5.3) defines a metric on C
0
([0,1]).
5.2.5. Prove that the function d
⬁
defined by equation (5.4) defines a metric on C
0
([0,1]).
5.2.6. Show that the metrics d
1
and d
⬁
on C
0
([0,1]) defined by equations (5.3) and (5.4), respec-
tively, are not equivalent metrics.
5.2.7. Let (X,d) be a metric space. Prove that the function d* defined by equation (5.5) is a
bounded metric on X.
5.2.8. Consider the sequence of functions f
n
(x) = x
n
on [0,1]. Show that this sequence of func-
tions converges in a pointwise fashion but not uniformly. Note also that although each
function is continuous, the limit function g(x) is not. Describe g(x).
5.2.9. Show that the rational numbers are not complete by giving an example of a Cauchy
sequence of rational numbers that does not converge to a rational number.
5.2.10. Sometimes one does not quite have a metric on a space.
d uv uv vuvu,,
()
==<-->
cos d
XY
XYZ
=
--
()
2
5,,
.
318 5 Point Set Topology
