Agoston M.K. Computer Graphics and Geometric Modelling: Mathematics

Подождите немного. Документ загружается.

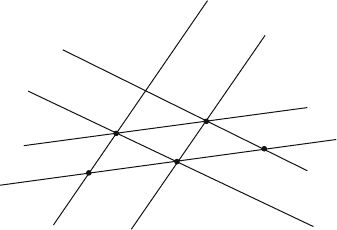
form A + rAB + sAC for all rational numbers r and s. These points are a dense set of

points in the plane. The ﬁnal step handles the points where r or s are irrational. See

[Gans69].

2.4.7. Corollary. An afﬁne transformation of the plane is completely determined by

what it does to three noncollinear points.

Proof. Showing that the corollary follows from Theorem 2.4.6 uses an, by now stan-

dard, argument that is left as an exercise for the reader.

We are ready to state and prove a fundamental theorem about afﬁne maps.

2.4.8. Theorem. Every afﬁne transformation of the plane can be described uniquely

by equations of the form (2.25). The determinant in (2.25b) is called the determinant

of the afﬁne transformation. Conversely, every such pair of equations deﬁnes an afﬁne

transformation.

Proof. We start with the converse. A transformation T deﬁned by equations (2.25)

has an inverse that is again deﬁned by linear equations of the same form. Let f(x,y)

= 0 be the equation of a line L. Then the set L¢=T(L) is deﬁned by the equation

f(T

-1

(x,y)) = 0. This shows that L¢ is a line and that T is an afﬁne map.

Next, let T be an afﬁne map and choose three noncollinear points. By Theorem

2.4.5 there is a map M deﬁned by equations (2.25) that agrees with T on those points.

Since we just showed that T is an afﬁne map, we have two afﬁne maps that act the

same on three noncollinear points. By Corollary 2.4.7, T = M and the theorem is

proved.

Because of Theorem 2.4.8 everything proved for the maps deﬁned by equations

(2.25) holds for afﬁne maps. We restate these properties to emphasize their validity

for afﬁne maps.

(1) Every afﬁne map in the plane is a composition of translations, rotations,

shears, and/or scaling transformations. Conversely, every composition of such

maps is an afﬁne map.

(2) There is a unique afﬁne transformation in the plane that maps three non-

collinear points into any other three noncollinear points.

2.4 Afﬁne Transformations 99

E = A + 2AB

Figure 2.19. Proving Theorem 2.4.6.

2.4.9. Theorem. The only afﬁne transformations of the plane that preserve angles

are similarities.

Sketch of proof. Let T be an afﬁne transformation that preserves angles. Choose

noncollinear points A, B, and C. If T(A,B,C) = (A¢,B¢,C¢), then one can show that

for some r > 0. Let U be the radial transformation U(p) = (1/r)p and let (A≤,B≤,C≤) =

(UT)(A,B,C). There is a unique motion M such that (A≤,B≤,C≤) = M(A,B,C). Now S =

-1

M is a similarity that agrees with T on A, B, and C. By Corollary 2.4.7, T and S

must be the same transformations.

Deﬁnition. The ratio of division of three distinct points A, B, and P on an oriented

line L in R

, denoted by (AB,P), is deﬁned by

(||AP|| and ||PB|| are the signed distances on the oriented line L.)

2.4.10. Proposition. Let A, B, and P be distinct points on an oriented line L. If

P = A + tAB = (1 - t)A + tB, then

In particular, (AB,P) is independent of the orientation of L.

Proof. See Figure 2.20. The proof is a straightforward consequence of the fact that

AP = tAB and PB = (1 - t)AB.

Using Proposition 2.4.10 it is easy to show that the ratio of division (AB,P) is

positive if P belongs to the segment [A,B] and negative otherwise.

2.4.11. Proposition. Let T be an afﬁne transformation of the plane. If A,B Œ R

then

AB,P

()

AB,P

()

= .

¢¢= ¢¢= ¢¢=A B AB B C BC A C ACr r and r,,

100 2 Afﬁne Geometry

tAB

(1–t)AB

||AP||

||PB||

Figure 2.20. The ratio of division.

for all t.

Proof. By Theorem 2.4.8, there is a nonsingular 2 ¥ 2 matrix M and a point P,

so that T(Q) = QM + P for all Q. Now all one has to do is use this formula for T to

evaluate both sides of the equation and show that they are equal.

2.4.12. Theorem. Afﬁne transformations of the plane preserve the ratio of division.

Proof. The theorem is an easy consequence of Propositions 2.4.10 and 2.4.11.

2.4.13. Theorem. Afﬁne transformations in the plane multiply area by the absolute

value of their determinant.

Proof. See [Gans69].

Theorem 2.4.13 points out one of the main intuitions one should have about deter-

minants, namely, that they are intrinsically connected with how transformations

expand or shrink area, volume, etc. A precise deﬁnition of volume will be given in

Chapter 4.

Deﬁnition. The equiafﬁne or equiareal group is the group of afﬁne transformations

with determinant ±1.

Recall our earlier comments how geometric properties are intimately connected

to certain groups of transformations. Here are three groups, the “metric” groups, and

their associated “metric” properties:

Deﬁnition. Afﬁne properties are properties preserved only by afﬁne transformations

(and not by projective transformations, which we will deﬁne shortly).

Some afﬁne properties are betweenness, the ratio of division, parallelism, and the

concurrence of lines.

Deﬁnition. Two ﬁgures F and F¢ are afﬁnely equivalent if there is an afﬁne transfor-

mation T with T(F) = F¢.

Any two segments, angles, triangles, parallelograms, lines, parabolas, ellipses, and

hyperbolas are afﬁnely equivalent. This means that one can use special simple ﬁgures

to prove things about general ﬁgures!

2.4.14. Example. To prove that the midpoints of all parallel chords of a parabola

X are collinear and lie on a line parallel to the axis. See Figure 2.21(a).

motions similarities equiafﬁne

distance angle size area

cc c

Ttt tT tT11-

()

()()

()

AB A B

2.4 Afﬁne Transformations 101

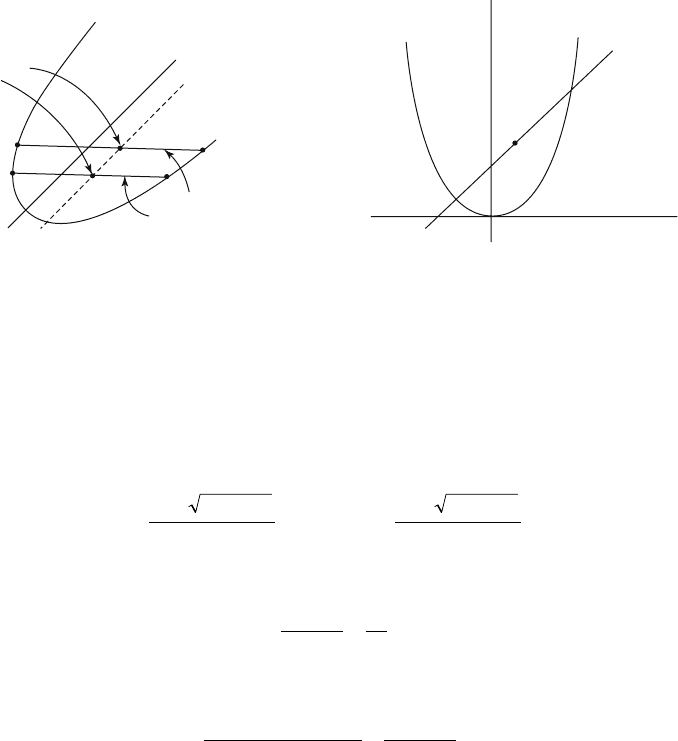
102 2 Afﬁne Geometry

Solution. Since all parabolas are afﬁnely equivalent we may restrict ourselves to the

special case of the parabola deﬁned by the equation y = x

and the family of chords

determined by the lines y = mx + b, where m is ﬁxed and b ≥ 0. See Figure 2.21(b).

To ﬁnd the intersection of the lines with the parabola, we must solve the equation

mx + b = x

. The two solutions are

The midpoint Q = (u,v) of such a chord is deﬁned by

and

which proves the result.

Finally, note that one could have developed afﬁne geometry without ﬁrst coordi-

natizing points. We could make points, lines, etc., undeﬁned terms and use axioms to

deﬁne their properties. This is the synthetic geometry approach. Coordinates could be

introduced at a later stage. The point is that, in the context of afﬁne geometry, the

exact lengths of geometric ﬁgures are not important. At most it is relative size that

counts, that is, the ratios of segments.

2.4.1 Parallel Projections

Deﬁnition. Let v be a nonzero vector in R

and let W be the family of parallel lines

with direction vector v. Let L

denote the line in W through the point p. If X is a

hyperplane in R

not parallel to v, then deﬁne a map

mx b mx b m b

++ +

xx m

mm b

and x

mm b

midpoints

axis

parallel

chords

(a)

(b)

y = x

y = mx + b

Figure 2.21. Midpoints of parallel chords for parabola are parallel to axis.

The map p

is called the parallel projection of R

onto the plane X parallel to v. If v is

orthogonal to X, then p

is called the orthogonal or orthographic projection of R

onto

the plane X; otherwise, it is called an oblique parallel projection. In general, if X and

Y are any subsets of R

, then the map that sends p in X to L

« Y in Y (wherever it

is deﬁned) is called the parallel projection of X to Y.

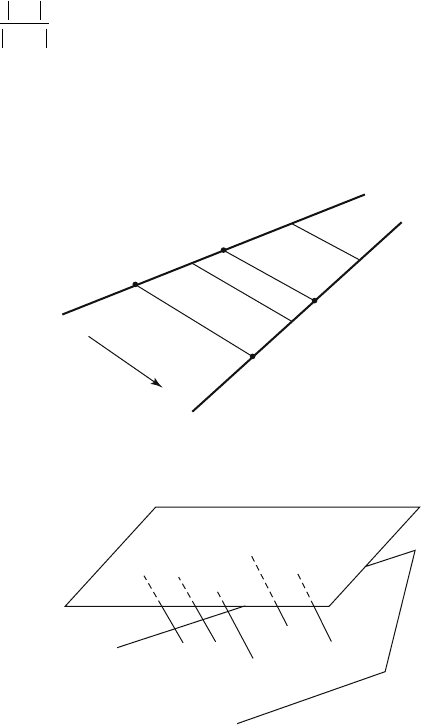
Figure 2.22 shows a parallel projection of a line L onto a line L¢ and Figure 2.23,

a parallel projection of a plane X onto a plane X¢. Note that the ratio of distances is

preserved in the case of parallel projections of a line onto another line. What this

means is that, referring to Figure 2.22, the ratio

is independent of A and B. This is not the case for parallel projections of one plane

onto another. For example, in Figure 2.23 the ratios

AB¢¢

pL X

()

=«.

: RX

2.4 Afﬁne Transformations 103

A¢

B¢

L¢

X¢

B¢

C¢

A¢

Figure 2.22. A parallel projection between

lines.

Figure 2.23. A parallel projection between

planes.

are probably not the same.

2.4.1.1. Example. To ﬁnd the parallel projection T of R

onto the plane X deﬁned

by the equation

parallel to v = (3,1,1).

Solution. Clearly, given a point p, if t is chosen so that p + tv belongs to X, then

T(p) = p + tv. Let p = (x,y,z). Solving

for t, gives t = (1/2) (-x + 2y - z). It follows that T is deﬁned by the equations

2.4.1.2. Theorem. A parallel projection between two hyperplanes in R

preserves

parallelism, concurrence, betweenness, and the ratio of division.

Proof. Easy.

2.4.1.3. Theorem. Any map of the plane onto itself that is a composition of

parallel projections is an afﬁne map. Conversely, every afﬁne map in the plane is a

composite of parallel projections.

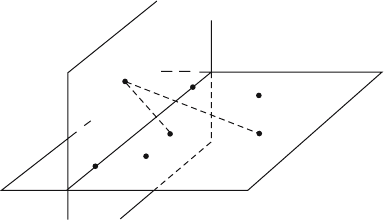
Sketch of proof. The ﬁrst statement follows from the fact that lines are preserved.

Now let T be an afﬁne map. Assume that A, B, and C are noncollinear points with

T(A,B,C) = (A¢,B¢,C¢). First, project R

to a plane X that contains A and B so that C

gets sent to a point C

. Next, project X back to R

in such a way as to send C

to C¢.

It follows that the composite of these two projections sends A to A, B to B, and C to

C¢. Repeat this process on A¢ and B¢. See Figure 2.24.

The construction in the proof of Theorem 2.4.1.3 shows that any afﬁne map can

be realized as a composite of at most six projections.

¢=-+++zxyz

¢=-+-+yxyz

¢=-+-+xxyz

xt ytzt+

()

=32 3

xyz-+=23

BC¢¢ ¢¢

and

104 2 Afﬁne Geometry

2.5 Beyond the Plane

Up to now, although some things applied to R

, most of the details were speciﬁcally

about transformations in the plane. The fact is that much of what we did generalizes

to higher dimensions.

We start with motions of R

2.5.1. Theorem. Every motion M:R

Æ R

can be expressed by equations of the

form

(2.29)

where A

= (a

) is an orthogonal matrix. Conversely, every such system of equations

deﬁnes a motion.

Proof. The discussion in Section 2.2.8 on frames showed that the theorem is valid

for motions in the plane. For the general case, assume without loss of generality that

M(0) = 0. The key facts are Theorem 2.2.4.1, which says that M is a linear trans-

formation (and hence is deﬁned by a matrix), and Lemma 2.2.4.3, which says that

M(u)•M(v) = u • v, for all vectors u and v. The rest of the proof simply involves ana-

lyzing the conditions M(e

)•M(e

) = e

•e

and is left as an exercise (Exercise 2.5.1).

In studying motions in the plane we made use of some important special motions,

such as translations, rotations, and reﬂections. Translations already have a general

deﬁnition. The natural generalization of the deﬁnition of a reﬂection is to replace lines

by hyperplanes.

Deﬁnition. Let X be a hyperplane in R

. Deﬁne a map S:R

Æ R

, called the reﬂec-

tion about the hyperplane X, as follows: Let A be a point in X and let N be a normal

◊◊ ◊ ◊ ◊

¢= + + + +xaxax axc

nn n nnn

11 2 2

...

¢= + + + +

◊◊ ◊ ◊ ◊

xaxax axc

1 11 1 12 2 1 1

2 21 1 22 2 2 2

...

2.5 Beyond the Plane 105

B¢

C¢

A¢

Figure 2.24. Afﬁne maps as composites

of parallel projections.

106 2 Afﬁne Geometry

vector for X. If P is any point in R

, then S(P) = P + 2PQ, where PQ is the orthogo-

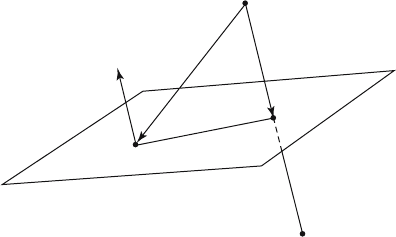
nal projection of PA on N. See Figure 2.25.

2.5.2. Theorem. Let S be a reﬂection about a hyperplane X.

(1) The deﬁnition of S depends only on the hyperplane and not on the point A

and normal vector N that are chosen for it in the deﬁnition.

(2) If t is chosen so that P + tN is the point where the line through P with direc-

tion vector N meets the plane X, then S(P) = P + 2tN.

(3) The ﬁxed points of S are just the points of X.

(4) If L¢ is a line orthogonal to X, then S(L¢) = L¢.

(5) Reﬂections about hyperplanes are motions.

Proof. The details of the proof are left as an exercise for the reader because it is

essentially the same as the proof of Theorem 2.2.3.1. That proof did not really use the

fact that vectors were two-dimensional.

2.5.3. Example. To ﬁnd the reﬂection S about the plane X deﬁned by the equation

Solution. Let A be any point in X. Since N = (1,-2,-2) is a normal vector for

X, if P is any point, then it is easy to show that the orthogonal projection of

PA on N is just tN, where t is chosen so that P + tN lies in X. Let P = (x,y,z).

Solving

for t, gives

t = (1/9)(-x + 2y + 2z + 3).

Since S(P) = P + 2tN, it follows that S has equations

xt y t z t+

()

=22223

xyz--=223.

P¢

Figure 2.25. Deﬁning a reﬂection in

higher dimensions.

Generalizing the concept of a rotation is a little less obvious. The simplest way to

get a deﬁnition is in a roundabout way by deﬁning a rigid motion ﬁrst and then use

the orientation-preserving nature of these maps.

Deﬁnition. Let M be a motion of R

and suppose the equations for M are as shown

in Theorem 2.5.1. The motion M is said to be a rigid motion if the matrix (a

) is a

special orthogonal matrix.

In analogy to the planar case we get

2.5.4. Theorem. A rigid motion of R

is an orientation-preserving map. Conversely,

every orientation-preserving motion of R

is a rigid motion.

Proof. Exercise.

Deﬁnition. A rigid motion R of R

that ﬁxes some point p is called a rotation. In

that case, we say that R is a rotation about p. The point p is called a center of the

rotation.

Is this deﬁnition of a rotation really what we want and does it generalize the intu-

itively simple notion of a rotation in the plane? Theorem 2.2.6.9 certainly shows that

the new deﬁnition is compatible with the old one.

2.5.5. Theorem. (The Principal Axis Theorem) Every rotation R in R

is a “rotation

about some line.” More precisely, with respect to some appropriate coordinate system,

R is just the rotation about the z-axis through an angle q, that is, the equations for R

in that coordinate system are just

(2.30)

In general, if R is a rotation in R

, then we can choose a coordinate system

with respect to which the n ¥ n matrix of coefﬁcients in the equation for R has the

form

¢=zz.

¢= +y x in y osscqq

¢= -xx ycos sinqq

¢= - + -zxyz

¢= + - -yxyz

¢= + + +xxyz

2.5 Beyond the Plane 107

108 2 Afﬁne Geometry

Conversely, every transformation of R

whose equation has such a matrix of coefﬁ-

cients is a rotation.

Proof. See [Lips68]. Note that rotations about the origin are linear transformations

so that one can talk about their associated matrices.

Theorem 2.5.5 suggests that the expression “rotation about a point” is perhaps

misleading in higher dimensions. Although it might be better to say “rotation about

a line,” we shall keep it in order to have a uniform terminology since it makes per-

fectly good sense in the plane. Actually, we shall see shortly in the next section that

one should really talk about directed lines here because the expression “rotation about

a line through an angle q” is ambiguous.

The main theorems about motions in R

can now be stated. Their proofs are very

similar to the proofs of the corresponding theorems about motions in the plane and

are omitted.

2.5.6. Theorem.

(1) A motion in R

is completely determined by what it does to n + 1 linearly inde-

pendent points.

(2) A rigid motion in R

is completely determined by what it does to n linearly

independent points.

(3) Every motion in R

can be described as a composition of a translation, a rota-

tion about the origin, and/or a reﬂection.

(4) Every rigid motion in R

is a composition of a translation and/or a rotation

about the origin.

Proof. Exercise.

Facts about similarities and afﬁne maps in the plane also generalize to R

2.5.7. Theorem. Every similarity transformation can be expressed by equations of

the form (2.29) where (a

) = (db

), d > 0, and (b

) is an orthogonal matrix. Conversely,

every such system of equations deﬁnes a similarity.

Proof. Exercise.

cos s

s cos

cos sin

sin cos

(2.31)